A box weighing 2000 N is to be slowly slid through 20 m on a straigh track having friction coefficient 0.2 with the box. A find the work done by the person pulling the box wilth a chain at angle with the horizontal. B. find the work when the person has chosen a value of which ensures him the minimum magnitude of the force.
The correct Answer is:A, B, D
To solve the problem step by step, we will break it down into two parts as specified in the question.
Part A: Finding the Work Done by the Person Pulling the Box
1. Identify the Given Values:
- Weight of the box (W) = 2000 N
- Distance (d) = 20 m
- Coefficient of friction (μ) = 0.2
2. Draw the Free Body Diagram:
- The box is being pulled at an angle θ with a force F.
- The vertical component of the force is Fsinθ.
- The horizontal component of the force is Fcosθ.
3. Calculate the Normal Force (N):
- The normal force can be expressed as:
N=W−Fsinθ
- Since the box is moving slowly, the frictional force (f) can be calculated as:
f=μN=μ(W−Fsinθ)
4. Set Up the Equation for Horizontal Motion:
- For the box to move, the horizontal component of the pulling force must equal the frictional force:
Fcosθ=μ(W−Fsinθ)
5. Rearranging the Equation:
- Rearranging gives:
Fcosθ+μFsinθ=μW
- Factoring out F:
F(cosθ+μsinθ)=μW
- Thus, the force F can be expressed as:
F=μWcosθ+μsinθ
6. Calculate the Work Done (W):
- The work done by the person pulling the box is given by:
Work=F⋅d⋅cosθ
- Substituting for F:
Work=(μWcosθ+μsinθ)⋅d⋅cosθ
- Plugging in the values:
Work=(0.2⋅2000cosθ+0.2sinθ)⋅20⋅cosθ
- Simplifying gives:
Work=8000cosθcosθ+0.2sinθ
Part B: Finding the Work Done for Minimum Force
1. Condition for Minimum Force:
- To minimize the force, we set the derivative of cosθ+μsinθ to zero:
tanθ=μ
- Thus, tanθ=0.2.
2. Calculate Cosine and Sine Values:
- Using tanθ=0.2:
sinθ=0.2√1+0.22=0.2√1.04≈0.196
cosθ=1√1+0.22=1√1.04≈0.98
3. Substituting Values into Work Equation:
- Substitute sinθ and cosθ back into the work equation:
Work=8000⋅0.980.98+0.2⋅0.196
- Calculate the denominator:
0.98+0.0392≈1.0192
- Thus, the work done becomes:
Work≈8000⋅0.981.0192≈7680 J
Final Answers:
- Part A: Work done when pulling at angle θ is 8000cosθcosθ+0.2sinθ.
- Part B: Work done for minimum force is approximately 7680 J.
- Weight of the box (W) = 2000 N
- Distance (d) = 20 m
- Coefficient of friction (μ) = 0.2
- The box is being pulled at an angle θ with a force F.
- The vertical component of the force is Fsinθ.
- The horizontal component of the force is Fcosθ.
- The normal force can be expressed as:
N=W−Fsinθ
- Since the box is moving slowly, the frictional force (f) can be calculated as:
f=μN=μ(W−Fsinθ)
- For the box to move, the horizontal component of the pulling force must equal the frictional force:
Fcosθ=μ(W−Fsinθ)
- Rearranging gives:
Fcosθ+μFsinθ=μW
- Factoring out F:
F(cosθ+μsinθ)=μW
- Thus, the force F can be expressed as:
F=μWcosθ+μsinθ
- The work done by the person pulling the box is given by:
Work=F⋅d⋅cosθ
- Substituting for F:
Work=(μWcosθ+μsinθ)⋅d⋅cosθ
- Plugging in the values:
Work=(0.2⋅2000cosθ+0.2sinθ)⋅20⋅cosθ
- Simplifying gives:
Work=8000cosθcosθ+0.2sinθ
- To minimize the force, we set the derivative of cosθ+μsinθ to zero:
tanθ=μ
- Thus, tanθ=0.2.
- Using tanθ=0.2:
sinθ=0.2√1+0.22=0.2√1.04≈0.196
cosθ=1√1+0.22=1√1.04≈0.98
- Substitute sinθ and cosθ back into the work equation:
Work=8000⋅0.980.98+0.2⋅0.196
- Calculate the denominator:
0.98+0.0392≈1.0192
- Thus, the work done becomes:
Work≈8000⋅0.981.0192≈7680 J
- Part A: Work done when pulling at angle θ is 8000cosθcosθ+0.2sinθ.
- Part B: Work done for minimum force is approximately 7680 J.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer16videos
WORK AND ENERGY
- BOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -28videos
WORK AND ENERGY
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer7videos
WAVE MOTION AND WAVES ON A STRING
Similar Questions
A block of mass m lying on a horizontal surface (coefficient of static friction =μs) is to be brought into motion by a pulling force F At what angle θ with the horizontal should the force F be applied so that its magnitude is minimum ? Also find the minimum magnitude
 View Solution
View SolutionA block of weight 100 N is slowly slid up on a smooth incline of inclination 370 by as person. Calculate the work done by the person in moving the block through a distance of 2.0 m, if the driving force is a. parallel to the incline and b. in the horizontal direction.
View SolutionA block of weight 100 N is slowly slide up on a smooth incline of inclination 370 by as person. Calculate the work done by the person in moving the block through a distance of 2.0 m, if the driving force is a. parallel to the incline and b. in the horizontal direction.
View SolutionA man pulls a box on a horizontal floor through a distance of 20 m with a force of 30 N applied along a rope tied to the box and making and angle of 60° with the horizontal. Calculate the work done.
View SolutionIn a children\'s park, there is a slide which has a total length of 10 m and a height of 8.0 m figure. Vertical ladder are provided to reach the top. A boy weighting 200 N climbs up the ladder to the top of the slide and slides down to the ground. The averages friction offered by the slide is three tenth of his weight. Find a. the work done by the ladder on the boy as he goes up. b. the work done by the slide on the boy as he comes down. Neglect any work done by forces inside the body of the boy.
View SolutionIn a children\'s park, there is a slide which has a total length of 10 m and a height of 8.0 m figure. Vertical ladder are provided to reach the top. A boy weighting 200 N climbs up the ladder to the top of the slide and slides down to the ground. The averages friction offered by the slide is three tenth of his weight. Find a. the work done by the ladder on the boy as he goes up. b. the work done by the slide on the boy as he comes down. Neglect any work done by forces inside the body of the boy.
View SolutionA uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is µ. The work done by the friction during the period the chain slips off the table is [−2kμMgL]. Find the value of k.
View SolutionA box of mass 200 kg is kept on a rough horizontal floor having coefficient of static friction μs=0.25 and coefficient kinetic friction μk=0.2. Find the work done by a man to slide the body slowly through 20 m with a minimum force. Also find the magnitude of the minimum force.
View SolutionA box of bananas weighing 40.0Nrests on a horizontal surface is 0.40 and the coefficient of kinetic friction is 0.20
a. If no horizontal force is applied to the box and the box is at rest , how large is the friction force excered on the box ?
b. What is the magnitude of the friction forces if a monkey applies a horizontal force of 6.0N to the box and the box is initial at rest?
c. What minimum horizontal force must the monkey apply to start the box in motion ?
d. What minimum horizontal force must the monkey apply to keep the box moving at constant velocity once it has been starts?
e. If the monkey applies a horizontal force of 18.0N What is the magnitude of the friction force and what is the box's acceleration ?View SolutionA uniform chain of mass m and length l is at the verge of sliding under the effect of gravity of the hanging part. Find the
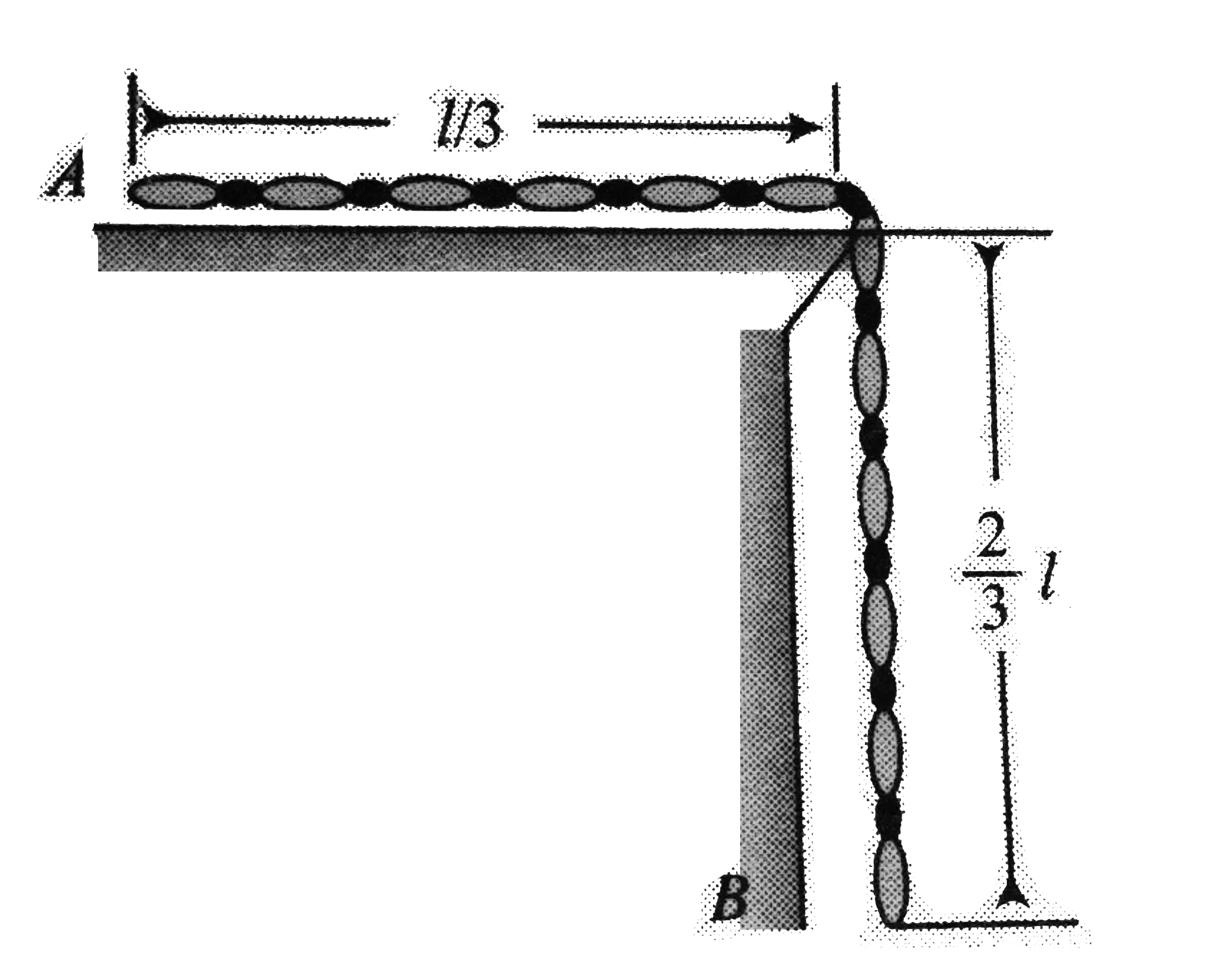
a. coefficient of friction, between chain and table.
b. work done by friction and gravity till the chain leaves the table if the hanging part is pulled gently and released.
c. speed of the chain at the time of leaving the table in part (b) .
d. work done by the external force acting at the end A of the chain in slowly pulling the chain completely onto the table.View Solution

