A boy of mass m1 , standing on a smooth horizontal surface, throws a sphere of mass m2 parallel to the surface. After a time t, if the separation between them becomes x, then show that the work done by the boy in throwing the sphere
=12(xt)2(m1m2m1+m2)Solution in Bengali
Let v denote the velocity of the sphere, and V denote the velocity of the boy, due to reaction. From the law of conservation of momentum ,
m1V=m2v ……..(1)
Separation between them after a time t.
x=(V+v)t ……..(2)
From (1) and (2) we get,
m2m1v+v=xtor,v=xtm1m1+m2
Similarly, V=xt⋅m2m1+m2
Work done in throwing the sphere
= sum of kinetic energies of the sphere and the boy
=12m1V2+12m2v2
=12m1(xt)2⋅m22(m1+m2)2+12m2(xt)2m21(m1+m2)2
=12(xt)2m1m2(m1+m2)2(m1+m2)
=12(xt)2⋅m1m2m1+m2
Topper's Solved these Questions
- WORK AND ENERGYBOOK - CHHAYA PUBLICATIONCHAPTER - WORK AND ENERGYEXERCISE - SECTION RELATED QUESTIONS
24 Videos
- WORK AND ENERGYBOOK - CHHAYA PUBLICATIONCHAPTER - WORK AND ENERGYEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
38 Videos
- WBCHSE QUESTION PAPER -2019BOOK - CHHAYA PUBLICATIONCHAPTER - WBCHSE QUESTION PAPER -2019EXERCISE - SECTION - II ( GROUP -D)
10 Videos
Similar Questions
Define work.
View SolutionA car is moving with a uniform velocity along a horizontal road. Does the engne of car do any work in this instance ?
View SolutionEquations of trajectory of a projectile are x=4 t, y=3t−5t2 , where x and y are in metre and t is in second . Find (i) maximum height attained by the projectille , (ii) horizontal range of the projectile .
View SolutionIf y+3=m1(x+2) and y+3=m2(x+2) are two tangents to the parabola y2=8x, then (a)m1+m2=0 (b) m1+m2=−1 (c)m1+m2=1 (d) none of these
View SolutionIf the equations ax2+2hxy+by2=0andy2−(m1+m2)xy+m1m2x2=0 represent the same curve, find m1+m2andm1m2.
View SolutionConsider two solid spheres of radii R_1 = 1 m, R_2 =2m and masses M_1 and M_2 , respectively . The gravitational field due to sphere (1) and (2) are shown. The value of M_1/M_2 is :
View SolutionTwo particles of masses m1andm2 move on the same plane. If their relative velocity is u, and the velocity of their centre of mass is v, show that the total kinetic energy is
12(m1+m2)v2+12(m1m2m1+m2)u2.
View SolutionThe acceleration of two bodies of mass m1 and m2 in contact on a horizontal surface is
View SolutionTwo blocks of masses m1 and m2(m1>m2) in contact with each other on frictionless, horizontal surface. If a horizontal force F is given onm1 set into motion with acceleration a, then reaction force on mass m1 by m2 is
View SolutionA dynamometer D is attached to weights of masses m_1 and m_2 Two forces F_1 and F_2 are applied to the masses F_1 and F_2 as shown. The entire arrangement is there on a smooth horizontal surface. The reading of the dynamometer, if m_1 = m_2 = m is
View SolutionIn the arrangement shown in the figure all surfaces are frictionless, the masses of the block are m1=20kgandm2=30kg. The accelerations of masses m1andm2 will be if F = 180N.
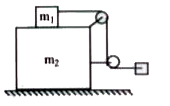
View SolutionIf y+3=m1(x+2) and y+3=m2(x+2) are two tangents to the parabola y2=8x, then (a)m1+m2=0 (b) m1+m2=−1 (c)m1+m2=1 (d) none of these
View SolutionTwo bodies of masses m1 and m2 are separated by certain distance. If →F12 is the force on m1 due to m2 and →F21 is the force on m2 due to m1 , then
View SolutionGravitational force between masses m1 and m2 is F. If a third mass m3 is placed near m1 and m2, then the force between m1 and m2 would be
View SolutionTwo bodies of masses m1andm2 are separated by certain distance. If →F12 is the force on m1 due to m2and→F21 is the force on m2 due to m1 then
View Solution
