A bucket tied to a string is lowered at a constant acceleration of g/4. If mass of the bucket is m and it is lowered by a distance l then find the work done by the string on the bucket.
−34mgl
34mgl
43mgl
−43mgl
The correct Answer is:C, D
To find the work done by the string on the bucket, we can follow these steps:
Step 1: Identify the forces acting on the bucket
The forces acting on the bucket are:
- The weight of the bucket acting downwards, which is mg.
- The tension in the string acting upwards, which we will denote as T.
Step 2: Write the equation of motion
Since the bucket is being lowered with a constant acceleration of g4, we can apply Newton's second law:
mg−T=ma
Here, a=g4. Substituting this into the equation gives:
mg−T=m(g4)
Step 3: Solve for the tension T
Rearranging the equation to isolate T:
T=mg−m(g4)
Factoring out mg:
T=mg(1−14)=mg(34)
Thus, the tension T is:
T=34mg
Step 4: Calculate the work done by the string
The work done by the string on the bucket is given by:
W=T⋅s⋅cos(θ)
Where:
- T is the tension we found,
- s is the distance the bucket is lowered, which is l,
- θ is the angle between the tension and the displacement. Since tension acts upwards and the displacement is downwards, θ=180∘ (or π radians), and cos(180∘)=−1.
Substituting the values:
W=(34mg)⋅l⋅(−1)
This simplifies to:
W=−34mgl
Final Answer
The work done by the string on the bucket is:
W=−34mgl
---
The forces acting on the bucket are:
- The weight of the bucket acting downwards, which is mg.
- The tension in the string acting upwards, which we will denote as T.
Since the bucket is being lowered with a constant acceleration of g4, we can apply Newton's second law:
mg−T=ma
Here, a=g4. Substituting this into the equation gives:
mg−T=m(g4)
Rearranging the equation to isolate T:
T=mg−m(g4)
Factoring out mg:
T=mg(1−14)=mg(34)
Thus, the tension T is:
T=34mg
The work done by the string on the bucket is given by:
W=T⋅s⋅cos(θ)
Where:
- T is the tension we found,
- s is the distance the bucket is lowered, which is l,
- θ is the angle between the tension and the displacement. Since tension acts upwards and the displacement is downwards, θ=180∘ (or π radians), and cos(180∘)=−1.
W=(34mg)⋅l⋅(−1)
This simplifies to:
W=−34mgl
The work done by the string on the bucket is:
W=−34mgl
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.2
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.3
2 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Miscellaneous Example
6 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionA block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionA block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionA man pulls out a block of mass m from the depth d with help of a light string. If acceleration of the block is g2 then the work done by the string on the block will be
View SolutionA block of mass 5kg is being raised vertically upwards by the help of a string attached to it. It rises with an acceleration of 2ms−2. Find the work done by the tension in the string if the block rises by 2.5m. Also find the work done by the gravity and the net work done.
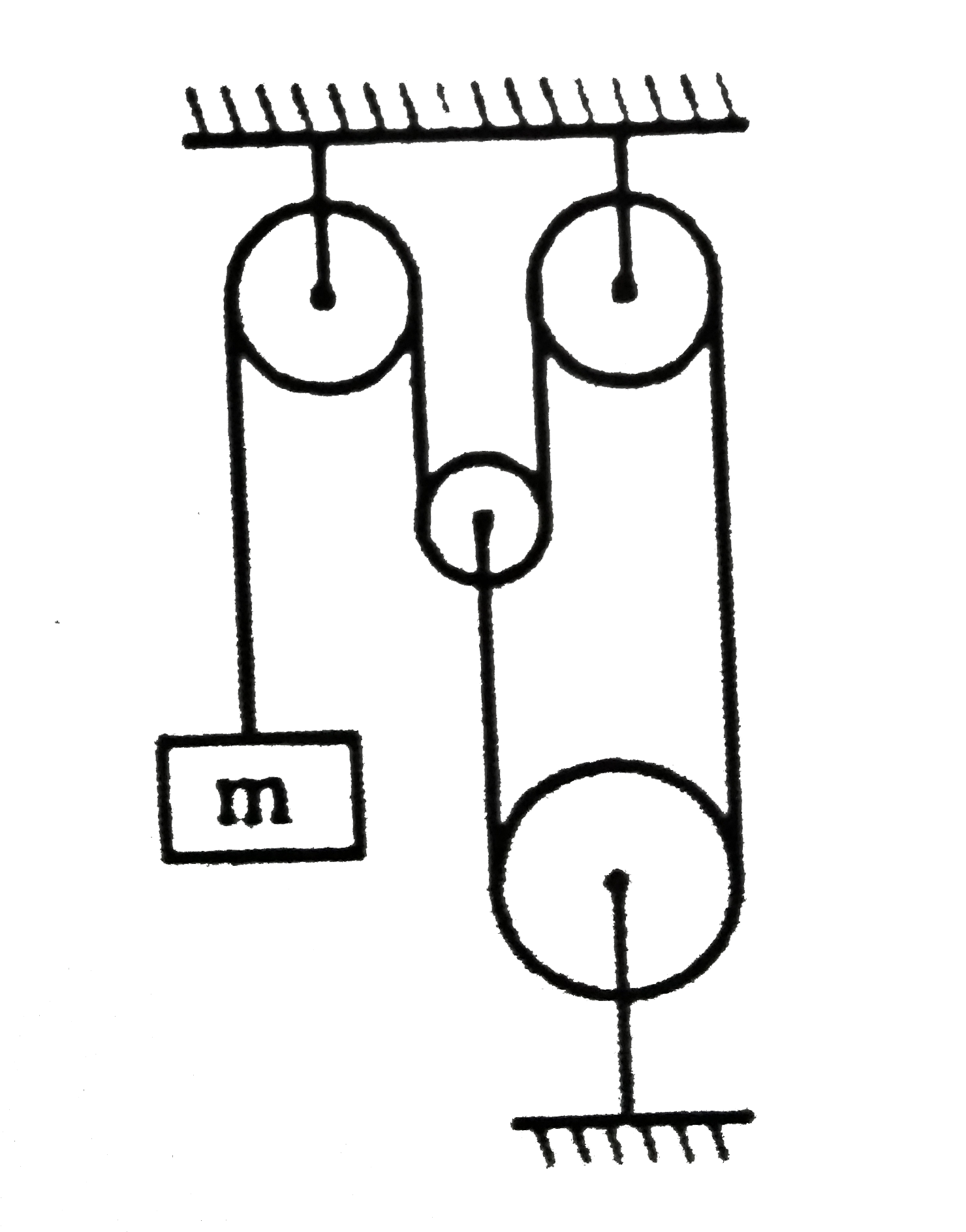
View SolutionIf the string & all the pulleys are ideal, acceleration of mass m is :-
View SolutionA mass M is lowered with the help of a string by a distance h at a distance acceleration g/2. The work done by the string will be
View SolutionA bucket full of water weighs 5 kg, it is pulled from a well 20 m deep. There is a small hole in the bucket through which water leaks at a constant rate. If it is observed that for every meter the bucket loses 0.2 kg mass of water, then the total work done in pulling the bucket up from the well is [g=10ms−2]
View SolutionA block of mass 2 kg is being brought down by a string. If the block acquires a speed 1(m)/(s) in dropping down 25 cm, find the work done by the string in the process.
View SolutionA string is used to pull a block of mass m vertically up by a distance h at a constant acceleration g3. The work done by the tension in the string is
View Solution
