A bulb is connected to a battery of p.d. 4 V and internal resistance 2.5 Ω. A steady current of 0.5 A flows through the circuit. Calculate :
the resistance of the bulb,
To solve the problem, we need to calculate the resistance of the bulb connected to a battery. Here are the steps to find the solution:
Step 1: Identify the given values
- Potential difference (V) = 4 V
- Internal resistance of the battery (r) = 2.5 Ω
- Current (I) = 0.5 A
Step 2: Use Ohm's Law to calculate the total resistance in the circuit
Ohm's Law states that:
V=I×R
Where:
- V is the voltage,
- I is the current,
- R is the total resistance.
We can rearrange this formula to find the total resistance (R):
R=VI
Step 3: Substitute the known values into the equation
Substituting the given values into the equation:
R=4V0.5A
R=8Ω
Step 4: Relate the total resistance to the internal resistance and the resistance of the bulb
The total resistance in the circuit is the sum of the internal resistance of the battery and the resistance of the bulb (R'):
R=R′+r
Where:
- R' is the resistance of the bulb,
- r is the internal resistance of the battery.
Step 5: Substitute the known values into the equation
We already found that the total resistance R is 8 Ω, and the internal resistance r is 2.5 Ω:
8Ω=R′+2.5Ω
Step 6: Solve for the resistance of the bulb (R')
Rearranging the equation to find R':
R′=8Ω−2.5Ω
R′=5.5Ω
Conclusion
The resistance of the bulb is:
R′=5.5Ω
---
- Potential difference (V) = 4 V
- Internal resistance of the battery (r) = 2.5 Ω
- Current (I) = 0.5 A
Ohm's Law states that:
V=I×R
Where:
- V is the voltage,
- I is the current,
- R is the total resistance.
R=VI
Substituting the given values into the equation:
R=4V0.5A
R=8Ω
The total resistance in the circuit is the sum of the internal resistance of the battery and the resistance of the bulb (R'):
R=R′+r
Where:
- R' is the resistance of the bulb,
- r is the internal resistance of the battery.
We already found that the total resistance R is 8 Ω, and the internal resistance r is 2.5 Ω:
8Ω=R′+2.5Ω
Rearranging the equation to find R':
R′=8Ω−2.5Ω
R′=5.5Ω
The resistance of the bulb is:
R′=5.5Ω
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(C) MULTIPLE CHOICE TYPE)
2 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
Similar Questions
A bulb is connected to a battery of p.d. 4 V and internal resistance 2.5 Ω. A steady current of 0.5 A flows through the circuit. Calculate :
the energy dissipated in the bulb in 10 minutes.
View SolutionA bulb is connected to a battery of p.d. 4 V and internal resistance 2.5 Ω. A steady current of 0.5 A flows through the circuit. Calculate :
the total energy supplied by the battery in 10 minutes,
View SolutionThe circuit diagram in Fig. shows three resistors 2Ω,4Ωand RΩ connected to a battery of e.m.f. 2 V and internal resistance 3 Ω. If current of 0.25 A flows through the circuit, find :
the p.d. across the internal resistance of the cell,
View SolutionThe circuit diagram in Fig. shows three resistors 2Ω,4Ωand RΩ connected to a battery of e.m.f. 2 V and internal resistance 3 Ω. If current of 0.25 A flows through the circuit, find :
the value of R.
View SolutionThe circuit diagram in Fig. shows three resistors 2Ω,4Ωand RΩ connected to a battery of e.m.f. 2 V and internal resistance 3 Ω. If current of 0.25 A flows through the circuit, find :
the p.d. across the 4 Ω resistor,
View SolutionThe circuit diagram in Fig. shows three resistors 2Ω,4Ωand RΩ connected to a battery of e.m.f. 2 V and internal resistance 3 Ω. If current of 0.25 A flows through the circuit, find :
the p.d. across the R Ωor 2 Ω resistor,
View SolutionThe wire of potentiometer has resistance 4Ω and length 1m. It is connected to a cell of e.m.f. 2V and internal resistance 1Ω. The current flowing through the potentiometer wire is
View SolutionA coil of self inductance 2.5 H and resistance 20Ω is connected to a battery of emf 120 V having internal resistance of 5Ω. Find :
The current in the circuit in steady state.
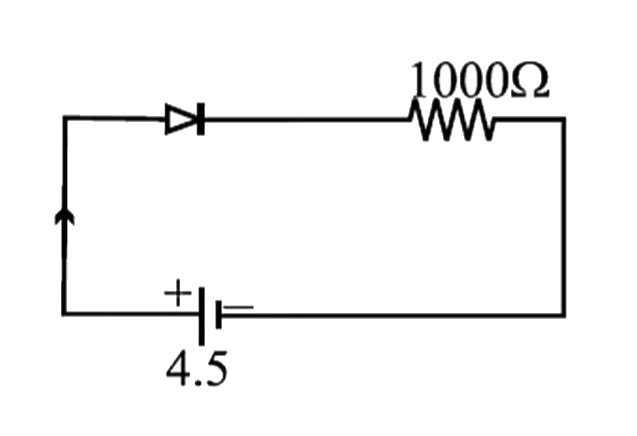
View SolutionA P-N junction diode connected to a battery of e.m.f. 4.5 V and an external resistance of 1000Ω. What is the value of current in the circuit, if potential barrier in the diode =0.5V
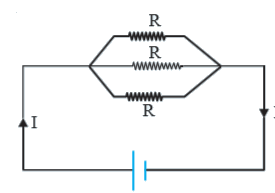
View SolutionThree equal resistances, each of RΩ are connected as shown in figure. A battery of emf 2 V and internal resistance 0.1Ω is connected across the circuit. Calculate the value of R for which the heat generated in the circuit is maximum?
View Solution
