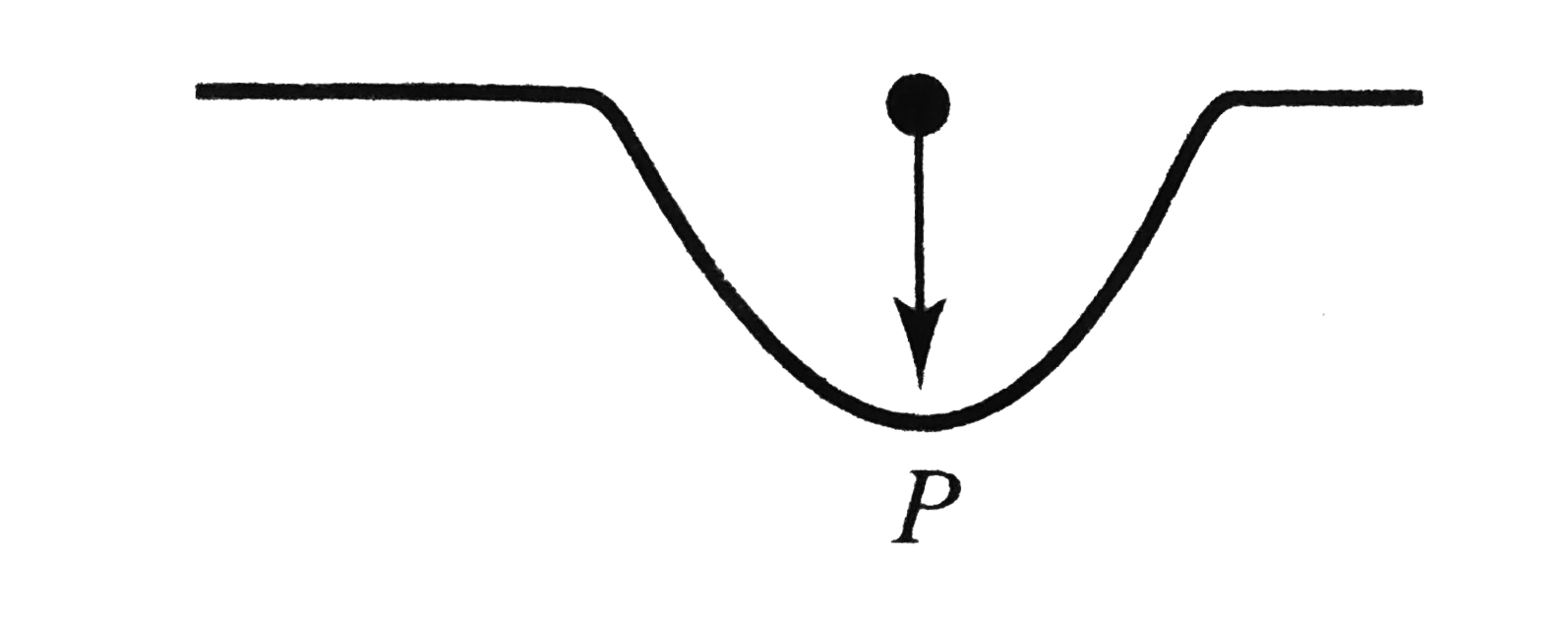
A car of mass m moving over a concave bridge of radius r .Find the normal reaction acting on car when it is at lowest point the bridge
To find the normal reaction acting on a car of mass m when it is at the lowest point of a concave bridge of radius r, we can follow these steps:
Step 1: Identify the forces acting on the car
At the lowest point of the concave bridge, two main forces are acting on the car:
- The gravitational force (mg) acting downwards.
- The normal reaction force (N) acting upwards from the surface of the bridge.
Step 2: Apply Newton's second law
At the lowest point, the car is undergoing circular motion. Therefore, the net force acting on the car must provide the necessary centripetal force required for circular motion. According to Newton's second law, we can express this as:
N−mg=mv2r
where:
- N is the normal reaction force,
- mg is the weight of the car,
- mv2r is the centripetal force required to keep the car moving in a circular path of radius r.
Step 3: Rearrange the equation to solve for N
Rearranging the equation gives us:
N=mg+mv2r
Step 4: Interpret the result
This equation shows that the normal reaction force N at the lowest point of the bridge is the sum of the gravitational force acting on the car and the centripetal force required for circular motion.
Final Answer
Thus, the normal reaction acting on the car when it is at the lowest point of the bridge is:
N=mg+mv2r
---
At the lowest point of the concave bridge, two main forces are acting on the car:
- The gravitational force (mg) acting downwards.
- The normal reaction force (N) acting upwards from the surface of the bridge.
At the lowest point, the car is undergoing circular motion. Therefore, the net force acting on the car must provide the necessary centripetal force required for circular motion. According to Newton's second law, we can express this as:
N−mg=mv2r
where:
- N is the normal reaction force,
- mg is the weight of the car,
- mv2r is the centripetal force required to keep the car moving in a circular path of radius r.
Rearranging the equation gives us:
N=mg+mv2r
This equation shows that the normal reaction force N at the lowest point of the bridge is the sum of the gravitational force acting on the car and the centripetal force required for circular motion.
Thus, the normal reaction acting on the car when it is at the lowest point of the bridge is:
N=mg+mv2r
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A car of mass m moving over a convex bridge of radius r .Find the normal reaction acting on car when it is at bighest point the bridge.
View SolutionA simple pendulum of length l is suspended from the ceilling of a car moving with a speed v on a circular horizontal road of radius r. a. Find the tension in the string when it is at rest with respect to the car. b.Find the time period of small oscillation.
View SolutionA car of mass 800 kg is moving with a constant velocity 40 km/h. Find the net force on it.
View SolutionA car of mass 800 kg is moving with a constant velocity 40 km/h . Find the net force on it.
View SolutionA car of mass m is moving on a level circular track of radius R if μs represents the static friction between the road and tyres of the car, the maximum speed of the car in circular motion is given by.
View SolutionA point mass m is moved in vertical circle of radius r with help of string. Velocity of mass is 7gr√ at lowest point. Tension in string at lowest point is
View SolutionA body of mass m is moving in a circle of radius angular velocity ω. Find the expression for centripetal force acting on it by the method of dimensions
View SolutionA car is moving with uniform speed over a circular bridge of radius R which subtends an angle of 90∘ at its center. Find the minimum posible speed so that the car can cross the bridge without losing the contact any where
View SolutionA spherical ball of mass m and radius r rolls without slipping on a rough concave surface of large radius R. It makes small oscillations about the lowest point. Find the time period.
View SolutionA car travelling on a smooth road passes through a curved portion of the road in the form of an arc of circle of radius 10m. If the mass of car is 120kg, find the reaction (in kN) on car at lowest point P where its speed is 20ms−1.
View Solution
