A car starts moving rectilinearly first with acceleration α=5ms−2 (the initial velocity is equal to zero), then uniformly, and finally, deceleration at the same rate α comea to a stop. The time of motion equals t=25s. The average velocity during this time is equal to <br>=72 km h^(-1) How long does the car move unitromly?
To solve the problem, we will follow these steps:
Step 1: Convert Average Velocity
The average velocity is given as 72 km/h. We need to convert this into meters per second (m/s) for consistency in units.
Average Velocity=72km/h=72×10003600=20m/s
Step 2: Define Variables
Let:
- t = time of acceleration (in seconds)
- 25−2t = time of uniform motion (in seconds)
- α=5m/s2 (acceleration and deceleration)
Step 3: Calculate Final Velocity After Acceleration
Using the first equation of motion, the final velocity v after accelerating for time t is:
v=u+at=0+5t=5tm/s
Step 4: Calculate Total Displacement
The total displacement S can be calculated as the sum of the displacements during the acceleration phase, the uniform motion phase, and the deceleration phase.
1. Displacement during acceleration:
S1=12at2=12⋅5⋅t2=5t22
2. Displacement during uniform motion:
S2=v⋅time=(5t)⋅(25−2t)=5t(25−2t)=125t−10t2
3. Displacement during deceleration:
S3=12at2=12⋅5⋅t2=5t22
Step 5: Total Displacement
The total displacement S is given by:
S=S1+S2+S3=5t22+(125t−10t2)+5t22
S=125t−10t2+5t2=125t−5t2
Step 6: Use Average Velocity Formula
The average velocity is defined as:
Average Velocity=Total DisplacementTotal Time
Substituting the known values:
20=125t−5t225
Multiplying both sides by 25:
500=125t−5t2
Rearranging gives:
5t2−125t+500=0
Dividing the entire equation by 5:
t2−25t+100=0
Step 7: Solve the Quadratic Equation
Using the quadratic formula t=−b±√b2−4ac2a:
t=25±√(−25)2−4⋅1⋅1002⋅1
t=25±√625−4002
t=25±√2252
t=25±152
Calculating the two possible values:
t=402=20andt=102=5
Step 8: Determine Time of Uniform Motion
We need to find the time of uniform motion:
Time of Uniform Motion=25−2t
For t=20:
Time of Uniform Motion=25−2(20)=25−40=−15(not possible)
For t=5:
Time of Uniform Motion=25−2(5)=25−10=15s
Final Answer
The car moves uniformly for 15 seconds.
---
The average velocity is given as 72 km/h. We need to convert this into meters per second (m/s) for consistency in units.
Let:
- t = time of acceleration (in seconds)
- 25−2t = time of uniform motion (in seconds)
- α=5m/s2 (acceleration and deceleration)
Using the first equation of motion, the final velocity v after accelerating for time t is:
The total displacement S can be calculated as the sum of the displacements during the acceleration phase, the uniform motion phase, and the deceleration phase.
S1=12at2=12⋅5⋅t2=5t22
S2=v⋅time=(5t)⋅(25−2t)=5t(25−2t)=125t−10t2
S3=12at2=12⋅5⋅t2=5t22
The total displacement S is given by:
S=S1+S2+S3=5t22+(125t−10t2)+5t22
S=125t−10t2+5t2=125t−5t2
The average velocity is defined as:
Average Velocity=Total DisplacementTotal Time
Substituting the known values:
20=125t−5t225
Multiplying both sides by 25:
500=125t−5t2
Rearranging gives:
5t2−125t+500=0
Dividing the entire equation by 5:
t2−25t+100=0
Using the quadratic formula t=−b±√b2−4ac2a:
t=25±√(−25)2−4⋅1⋅1002⋅1
t=25±√625−4002
t=25±√2252
t=25±152
Calculating the two possible values:
t=402=20andt=102=5
We need to find the time of uniform motion:
Time of Uniform Motion=25−2t
For t=20:
Time of Uniform Motion=25−2(20)=25−40=−15(not possible)
For t=5:
Time of Uniform Motion=25−2(5)=25−10=15s
The car moves uniformly for 15 seconds.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Solved Examples9videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.117videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer9videos
KINEMATICS-2
Similar Questions
A car starts moving along a line, first with acceleration a=5 ms−2 starting from rest then uniformly and finally decelerating at the same rate a, comes to rest.The total time of motion is τ=25s. The average velocity during the time is equal to lt v gt =72 km/hr.How long does the partial move uniformly ?
View SolutionA car start moving along a line, fisrt with acceleration a=5ms−2 starting from rest then uniformly and finally decelerating at the same rate a, comes to rest. The total time of motion is π=25s. The average velocity during the time is equal to <v>=72kmhr. How long does the particle move uniformly ?
View Solution
Knowledge Check
- Question 1 - Select One
A car starts from rest and accelerates at uniform rate of 6ms−2 for some time, then moves with constant speed for some time and retards at the same uniform rate and comes to rest. Total time for the journey is 24 s and average speed for journey is 20ms−1 , How long does the car move with constant speed?
A4 sB8 sC12 sD16 s - Question 2 - Select One
The velocity- time graph of the particle moving along a straight line is shown. The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the average velocity during the motion is 20ms−1, then the value of t is
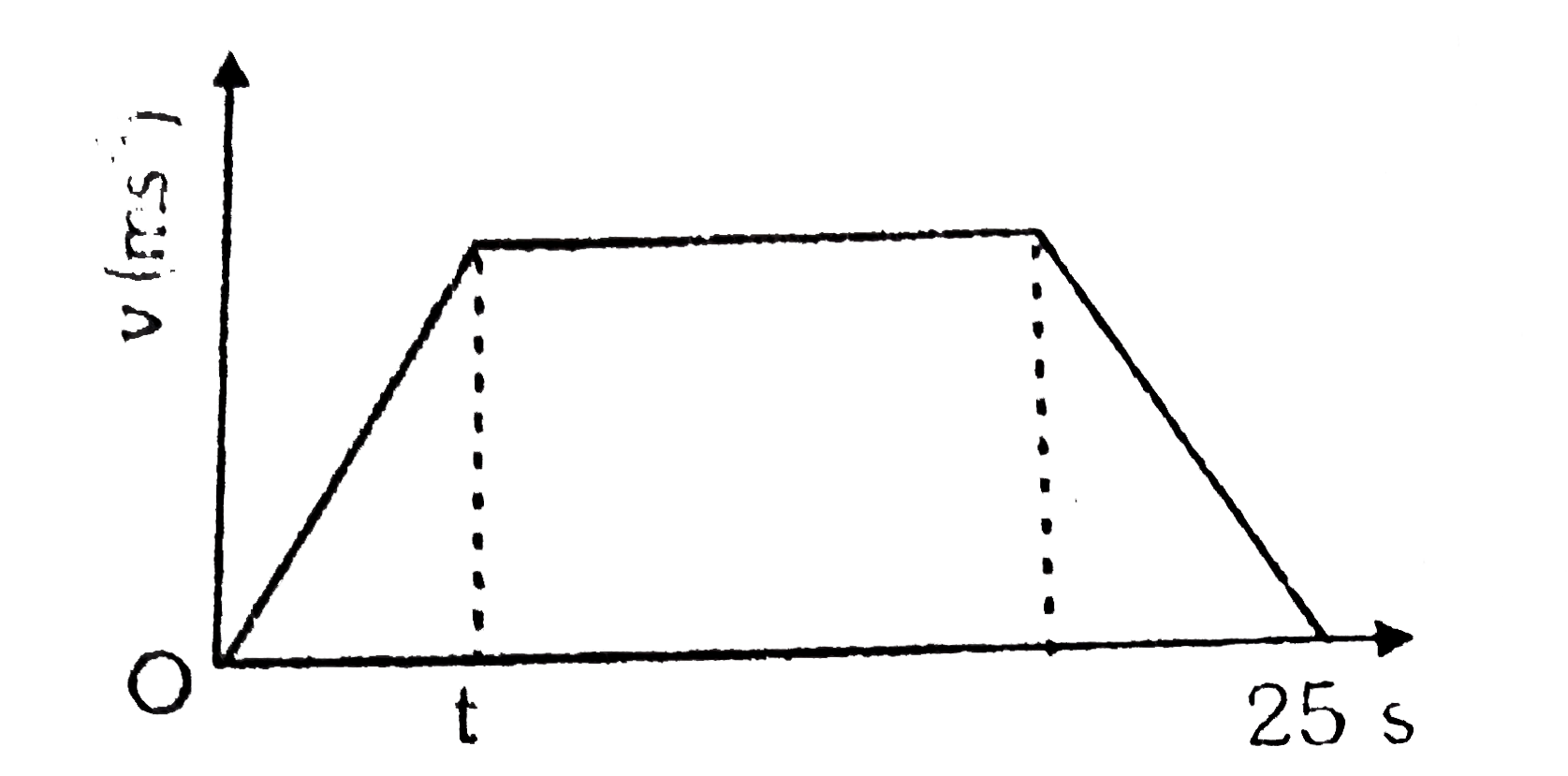 A3 secB5 secC10 secD12 sec
A3 secB5 secC10 secD12 sec - Question 3 - Select One
A car starts from rest, attain a velocity of 36kmh−1 with an acceleration of 0.2ms−2, travels 9 km with this uniform velocity and then comes to halt with a uniform deaceleration of 0.1 ms−2. The total time of travel of the car is
A1050 sB1000 sC950 sD900 s
A car starts moving along a line, first with acceleration a=5m/s2 starting from rest then uniformly and finally decelerating at the same rate till it comes to rest. The total of motion is 25s. The average speed during the time is 20m/s. The particle moves uniformly for (2.5x) second. Find the value of x.
View SolutionA train starting from rest moves with a uniform acceleration of 0.2m/s2 for 5 minutes . Calculate the speed acquired and the distance travelled in this time.
View SolutionA cockroach moves rectilinearly such that after sometime t0 let its (instantaneous) velocity be equal to its average velocity over that time. Referring to the SΔt graph as shown in , for the motion of the cockroach, find the time t0 and the average velocity of the cockroach over the time t0
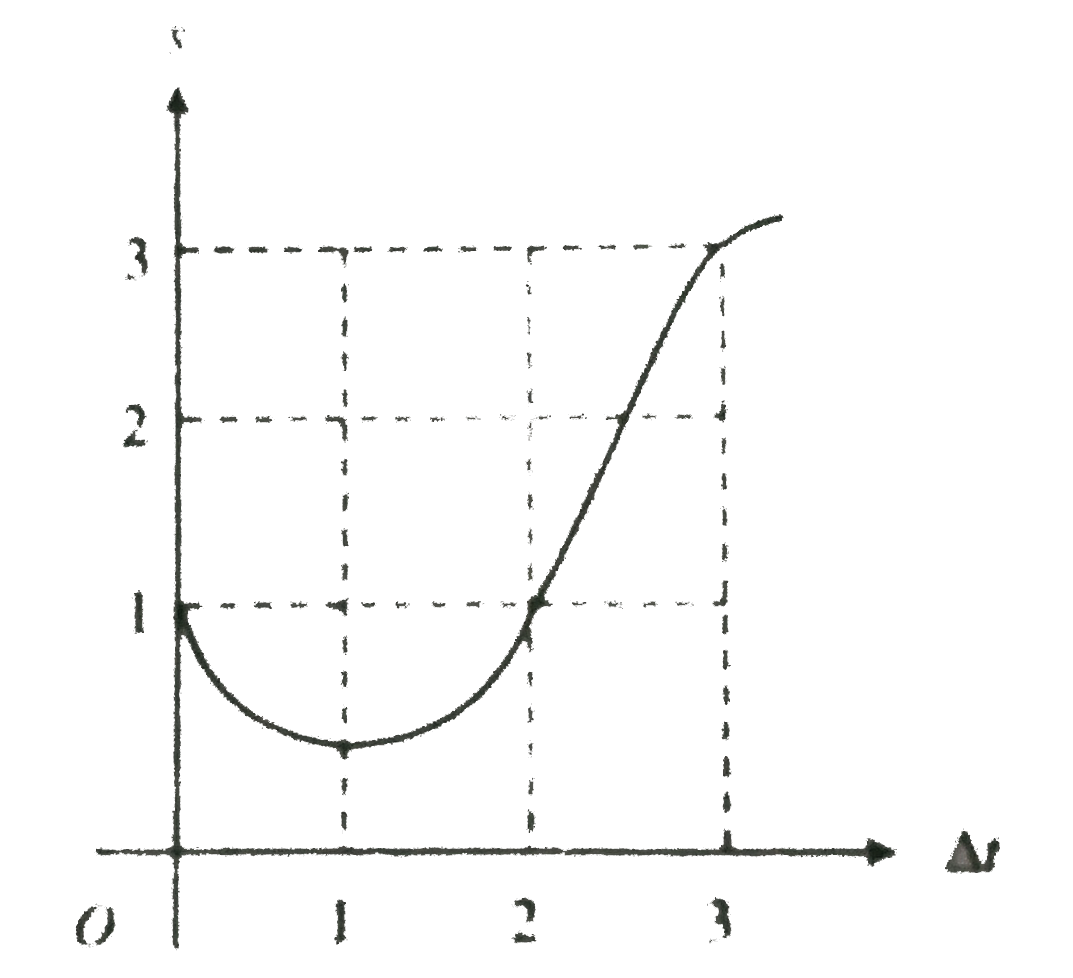 . View Solution
. View SolutionThe velocity-time graph of a particle moving along a straight line is shown is . The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The value of t is.View SolutionA train starts from rest and moves with uniform acceleration α for some time and acquires a velocity v it then moves with constant velocity for some time and then decelerates at rate β and finally comes to rest at the next station. If L is distance between two stations then total time of travel is
View Solution

