A chain of 100 links is 1 m long and has a mass of 2 kg . With the ends fastened together it is set roation at 3000 rpm.then centripetal force on each link is
3.14 N
31.4 N
314 N
3140 N
The correct Answer is:C
To solve the problem of finding the centripetal force on each link of a chain rotating at 3000 rpm, we can follow these steps:
Step 1: Determine the mass of each link
The total mass of the chain is given as 2 kg, and there are 100 links in total. Therefore, the mass of each link can be calculated as:
Mass of each link=Total massNumber of links=2kg100=0.02kg
Step 2: Convert the rotational speed from rpm to radians per second
The angular velocity ω in radians per second can be calculated using the formula:
ω=2πn60
where n is the rotational speed in rpm. Substituting n=3000:
ω=2π×300060=100πrad/s
Step 3: Determine the radius of the circular path
The length of the chain when straight is 1 meter, which becomes the circumference of the circular path when the chain is formed into a circle. The relationship between circumference C and radius r is given by:
C=2πr
Setting C=1m:
1=2πr⟹r=12πm
Step 4: Calculate the centripetal force on each link
The centripetal force Fc acting on each link can be calculated using the formula:
Fc=mω2r
Substituting the values we have:
- m=0.02kg
- ω=100πrad/s
- r=12πm
Now substituting these into the centripetal force formula:
Fc=0.02×(100π)2×12π
Calculating (100π)2:
(100π)2=10000π2
Now substituting this back into the equation for Fc:
Fc=0.02×10000π2×12π
Simplifying:
Fc=0.02×10000×π22π=0.02×10000×π2=100πN
Step 5: Calculate the numerical value of the centripetal force
Using π≈3.14:
Fc≈100×3.14=314N
Final Answer
The centripetal force on each link is approximately 314 N.
---
The total mass of the chain is given as 2 kg, and there are 100 links in total. Therefore, the mass of each link can be calculated as:
The angular velocity ω in radians per second can be calculated using the formula:
The length of the chain when straight is 1 meter, which becomes the circumference of the circular path when the chain is formed into a circle. The relationship between circumference C and radius r is given by:
The centripetal force Fc acting on each link can be calculated using the formula:
- ω=100πrad/s
- r=12πm
Using π≈3.14:
The centripetal force on each link is approximately 314 N.
Topper's Solved these Questions
Similar Questions
A body weighing 13 kg is suspended by two strings 5 m and 12 m long, their other ends being fastened to the extremities of a rod 13 m long. If the rod be so held that the body hangs immediately below the middle point. The tensions in the strings are (1) 12 kg and 13 kg (2) 5 kg and 5 kg (3) 5 kg and 12 kg (4) 5 kg and 13 kg
View SolutionIf Fig. 5-48, a chain consisting of five links, each of mass 0.100 kg, is lifted vertically with constant acceleration of magnitude a=2.50 m/s2. Find the magnitudes of (a) the force on link 1 from link 2, (b) the force on link 2 from link 3, (c) the force on link 3 from link 4, and (d) the force on link 4 from link 5. Then find the magnitudes of (e) the force →F on the top link from the person lifting the chain and (f) the net force accelerating each link.
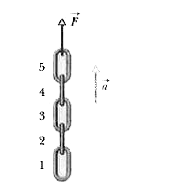
View Solution
Knowledge Check
A chain of 125 links is 1.25 m long and has a mass of 2 kg with the ends fastened together it is set rotating at 3000 revmin. Find the centripetal force on each link -
A3.14 NB314 NC13.14ND1314NA chain of 125 links is 1.25 m long and has mass of 2 kg with the ends fastened together. It is set for rotating at 50 revolutions per second. The centripetal force on each link is
A3.14 NB0.314 NC314 NDNone of theseA store of mass 1 kg , attached at the end of a 1 m long string is whirled in a horizontal circle . IF the string makes an angle of 30∘ with the vertical , then the approximate value of the centripetal force acting on the stone is (g=10m/s2)
A4NB5NC6ND7N
A particle of mass 2 kg is moving along a circular path of radius 1 m. If its angular speed is 2π rad s−1, the centripetal force on it is
View SolutionA chain of 5 links each of mass 0.1 kg is lifted vertically with a constant acceleration 1.2 ms2. The force of interaction between the top link and the one immediately below it is
View SolutionA body of mass 5 kg is moving in a circle of radius m 1 with an angular velocity of 2 radian/sec . The centripetal force is
View SolutionA ring of mass 100 kg and diameter 2 m is rotating at the rate of (300π) rpm. Then
View SolutionIf a body of 5 kg is moving in a circle of radius 5 m with a velocity 100 m s−1, then the centripetal force acting on the body is
View Solution
