A chain of length L and mass M is held on a frictionless table with part hanging over the edge. Work done in pulling the chain is directly proportional to
Let = mass per unit length of the chain and y is the length of the chain hanging over the edge. So the mass of the chain of length y will be and the force acting on it due to gravity will be .
The work done in pulling the length of the chain on the table.
[dy is negative as y is decreasing]
As the chain is pulled slowly,
F=Weight of the hanging chain
i.e.,
So the work done in pulling the hanging portion on the table,
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples
15 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
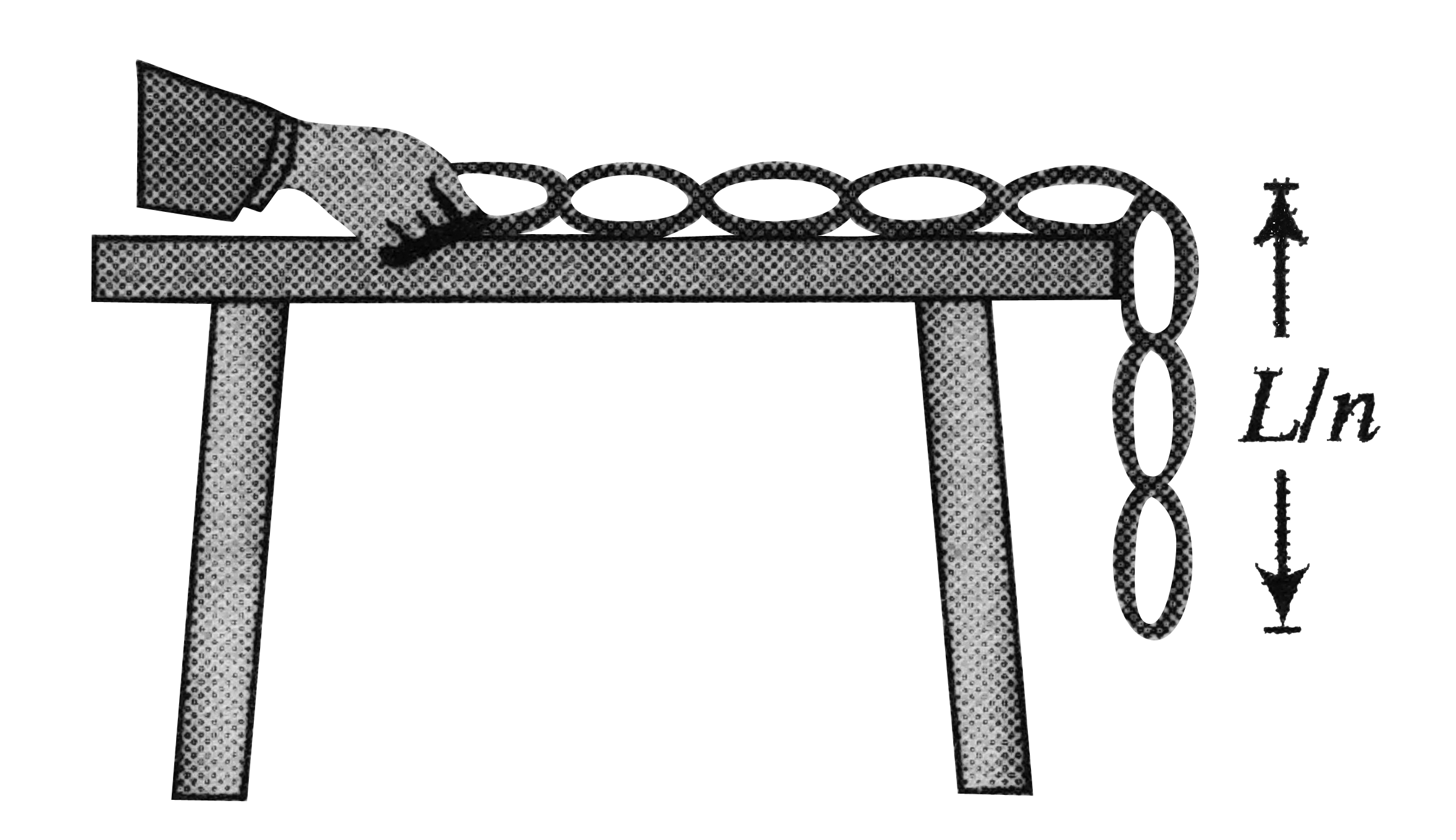
View SolutionA uniform chain of mass M and length L is lying on a frictionless table in such a way that its 1/3 parts is hanging vertically down. The work done in pulling the chain up the table is
View Solution
Knowledge Check
A chain of uniform mass m and length L is held on a frictionless table in such a way that its 1nth part is hanging below the edge of table. The work done to pull the hanging part of chain is :−
A√nBnCn−3Dn−2A uniform chain of length L and mass M is lying on a smooth table and one-third of its length is hanging vertically down over the edge of the table. If g is the acceleration due to gravity, the work required to pull the hanging part on to the table is
AMgLBMgL3CMgL9DMgL18
A uniform chain of length l and mass m is placed on a smooth table with one-fourth of its length hanging over the edge. The work that has to be done to pull the whole chain back onto the table is :
View SolutionA chain is held on a frictionless table with 1/n th of its length hanging over the edge. If the chain has a length L and a mass M, how much work is required to pull the hanging part back on the table?
View SolutionA uniform chain has a mass M and length L. It is placed on a frictionless table with length l0 hanging over the edge. The chain begins to slide down. Ten, the speed v with which the end slides down from the edge is given by
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?
View SolutionA uniform chain of length l and mass m overhangs a smooth table with its two third part lying on the table. Find the kinetic energy of the chain as it completely slips off the table.
View SolutionA uniform chain of length l is placed on rough table with length l/n (where n>1) , hanging over the edge. If the chain just begins to slide off the table by itself from this position, the coefficient of friction between the chain and the table is
View Solution
