Question

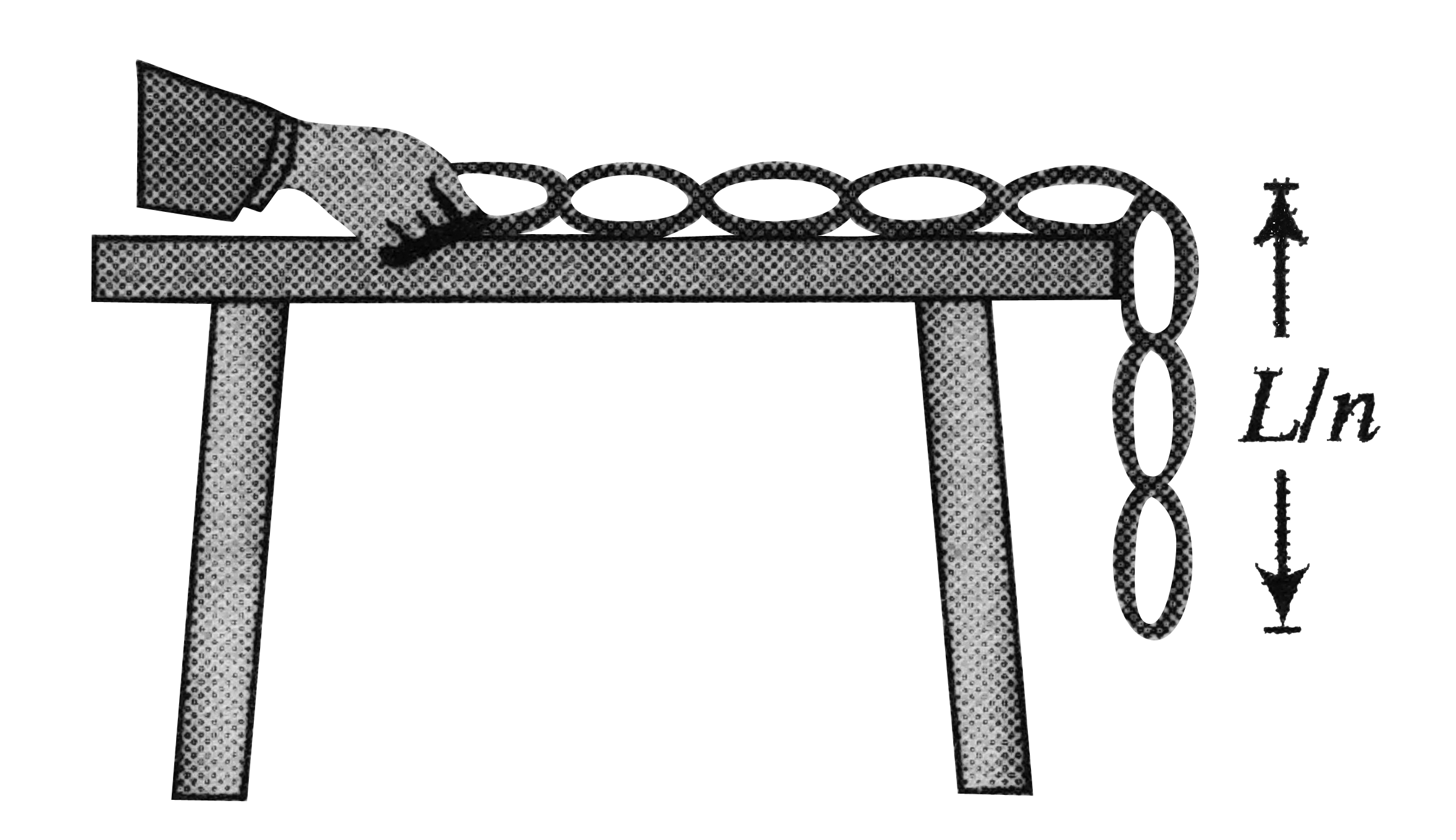
A uniform chain of length $l$ is placed on rough table with length $l/n$ (where $n>1$) , hanging over the edge. If the chain just begins to slide off the table by itself from this position, the coefficient of friction between the chain and the table is

Options

A. `1//n`
B. `1//(n-1)`
C. `1//(n+1)`
D. `(n-1)//(n+1)`

Answer

B

Explanation

To solve the problem, we need to determine the coefficient of friction (μ) between the chain and the table when a uniform chain of length $$l$$ is placed on a rough table of length $$\frac{l}{n}$$ (where $$n>1$$), with a portion of it hanging over the edge.

1. Understanding the Setup:
- The total length of the chain is \( l \).
- The length of the chain on the table is \( \frac{l}{n} \).
- Therefore, the length of the chain hanging over the edge is:
\( l_{\text{hanging}} = l - \frac{l}{n} = l \left(1 - \frac{1}{n}\right) = \frac{l(n-1)}{n} \)

2. Calculating the Mass of the Hanging Part:
- Let the linear mass density of the chain be \( \gamma \) (mass per unit length).
- The mass of the hanging part of the chain is:
\( m_{\text{hanging}} = \gamma \cdot l_{\text{hanging}} = \gamma \cdot \frac{l(n-1)}{n} \)

3. Weight of the Hanging Part:
- The weight of the hanging part is given by:
\( W_{\text{hanging}} = m_{\text{hanging}} \cdot g = \left(\gamma \cdot \frac{l(n-1)}{n}\right) g \)

4. Frictional Force on the Chain:
- The frictional force (\( F_f \)) acting on the chain on the table is given by:
\( F_f = \mu \cdot N \)
- Here, \( N \) is the normal force, which equals the weight of the portion of the chain on the table. The mass of the chain on the table is:
\( m_{\text{on table}} = \gamma \cdot \frac{l}{n} \)
- Thus, the normal force is:
\( N = m_{\text{on table}} \cdot g = \left(\gamma \cdot \frac{l}{n}\right) g \)
- Therefore, the frictional force becomes:
\( F_f = \mu \cdot \left(\gamma \cdot \frac{l}{n} \cdot g\right) \)

5. Setting Up the Equation:
- For the chain to just begin to slide, the weight of the hanging part must equal the frictional force:
\( W_{\text{hanging}} = F_f \)
- Substituting the expressions we derived:
\( \left(\gamma \cdot \frac{l(n-1)}{n}\right) g = \mu \cdot \left(\gamma \cdot \frac{l}{n} \cdot g\right) \)

6. Canceling Common Terms:
- We can cancel \( \gamma \), \( l \), and \( g \) from both sides (assuming they are non-zero):
\( \frac{(n-1)}{n} = \mu \cdot \frac{1}{n} \)

7. Solving for the Coefficient of Friction (μ):
- Rearranging gives:
\( \mu = \frac{(n-1)}{n} \)

Final Answer:
The coefficient of friction between the chain and the table is:
\( \mu = \frac{n-1}{n} \)