A circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is v. If a small transverse disturbance is produced at a point of the loop, with what speed (relative to the string) will this disturbance travel on the string ? Solution in Tamil
m=mass per unit length of the string.
R=Radius of the loop
w=angular velocity
V=Linear velocity of the string
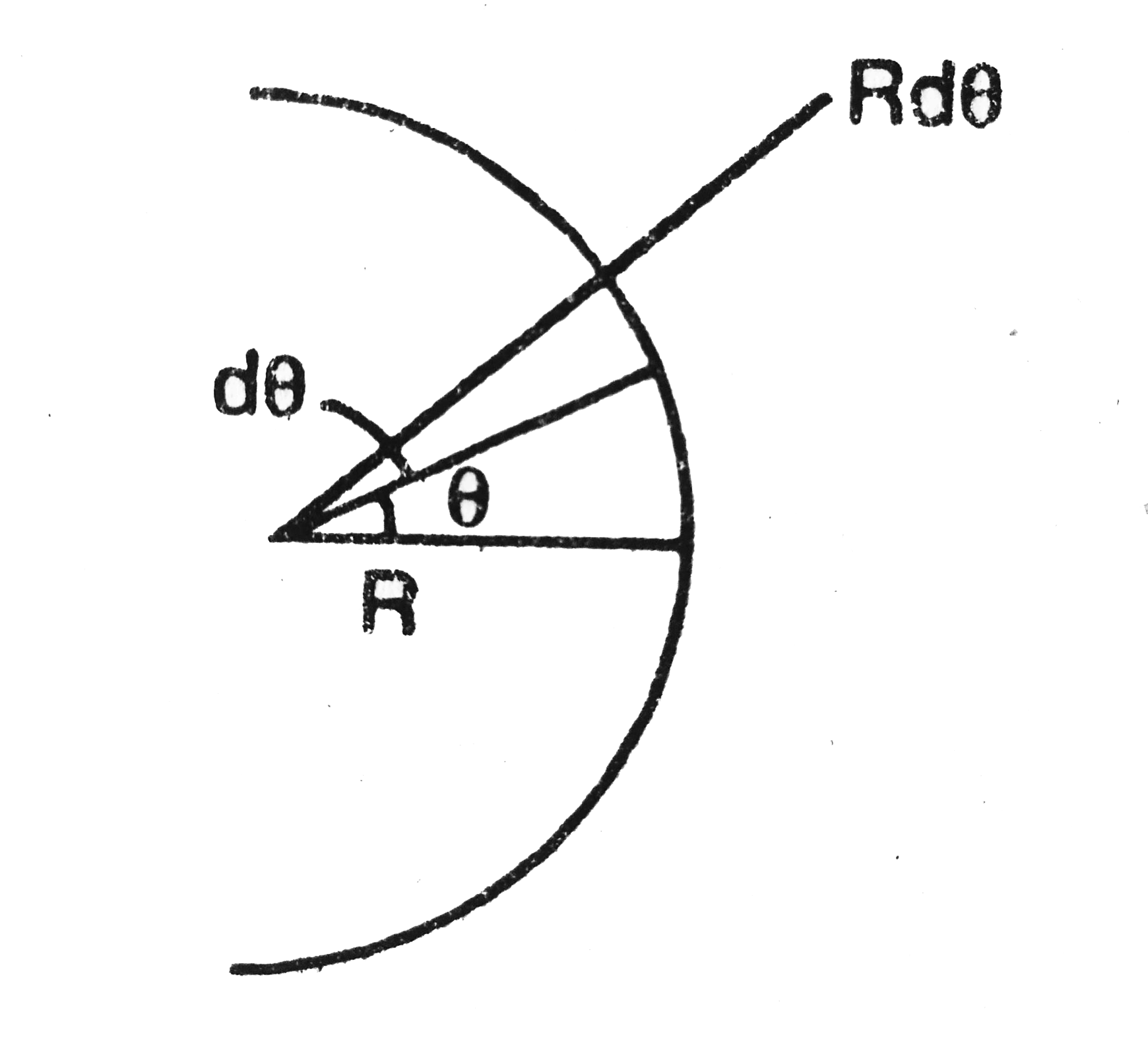
consider one half of the string as shown in ure.
The hal loop experiences centrifugl force at every point, away from centre, which ils balanced by tension 2T.
Consider an elemennt of angular part dthη at angle θ.<br>consra¬here≤mentsymmetric→this.<br>Cenrifugalforceexperiencebythee≤ment=(mRdtheta)w^2R<br>(......Length of element =Rdtheta, mass =mRdtheta)
Resolving in to rectangular components, net force on the two symmetirc elements,
dF=2mR^2dthetaw^2sintheta<br>[Horizontalcompo≠ntscncelseachother]<br>so,→talF=int_0^(pi/2)2mR^2omega^2sithetadtheta<br>=2mR^2omega^2[-costheta]<br>=2mR^2w^2<br>Again 2T=2mr^2w^2<br>rarr T=mR^2w^2<br>velocityoftransversevibration<br>V=sqrt((T/m))<br>=sqrt((mR^2omega^I2)=omegaR=V
Topper's Solved these Questions
Similar Questions
A mechanical wave propagastes in a medium along the X-axis. The particles of the medium moves in what direction?
View SolutionThe gravitational force acting on a particle of 1 g due to a similar particle is equal to 6.67×10−17N. Calculate the separation between the particles.
View SolutionThe mass of a cyclist together with the bicycle is 90 kg. Calculate the work done by cyclist if the speed increases from 6km/h to 12 km/h.
View SolutionA uniform circular hoop of string is rotating clockwise in the absence of gravity. The tangential speed is v_(0) . Find the speed of the wave travelling on this string.
View SolutionA string of length 2L, obeying Hooke's Law, is stretched so that its extension is L. The speed of the tranverse wave travelling on the string is v. if the string is futher stretched so that the extension in the string becomes 4L. The speed of transverse wave travelling on the string will be
View SolutionA loop of a string of mass length μ and redius R is rotated about an axis passing through centre perpendicular to the plane with an angular velocity ω.A small disturbance is created in the loop having the same sense of rotation. The linear speed of the disturbance observer is
View SolutionA particle of mass m is tied to a light string of length l and rotated along a vertical circular path. What should be the minimum speed at the highest point of its path so that the string does not become slack at any position ?
View SolutionA string has a linear mass density mu . The ends of the string are joined to form a closed loop and is given the shape of a circular ring of radius R. this ring is now rotated about its axis with an angular velocity omega Q. The velocity of a transverse wave set up in the string, with respect to the string is
View SolutionA string of mass 0.250 kg is under a tension of 2 N. The length of the stretched string is 2 m. A transverse wave disturbance starts at one end of the string . How long will the disturbance take to reach the other end ?
View SolutionA loop of a string of mass per unit length mu and radius R is rotated about an axis passing through centre perpendicular to the plane with an angular velocityco. A small disturbance is created in the loop having the same sense of rotation. The linear speed of the disturbance for a stationary observer is :
View SolutionA string of mass M as a circular loop rotates abot its axis on a frictionless horizontal plane at a uniform rate so that the tangential plane at a uniform rate so that the tangential speed of any particle of the string is v. If a small transverse disturbance is produced at a point of the loop, then speed (relative to the string) of disturbance on the string is
View SolutionA sinusoidal transverse wave is traveling on a string. Any point on the string
View SolutionA loop of a string of mass per unit length is U and radius R is rotated about an axis passing through centre perpendicular to the plane with an angular velocity ω . A small disturbance is created in the loop having the same sense of rotation. The linear speed of the disturbance for a stationary observer is xRω . Find 'x
View SolutionThe extension in a string obeying Hooke's law is x. The speed of transverse wave in the stretched string is v. If the extension in the string is increased to 1.5x, the speed of transverse wave will be
View SolutionA loop of a string of mass per unit length is |l and radius R is rotated about an axis passing through centre perpendicular to the plane with an angular velocity CO. A small disturbance is created in the loop having the same sense of rotation. The linear speed of the disturbance for a stationary observer is xRomega . Find .x..
View SolutionA string of length I is stretched by II 30 and transverse waves in the string are found to travel at a -speed v_0 . Speed of transverse waves when the string is stretched by II15 will be :
View Solution
