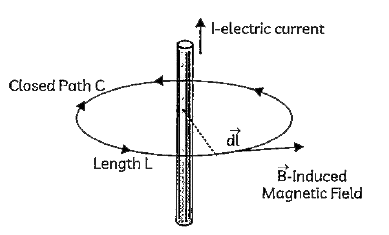
A coaxial cable consists of a thin inner conductor fixed along the axis of a hollow outer conductor. The two conductor carry equal currents in opposite directions. Let be the magnetic fields in the region between the conductors and outside the conductor, respectively. Then,
A
B
C
D
Video Solution
Text Solution
The correct Answer is:C
(c) Apply Ampere's circuital law to
the coaxial circular loops and
. The magnetic field is at all
points on at all points
on for and 0 for .
Hence,
[As ]

|
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICSChapter:SOURCES OF MAGNETIC FIELDExercise:Exercise (multiple Currect )
Explore 5 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICSChapter:SOURCES OF MAGNETIC FIELDExercise:Exercise (assertion-reasioning )
Explore 2 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICSChapter:SOURCES OF MAGNETIC FIELDExercise:Exercise (subjective )
Explore 10 Videos
- SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSBook:CENGAGE PHYSICSChapter:SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSExercise:QUESTION BANK
Explore 12 Videos
- THERMAL PROPERTIES OF MATTERBook:CENGAGE PHYSICSChapter:THERMAL PROPERTIES OF MATTERExercise:Question Bank
Explore 40 Videos
Similar Questions
Knowledge Check
If two conductors carry current in opposite direction, then:Athere is no force between themBforce is repulsiveCforce is attractiveDindependent of forceA coaxial cable consists of a thin inner current carrying conductor fixed along the axis of a hollow current conductor, Let B1 and B2 be the magnetic fields in the region between the conductors, and outside the conductor respectively.
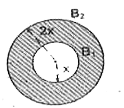 AB1≠0,B2≠0 for conductors carrying equal currents in opposite directionsBB1≠),B2=0, for conductors carrying equal currents in opposite directionsCB1≠0,B2≠0, for conductors carrying equal currents in same directionsDB1=B2 for conductors carrying equal currents in same directions at distance x and 2x from the axis respectively. 2x gt the radius of the outer cable and x lies between the cables.
AB1≠0,B2≠0 for conductors carrying equal currents in opposite directionsBB1≠),B2=0, for conductors carrying equal currents in opposite directionsCB1≠0,B2≠0, for conductors carrying equal currents in same directionsDB1=B2 for conductors carrying equal currents in same directions at distance x and 2x from the axis respectively. 2x gt the radius of the outer cable and x lies between the cables.Two conductors having current in opposite direction………………..
AAttract each otherBRepel each otherCDo not affect each otherDEffect depends on the material of wire
Magnetic Field due to a Current Carrying Conductor
View SolutionIn a coaxial, straght cable, the central conductor and the outer conductor carry equal currents in opposite directions.The magnetic field is non-zero.
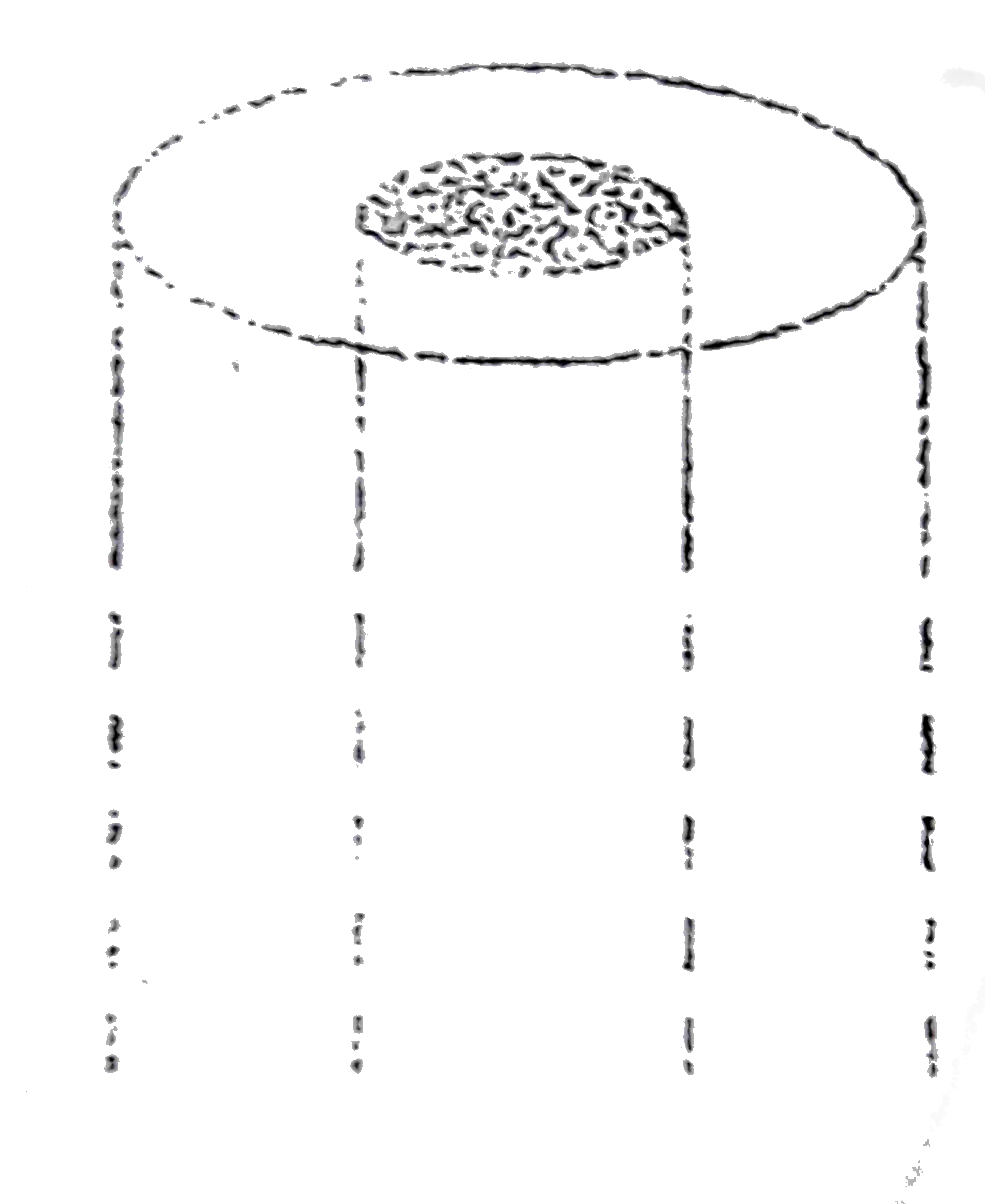
View SolutionA thin, straight conductor lies along the axis of a hollow conductor of radius R. The two carry equal currents in the same direction. The magnetic field B is plotted against the distance r from the axis. Which of the following best represents the resulting curve?
View SolutionThe two linear parallel conductors carrying currents in the opposite direction…………………each other.
View SolutionThe magnetic field near a current carrying conductor is given by
View Solution
