Two positive charges q1andq2 are moving with velocities v1andv2 when they are at points A and B, respectively, as shown in Fig. The magnetic force experienced by charge q1 due to the other charge q2 is
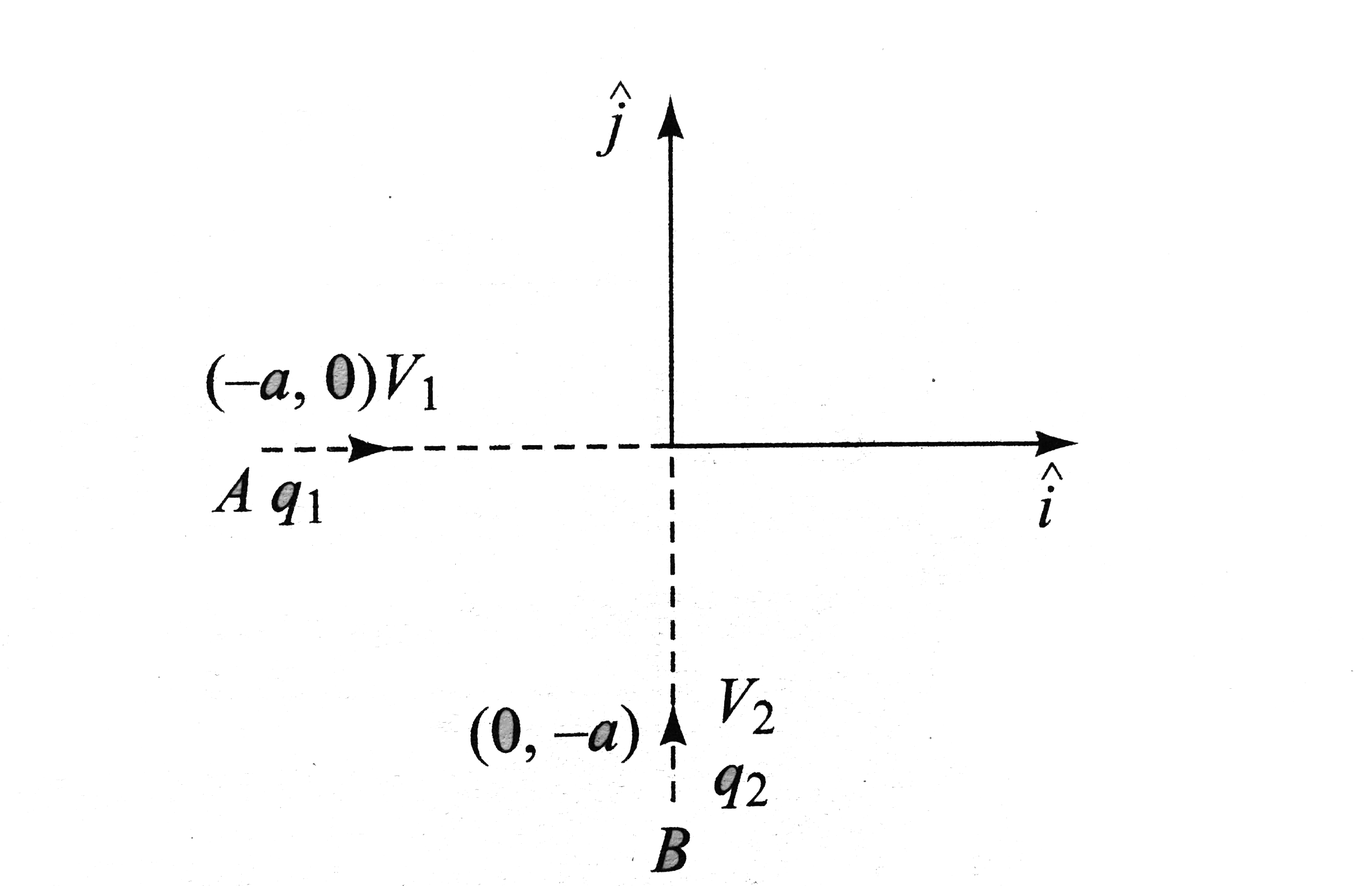
μ0q1q2v1v28√2πa2
μ0q1q2v1v24√2πa2
μ0q1q2v1v22√2πa2
μ0q1q2v1v2√2πa2
The correct Answer is:A
(a) Magnetic field at A due to q2: →B2=μ04πq2v2rsin45∘r3ˆk where r=√2a
⇒→B2=μ04πq2v22√2a2ˆk
Force on q1:→F=q1→v1×→B2=μ04πq1q2v1v22√2a2(−ˆj)
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (multiple Currect )
5 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (assertion-reasioning )
2 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (subjective )
10 Videos
- SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - QUESTION BANK
12 Videos
- THERMAL PROPERTIES OF MATTERBOOK - CENGAGE PHYSICSCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Bank
40 Videos
Similar Questions
Two particles having identical masses and charges 2Q and Q are moving with velocities v and 2v resp. In uniform magnetic field B. Find ratio of radius of circle described by them
View SolutionFour point charges Q , q Q and q are placed at the corners of a square of side 'a' as shown in the Fig. Find the
resultant electric force on a charge Q
View Solution
Knowledge Check
The value of charge q1,q2andq3 as shown in the figure are
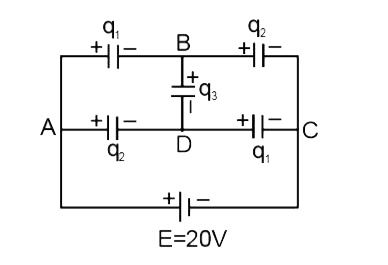 Aq1=32μC,q2=24μC,q3=−8μCBq1=48μc,q2=16μC,q3=+8μCCq1=32μC,q2=24μC,q3=+8μCDq1=3μC,q2=4μC,q3=+2μC
Aq1=32μC,q2=24μC,q3=−8μCBq1=48μc,q2=16μC,q3=+8μCCq1=32μC,q2=24μC,q3=+8μCDq1=3μC,q2=4μC,q3=+2μCThe magnetic Lorentz force experienced by a charge q, entering a magnetic field →B with a velocity v is
A√q(→B×→)Bq(→B×→v)Cq(→v.→B)Dq(→v×→B)Three positive point charges q1,q2,andq3 form an isolated system. Suppose the charges have generated a property due to which like charges also attract. The charges are moving along a circle with same speed maintaining angles as shown in the figure. The charge q1 experiences a force f1 due to other two charges. Similarly, q2 experiences a force f2 and q3, a force f3. The ratio of f1:f2:f3 is
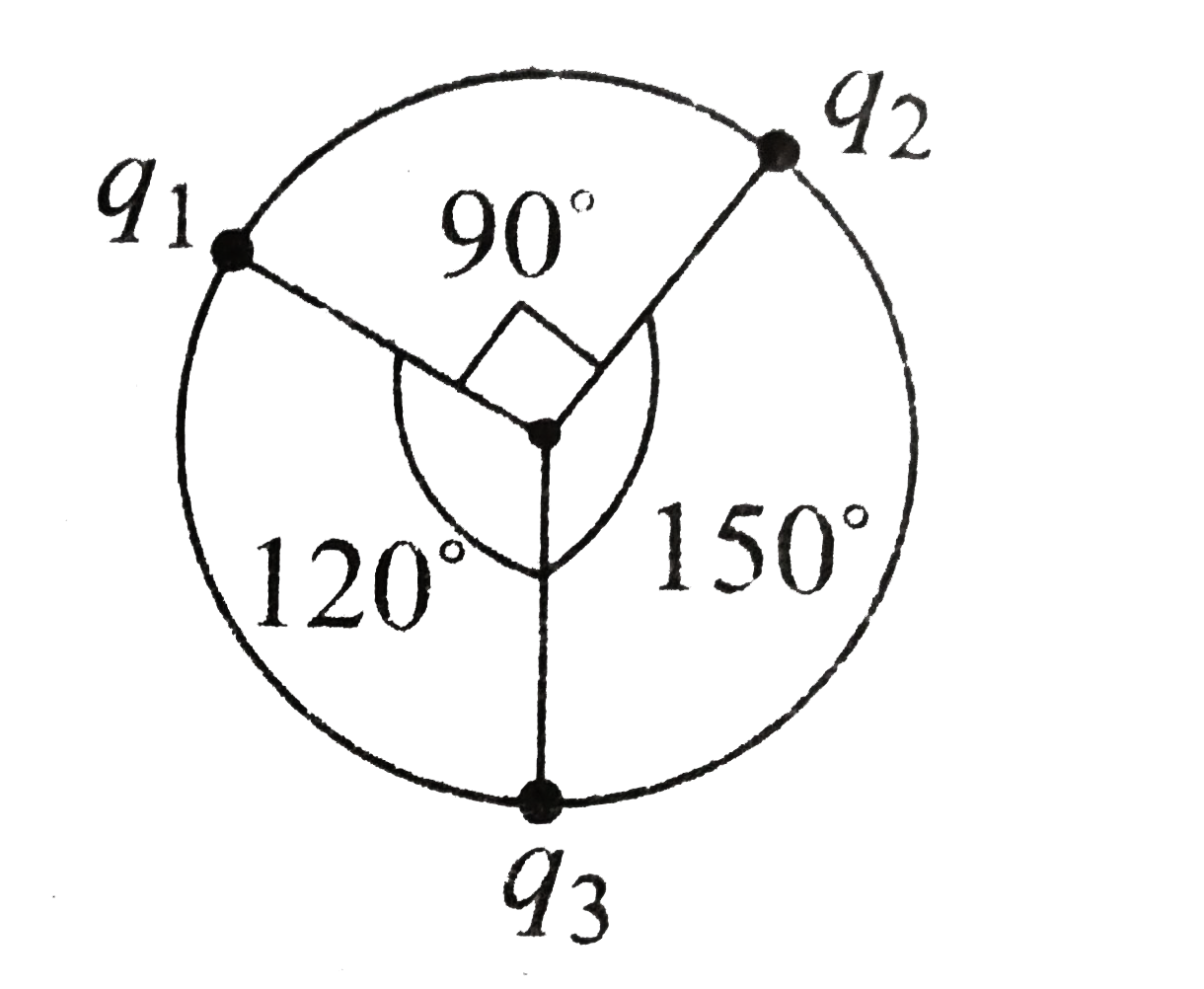 A1:1:1Bq1:q2:q3C1:√3:2DThis ratio cannot be calculated
A1:1:1Bq1:q2:q3C1:√3:2DThis ratio cannot be calculated
Two point like charges q1 and q2 are fixed in free space. At every point on the curve shown, the net electrostatic potential created by these charges is V. Find the separation r between the charges.
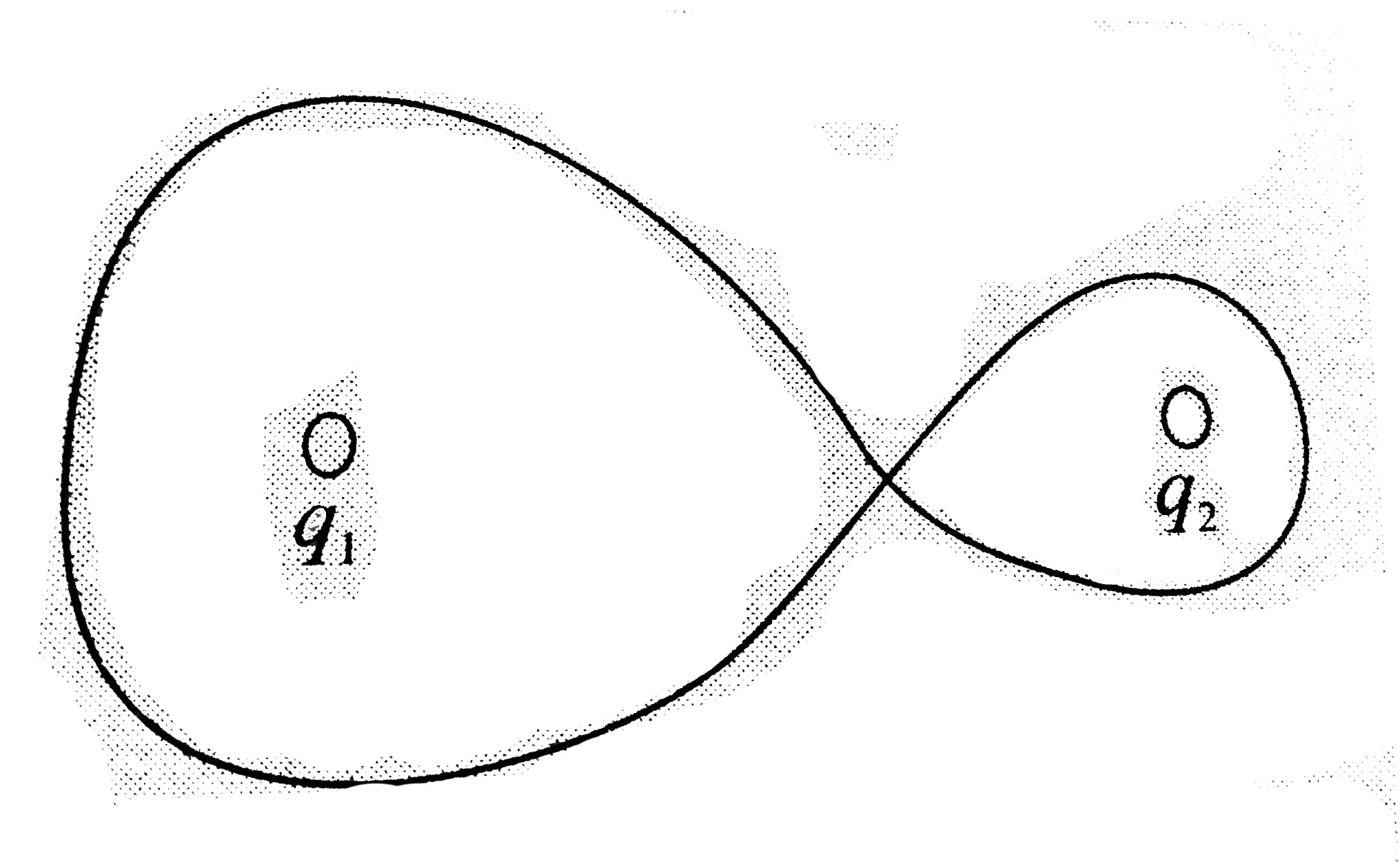 .
.
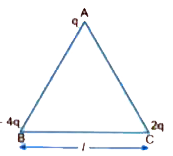
View SolutionThree point charges q,−4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the Fig. Obtain the experssion for the magnitude of the resultant electric force acting on the charge q.
View SolutionState the Biot-Savart law which gives the magnetic field B at a distance r from a current element. Hence obtain an expression for the magnetic field BQ due to a point charge Q moving with constant velocity v (assumed non-relativistic).
Point charge Q and Q' are constrained to move along the x-and y-axes, respectively, with the same uniform speed v. AT time t = 0 both charges are at the origin. At time t calculate the Lorentz force F on Q' due to the magnetic field of Q
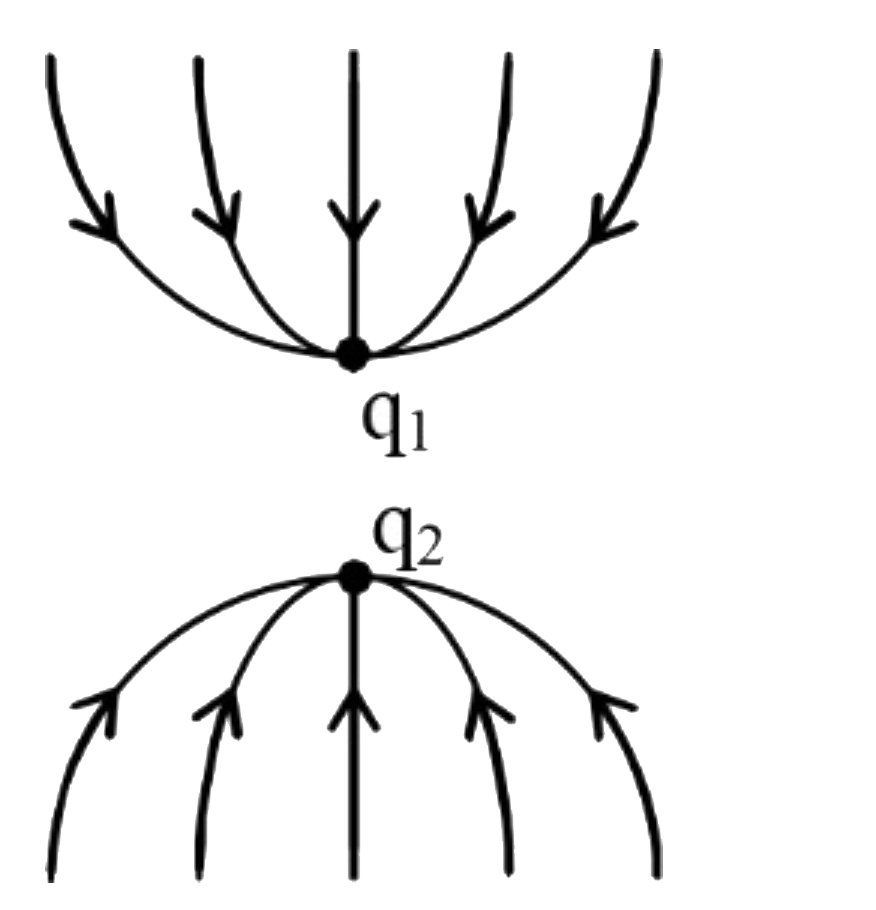
View SolutionIn the adjoining figure, the electric field lines for charges q1andq2 are shown. Identify the sign of the charges.
View SolutionTwo charge +q1 and +q2 placed on x-axis at posiution A and B as shown in figure. Which graph best represent potential as a function of distance r from charge q1

View Solution
