A coil of inductance 1H and resistance 10Ω is connected to a resistanceless battery of emf 50V at time t=0. Calculate the ratio of rthe rate which magnetic energy is stored in the coil to the rate at which energy is supplied by the battery at t=0.1s.
The correct Answer is:C
To solve the problem, we need to calculate the ratio of the rate at which magnetic energy is stored in the coil to the rate at which energy is supplied by the battery at t=0.1s.
1. Identify the given values:
- Inductance L=1H
- Resistance R=10Ω
- EMF of the battery E=50V
2. Calculate the time constant τ:
τ=LR=1H10Ω=0.1s
3. Determine the steady-state current I0:
I0=ER=50V10Ω=5A
4. Find the current I(t) at t=0.1s:
I(t)=I0(1−e−tτ)=5(1−e−0.10.1)=5(1−e−1)
I(0.1)=5(1−1e)
5. Calculate didt:
didt=I0τe−tτ=50.1e−1=50e−1
6. Find the voltage across the inductor VL:
VL=Ldidt=1⋅(50e−1)=50e−1V
7. Calculate the rate at which magnetic energy is stored dUdt:
- The energy stored in the inductor is given by U=12LI2.
- The rate of change of energy is:
dUdt=LIdidt=1⋅(5(1−1e))⋅(50e−1)
dUdt=250(1−1e)e−1
8. Calculate the rate at which energy is supplied by the battery P:
P=E⋅I=50⋅5(1−1e)=250(1−1e)
9. Find the ratio of the rate of magnetic energy storage to the rate of energy supplied:
Ratio=dUdtP=250(1−1e)e−1250(1−1e)=e−1
Final Result:
The ratio of the rate at which magnetic energy is stored in the coil to the rate at which energy is supplied by the battery at t=0.1s is 1e.
- Inductance L=1H
- Resistance R=10Ω
- EMF of the battery E=50V
τ=LR=1H10Ω=0.1s
I0=ER=50V10Ω=5A
I(t)=I0(1−e−tτ)=5(1−e−0.10.1)=5(1−e−1)
I(0.1)=5(1−1e)
didt=I0τe−tτ=50.1e−1=50e−1
VL=Ldidt=1⋅(50e−1)=50e−1V
- The energy stored in the inductor is given by U=12LI2.
- The rate of change of energy is:
dUdt=LIdidt=1⋅(5(1−1e))⋅(50e−1)
dUdt=250(1−1e)e−1
P=E⋅I=50⋅5(1−1e)=250(1−1e)
Ratio=dUdtP=250(1−1e)e−1250(1−1e)=e−1
The ratio of the rate at which magnetic energy is stored in the coil to the rate at which energy is supplied by the battery at t=0.1s is 1e.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 Single Correct37videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 More Than One Correct10videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective Questions3videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
At t=0 , switch S closed. Find the ratio of the rate at which magnetic energy stored in the coil to the rate at which energy is supplied by the battery at time t=τ=L/R .
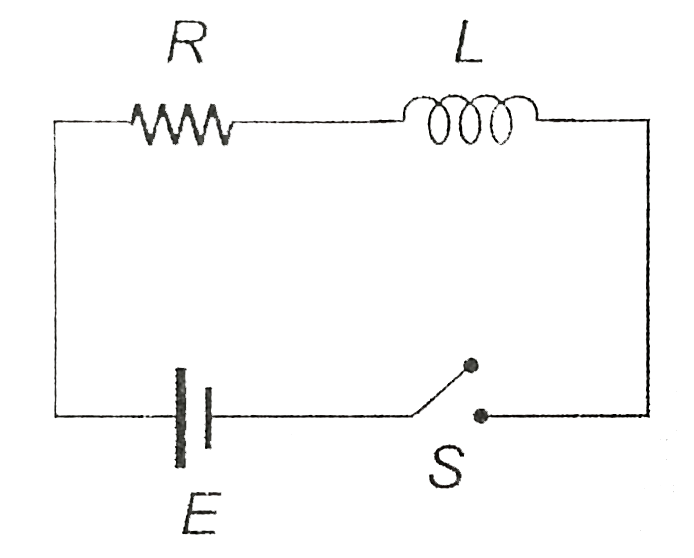 View Solution
View SolutionAn inductor of self-inductance L and resistor of resistance R are connected in series to a battery of emf E and negligible resistance.Calculate the maximum rate at which energy is stored in the inductor.
View Solution
Knowledge Check
- Question 1 - Select One
A coil of inductance 8.4mH and resistance 6 Ω is connected to a 12V battery. The current in the coil is 1.0A at approximately the time.
A500secB20 secC35 millisecD1 millisec - Question 2 - Select One
A coil of inductance 8.4 mH and resistance 6(Ω) is connected to a 12 V battery. The current in the coil is 1.0 A at approximately the time
A500sB25 sC35 sD1 ms - Question 3 - Select One
A coil of inductance 8.4 mH and resistance 6ω is connected to 12 V battery. The current in the coil is 1 A at approximately the time
A500 sB20 sC35 msD1 ms
A coil of inductance 1.0H and resistance 100Ω is connected to a battery of emf 12V. Find the energy stored in the magnetic field associated with the coil at an instant 10ms after the circuit is switched on.
View SolutionA coil of reistance 5Ω and inductance 1H is connected to a battery of 10V. At t=0s, calculate (i) the current in the circuit (ii) the rate of rise of current (iii) the rate at which energy is supplied by the battery, (iv) the rate at which energy is dissipated as heat and (v) the rate at which energy is stored in the magnetic field of the coil.
View SolutionA coil of inductance 8.4 mH and resistance 6ω is connected to 12 V battery. The current in the coil is 1 A at approximately the time
View SolutionA coil of inductance 8.4 mH and resistance 6Ω is connected to a 12 V battery. Approximately how much time it takes to attain a current of 1 A ?
View SolutionA coil of inductance 8.4 mH and resistance 6Ω is connected to a 12 V battery. Approximately how much time it takes to attain a current of 1 A ?
View Solution

