A concave shaving mirror has a radius of curvature of 35.0cm. It is positioned so that the (upright) image of man's face is 2.50 times the size of the face. How far is the mirror from the face ?
5.25cm
21.0cm
10.5cm
42cm
The correct Answer is:C
To solve the problem step by step, we will follow the principles of optics, particularly the mirror formula and magnification.
Step 1: Identify Given Data
- Radius of curvature (R) = 35.0 cm
- Magnification (M) = 2.50 (the image is upright and enlarged)
Step 2: Calculate the Focal Length (F)
The focal length (F) of a concave mirror is given by the formula:
F=R2
Substituting the value of R:
F=35.0cm2=17.5cm
Step 3: Relate Magnification to Object and Image Distances
The magnification (M) is given by the formula:
M=−VU
Where:
- V = Image distance
- U = Object distance
Since the image is upright and enlarged, we can write:
M=HiHo=2.50
This leads to:
2.50=−VU⟹V=−2.50U
Step 4: Use the Mirror Formula
The mirror formula is given by:
1F=1V+1U
Substituting the value of V from the previous step:
117.5=1−2.50U+1U
Step 5: Simplify the Equation
To combine the terms on the right side:
117.5=−1+2.502.50U
This simplifies to:
117.5=1.502.50U
Step 6: Cross Multiply to Solve for U
Cross multiplying gives:
1.50⋅17.5=2.50U
Calculating the left side:
26.25=2.50U
Now, solving for U:
U=26.252.50=10.5cm
Conclusion
The distance of the mirror from the face (U) is 10.5 cm.
---
- Radius of curvature (R) = 35.0 cm
- Magnification (M) = 2.50 (the image is upright and enlarged)
The focal length (F) of a concave mirror is given by the formula:
F=R2
Substituting the value of R:
F=35.0cm2=17.5cm
The magnification (M) is given by the formula:
M=−VU
Where:
- V = Image distance
- U = Object distance
M=HiHo=2.50
This leads to:
2.50=−VU⟹V=−2.50U
The mirror formula is given by:
1F=1V+1U
Substituting the value of V from the previous step:
117.5=1−2.50U+1U
To combine the terms on the right side:
117.5=−1+2.502.50U
This simplifies to:
117.5=1.502.50U
Cross multiplying gives:
1.50⋅17.5=2.50U
Calculating the left side:
26.25=2.50U
Now, solving for U:
U=26.252.50=10.5cm
The distance of the mirror from the face (U) is 10.5 cm.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Reflection Of Light By Spherical Mirrors
5 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Refraction
10 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSERTION AND REASON
15 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 3
50 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A convex mirror has its radius of curvature 20 cm. Find the position of the image of an object placed at a distance of 12 cm from the mirror.

View SolutionA concave mirror has a radius of curvature of 24 cm. How far is
an object from the mirror if an image is formed that is:
(a) virtual and 3.0 times the size of the object,
(b) real and 3.0 times the size of the object and
(c) real and 1/3 the size of the object?.
View SolutionA concave mirror having a radius of curvature 40 cm is placed in front of an illuminated point source at a distance of 30 cm from it. Find the location of the image.
View SolutionAn object 5 cm tall is placed 1 m from a concave spherical mirror which has a radius of curvature of 20 cm. The size of the image is
View SolutionAn object 5 cm tall is placed 1 m from a concave spherical mirror which has a radius of curvature of 20 cm. The size of the image is
View Solution(i) Calculate the distance of an object of height h from a concave mirror of radius of curvature 20 cm , so as to obtain a real image of magnification 2. find the location of image also .
(iii) Using mirror formula explain Why does a convex always produce a virtual image .
View SolutionA concave mirror has a focal length of 20 cm. Find the position or positions of an object for which the image-size is double of the object-size.
View SolutionAn object is placed 21 cm in fron of a concave mirror of radius of curvature 20 cm.A glass slab of thicknes 3 cm and refractive index 1.5 is palced close to the mirror in the space between the object and the mirror. Find the position of the final image fromed. The distance of the nearer surface of the slab from the mirror is 10 cm.
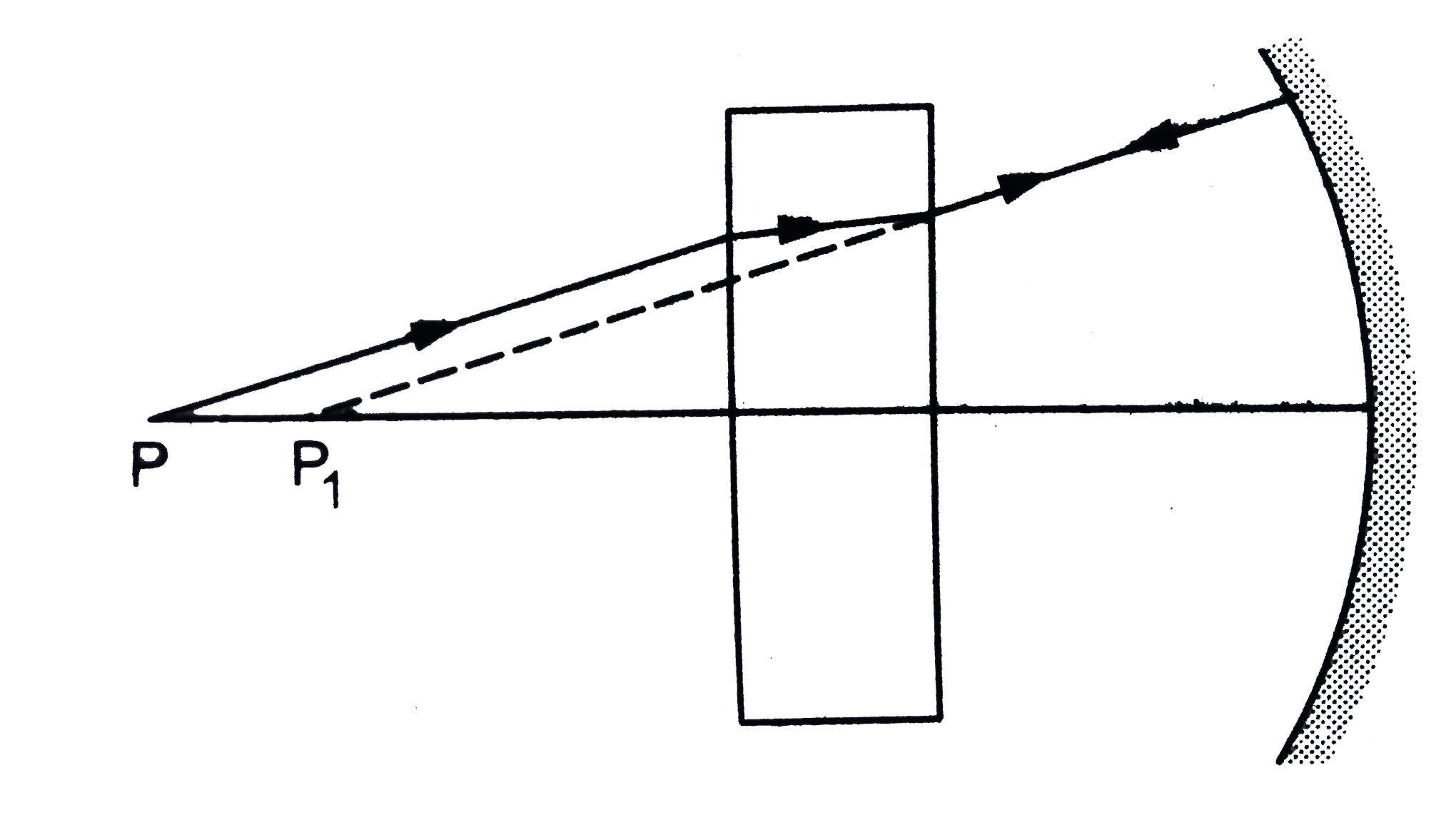
View SolutionA concave mirror has a focal length 20 cm. The distance between the two positions of the object for which the image size is double of the object size is
View SolutionA convex mirror used for rear view on an automobile has a radius of curvature of 3.00 m. If a bus is located at 5.00 m from this mirror, find the position, nature and size of the image.
View Solution
