Light travels in two media A and B with speeds 1.8×108ms–1and2.4×108ms–1 respectively. Then the critical angle between them is
sin−1(23)
tan−1(34)
tan−1(23)
sin−1(34)
The correct Answer is:D
To find the critical angle between two media A and B, we can use the relationship between the speeds of light in the two media and their refractive indices.
1. Identify the speeds of light in the two media:
- Speed of light in medium A, VA=1.8×108m/s
- Speed of light in medium B, VB=2.4×108m/s
2. Calculate the refractive indices of the two media:
- The refractive index n of a medium is given by the formula:
n=cV
where c is the speed of light in vacuum (approximately 3×108m/s).
- For medium A:
nA=cVA=3×1081.8×108=31.8=53
- For medium B:
nB=cVB=3×1082.4×108=32.4=54
3. Use the formula for critical angle:
- The critical angle θc can be found using Snell's law:
sinθc=nBnA
- Substituting the values of the refractive indices:
sinθc=5453=54×35=34
4. Calculate the critical angle:
- To find the critical angle θc, take the inverse sine:
θc=sin−1(34)
Final Answer:
The critical angle between the two media is:
θc=sin−1(34)
- Speed of light in medium A, VA=1.8×108m/s
- Speed of light in medium B, VB=2.4×108m/s
- The refractive index n of a medium is given by the formula:
n=cV
where c is the speed of light in vacuum (approximately 3×108m/s).
- For medium A:
nA=cVA=3×1081.8×108=31.8=53
- For medium B:
nB=cVB=3×1082.4×108=32.4=54
- The critical angle θc can be found using Snell's law:
sinθc=nBnA
- Substituting the values of the refractive indices:
sinθc=5453=54×35=34
- To find the critical angle θc, take the inverse sine:
θc=sin−1(34)
The critical angle between the two media is:
θc=sin−1(34)
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Reflection Of Light By Spherical Mirrors5videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Refraction10videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSERTION AND REASON15videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 350videos
PRACTICE PAPPER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS EXERCISE - Assertion And Reason15videos
SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS
Similar Questions
The velocities of light in two different media are 2*108m/s and 2.5 * 108m/s respectively.The critical angle for these media is
View SolutionA body travelling with uniform acceleration crosses two point A and B with velocities 20ms−1 and 30ms−1 respectively. The speed of the body at the mid-point of A and B is.
View SolutionThe speed of light in media M1 and M2 are 1.5×108m/sand2.0×108m/s respectively. A ray of light enters from medium M1 to M2 at an incidence angle i. If the ray suffers total internal reflection, the value of i is.
View SolutionTwo particles A and B are thown vertically upward with velocity, vertically upward with velocity, 5ms−1 and 10ms−1 respectively (g=10 m s^(-2)), Find separation between them after 1s.
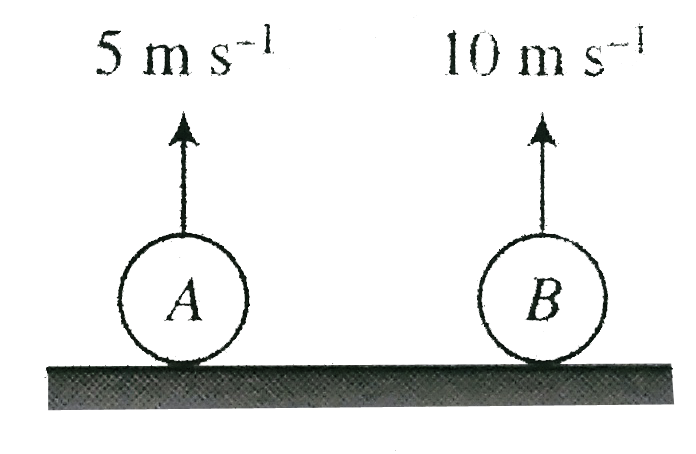 .View Solution
.View SolutionTwo transparent media A and B are separated by a plane boundary. The speed of light in medium A is 2.0×108ms−1 and in medium B is 2.5×108ms−1. The critical angle for which a ray of light going from A to B suffers total internal reflection is
View SolutionTwo transparent media A and B are separated by a plane boundary. The speed of light in medium A is 2.0×108ms−1 and in medium B is 2.5×108ms−1. The critical angle for which a ray of light going from A to B suffers total internal reflection is
View SolutionThe wavelengths for the light of red and blue colours are nearly 7.8×10−7mand4.8×10−7 m respectively.
(a) Which colour has greater speed in vacuum ?
(b) Which colour has greater speed in glass?View SolutionThe refractive index of glass with respect to water is 98. If the velocity of wavelength of light in glass are 2×108m/s and 4000 Å respectively, find the velocity and wavelength of light in water.
View Solution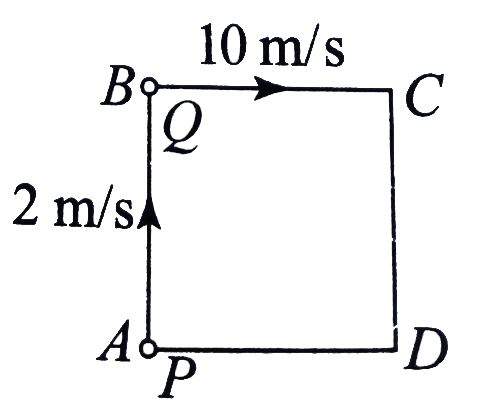
Two men P & Q are standing at corners A & B of square ABCD of side 8m. They start moving along the track with constant speed 2m/s and 10m/s respectively. Find the time when they will meet for the first timeView SolutionTwo particles A and B start moving simultaneously along the line joining them in the same direction with acceleration of 1m/s2and2m/s2 and speeds 3m/sand1m/s respectively. Initially, A is 10 m behind B. What is the minimum distance between them?
View Solution

