A converging bundle of rays travel from water (refractive index 4/3 ) to glass (refractive index 1.5) through a convex interface of radius 16cm. In the absence of the interface, The rays would have converged to a point 32 cm from the pole of the interface. Where will the rays actually meet after refraction?
To solve the problem, we will use the formula derived from the lens maker's equation for refraction at a spherical surface. The formula is given by:
n2V−n1U=n2−n1R
Where:
- n1 is the refractive index of the medium from which the rays are coming (water, 43).
- n2 is the refractive index of the medium into which the rays are entering (glass, 1.5).
- U is the object distance (the distance from the pole of the interface to the point where rays would converge without the interface, which is 32cm).
- V is the image distance (the distance from the pole of the interface to the point where the rays actually meet after refraction).
- R is the radius of curvature of the convex surface (given as 16cm).
Step 1: Identify the values
- n1=43
- n2=1.5
- U=−32cm (the object distance is taken as negative in the lens formula)
- R=16cm
Step 2: Substitute the values into the formula
1.5V−43−32=1.5−4316
Step 3: Simplify the equation
First, calculate n2−n1:
1.5−43=32−43=96−86=16
Now substitute this back into the equation:
1.5V+43×32=1616
Step 4: Calculate 43×32
496=124
Step 5: Substitute and simplify
Now we have:
1.5V+124=196
Step 6: Isolate 1.5V
1.5V=196−124
Step 7: Find a common denominator and simplify
The common denominator for 96 and 24 is 96:
124=496
So,
1.5V=1−496=−396=−132
Step 8: Solve for V
1.5V=−132⟹V=−1.5×32=−48cm
Conclusion
The rays will actually meet at a distance of 48cm on the same side as the object, indicating that the image is virtual.
- n1 is the refractive index of the medium from which the rays are coming (water, 43).
- n2 is the refractive index of the medium into which the rays are entering (glass, 1.5).
- U is the object distance (the distance from the pole of the interface to the point where rays would converge without the interface, which is 32cm).
- V is the image distance (the distance from the pole of the interface to the point where the rays actually meet after refraction).
- R is the radius of curvature of the convex surface (given as 16cm).
- n1=43
- n2=1.5
- U=−32cm (the object distance is taken as negative in the lens formula)
- R=16cm
1.5V−43−32=1.5−4316
First, calculate n2−n1:
1.5−43=32−43=96−86=16
1.5V+43×32=1616
496=124
Now we have:
1.5V+124=196
1.5V=196−124
The common denominator for 96 and 24 is 96:
124=496
So,
1.5V=1−496=−396=−132
1.5V=−132⟹V=−1.5×32=−48cm
The rays will actually meet at a distance of 48cm on the same side as the object, indicating that the image is virtual.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.615videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.721videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.412videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.315videos
ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit27videos
HEATING EFFECT OF CURRENT
Similar Questions
A converging set of ray, traveling from water to air, is incident on a plane interface. In the absence of the interface, the rays would have converged to a point O, 60 cm above the interface. However, dur to refraction the rays will bend. At what distance above the interface will the rays actually converge?
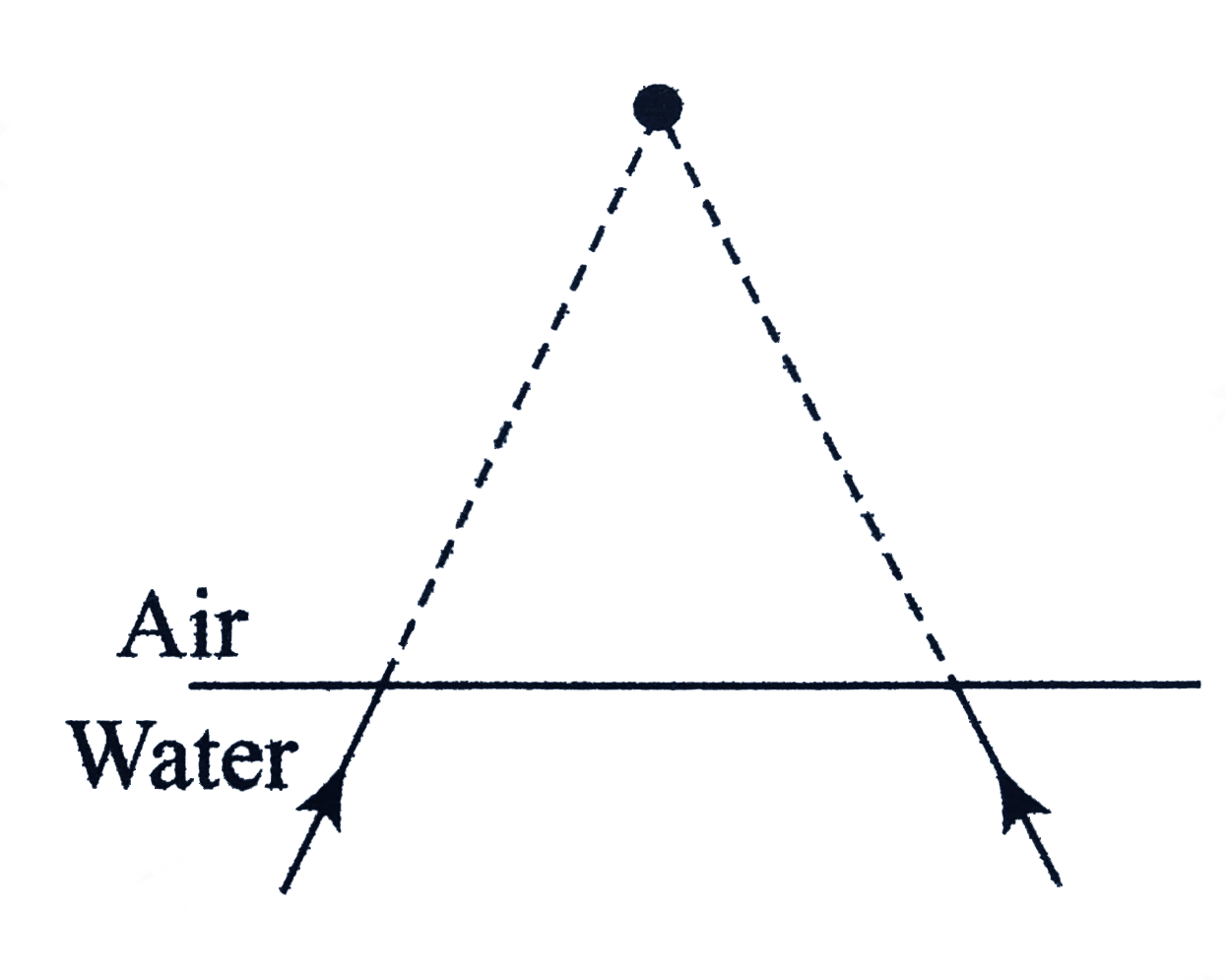 View Solution
View SolutionA converging beam of light is incident on a concave lens of focal length 20 cm. In the absence of the lens, the beam converges at a point 10 m behind the lens. Find where the beam will converge/diverge after refraction.
View SolutionA denser medium of refractive index 1.5 has a concave surface of radius of curvature 12 cm. An object is situated in the denser medium at a distance of 9 cm from the pole. Locate the image due to refraction in air.
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
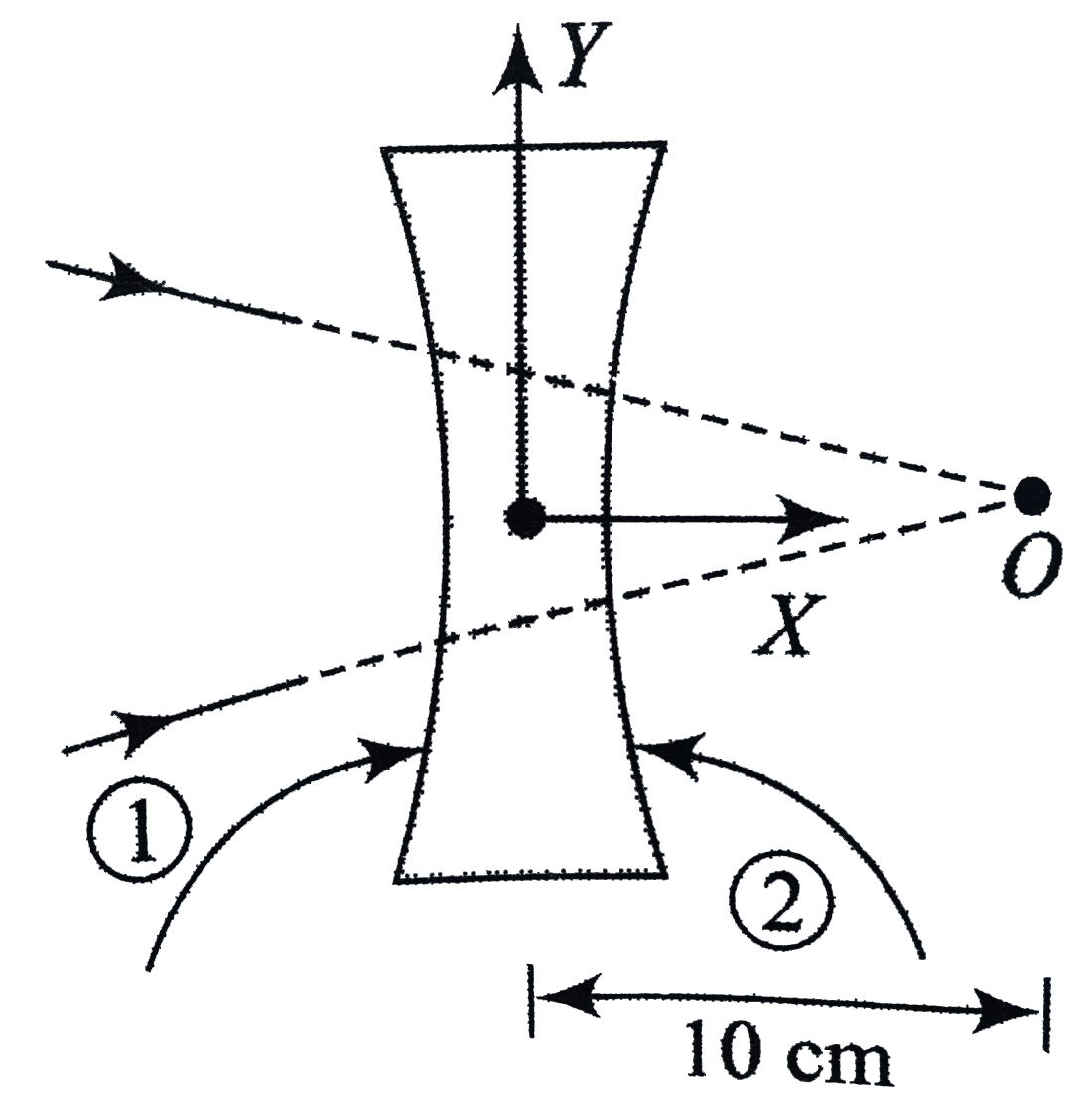 View Solution
View SolutionA plano-convex lens has refractive index 1.6 and radius of curvature 60 cm. What is the focal length of the lens?
View SolutionA ray of light passes from a medium A having refractive index 1.6 to the medium B having refractive index 1.5. The value of critical angle of medium
A is….View SolutionA double convex thin lens made of glass (refractive index μ=1.5) has both radii of curvature of magnitude 20 cm . Incident light rays parallel to the axis of the lens will converge at a distance L such that
View SolutionA double convex thin lens made of glass (refractive index μ=1.5) has both radii of curvature of magnitude 20 cm . Incident light rays parallel to the axis of the lens will converge at a distance L such that
View SolutionA converging lens of refractive index 1.5 is kept in a liquid medium having the same refractive index. What would be the focal length of the lens in the medium ?
View SolutionIn a medium of refractive index 1.6 and having a convex surface has a point object in it at a distance of 12 cm from the pole. The radius of curvature is 6 cm. Locate the image as seen from air
View Solution

