A converging lens is used to obtain an image of an object placed in front of it. The inverted image is formed between of the lens.
Where is the object placed ?
To solve the problem, we need to analyze the situation involving a converging lens and the formation of an image. Here are the steps to find out where the object is placed:
Step 1: Understand the Lens and Image Formation
A converging lens (convex lens) can form images based on the position of the object relative to the focal points (F) and twice the focal points (2F). The lens has two focal points: F1 (for the object side) and F2 (for the image side).
Step 2: Identify the Image Location
According to the problem, the inverted image is formed between F2 and 2F2. This means the image is located in the region closer to the lens than 2F2 but farther than F2.
Step 3: Apply the Lens Formula
The lens formula is given by:
1f=1v−1u
where:
- f = focal length of the lens
- v = image distance (positive if the image is on the opposite side of the object)
- u = object distance (negative as per sign convention for real objects)
Step 4: Determine Object Position
For a real and inverted image to be formed between F2 and 2F2, the object must be placed beyond 2F1 (the object side). This is because:
- If the object is placed between F1 and the lens, the image would be virtual and upright.
- If the object is placed at F1, the image would be at infinity.
- If the object is placed beyond 2F1, the image will be real, inverted, and located between F2 and 2F2.
Conclusion
Thus, the object must be placed beyond 2F1 to ensure that the image is formed between F2 and 2F2.
Final Answer
The object should be placed beyond 2F1.
---
A converging lens (convex lens) can form images based on the position of the object relative to the focal points (F) and twice the focal points (2F). The lens has two focal points: F1 (for the object side) and F2 (for the image side).
According to the problem, the inverted image is formed between F2 and 2F2. This means the image is located in the region closer to the lens than 2F2 but farther than F2.
The lens formula is given by:
1f=1v−1u
where:
- f = focal length of the lens
- v = image distance (positive if the image is on the opposite side of the object)
- u = object distance (negative as per sign convention for real objects)
For a real and inverted image to be formed between F2 and 2F2, the object must be placed beyond 2F1 (the object side). This is because:
- If the object is placed between F1 and the lens, the image would be virtual and upright.
- If the object is placed at F1, the image would be at infinity.
- If the object is placed beyond 2F1, the image will be real, inverted, and located between F2 and 2F2.
Thus, the object must be placed beyond 2F1 to ensure that the image is formed between F2 and 2F2.
The object should be placed beyond 2F1.
Topper's Solved these Questions
Similar Questions
A converging lens is used to obtain an image of an object placed in front of it. The inverted image is formed between F2and2F2 of the lens.
Draw a ray diagram to illustrate the formation of the image obtained.
View SolutionA convex lens has a focal length of 20 cm. It is used to form an image of an object placed 15 cm from lens. The image is
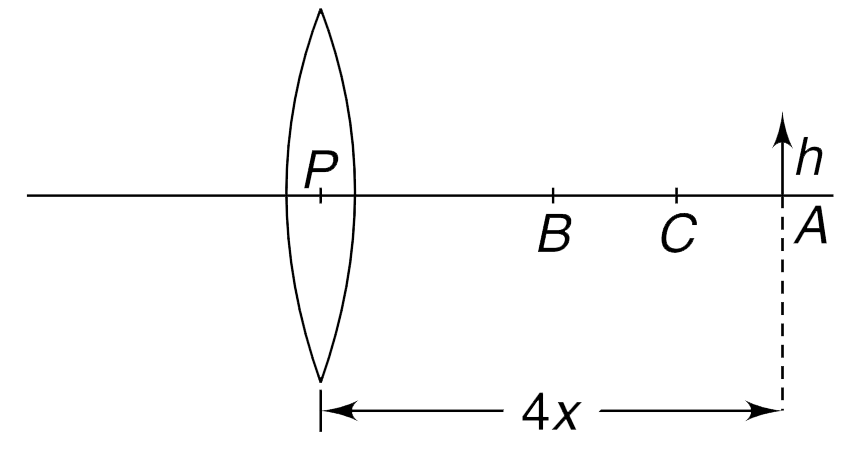
View SolutionA thin converging lens forms a real image of an object located far away from the lens. The image is formed at A at a distance 4x from the lens and height of the image is h. A thin diverging lens of focal length x is placed at B[PB=2x] and a converging lens of focal length 2x is placed at C[PC=3x]. The principal axes of all lenses coincide. Find the height of the final image formed.
View SolutionA convex lens is used to obtain a magnified image of an object on a screen . The object is at a distance 10 m from the lens . If the magnification is 19. the focal length of the lens is
View SolutionA convex lens made of glass (μg=3/2) has focal length f in air. The image of an object placed in front of it is inverted, real and magnified. Now the whole arrangement distance between object and lens. Then
View SolutionWhere should an object be placed in front of a convex lens to get a real to get real image of the size of the object ?
View SolutionTwo converging lenses have focal length f1andf2 The optical axes of the two lenses coincide. This lens system is used to form an image of an object . It is observed that the size of the image does not depend on the distance between the system and object . The distance between lenses is
View SolutionAn object is placed at 10 cm from a lens and real image is formed with magnification of 0.5. Then the lens is
View SolutionWhich lens can produce a real and inverted image of an object ?
View SolutionWhere should an object be placed in front of a convex lens in order to get :
an enlarged real image
View Solution
