A convex spherical refracting surface with radius R separates a medium having refractive index 5/2 from air. As an object is moved towards the surface from far away from the surface along the principle axis, its image
changes from real to virtual when it is at a distance R from the surface
changes from virtual to real when it is at a distance R from the surface
changes from real to virtual when it is at a distance 2R/3 from the surface
changes from virtual to real when it is at a distance 2R/3 from the surface
The correct Answer is:c.
To solve the problem, we need to analyze the behavior of the image formed by a convex spherical refracting surface as an object approaches it. The surface separates a medium with a refractive index of 52 (or 2.5) from air (with a refractive index of 1).
1. Identify the Refractive Indices:
- Let μ1=1 (refractive index of air).
- Let μ2=52=2.5 (refractive index of the medium).
2. Sign Convention:
- For a convex surface, the radius of curvature R is positive.
- The object distance u is considered negative as per the sign convention (since the object is on the same side as the incoming light).
3. Use the Refraction Formula:
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
Substituting the values of μ1 and μ2:
2.5v−1u=2.5−1R
Simplifying:
2.5v−1u=1.5R
4. Rearranging the Equation:
Rearranging gives:
2.5v=1.5R+1u
Taking the LCM:
2.5v=1.5u+RRu
Thus,
v=2.5Ru1.5u+R
5. Determine the Image Nature:
- Initially, when the object is far away (i.e., u→−∞), the image is real and formed on the opposite side of the surface.
- As the object moves closer, we need to check the nature of the image at specific distances.
6. Check for u=R:
Substituting u=−R:
v=2.5R(−R)1.5(−R)+R=−2.5R2−0.5R=5R
Since v is positive, the image is real.
7. Check for u=2R3:
Substituting u=−2R3:
v=2.5R(−2R3)1.5(−2R3)+R=−5R23−R=5R3
Here, v is positive, indicating a real image.
8. Conclusion:
The image changes from real to virtual when the object is at a distance of 2R3 from the surface. Thus, the correct option is that the image changes from real to virtual at u=2R3.
Final Answer:
The image changes from real to virtual when the object is at a distance of 2R3 from the surface.
- Let μ1=1 (refractive index of air).
- Let μ2=52=2.5 (refractive index of the medium).
- For a convex surface, the radius of curvature R is positive.
- The object distance u is considered negative as per the sign convention (since the object is on the same side as the incoming light).
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
Substituting the values of μ1 and μ2:
2.5v−1u=2.5−1R
Simplifying:
2.5v−1u=1.5R
Rearranging gives:
2.5v=1.5R+1u
Taking the LCM:
2.5v=1.5u+RRu
Thus,
v=2.5Ru1.5u+R
- Initially, when the object is far away (i.e., u→−∞), the image is real and formed on the opposite side of the surface.
- As the object moves closer, we need to check the nature of the image at specific distances.
Substituting u=−R:
v=2.5R(−R)1.5(−R)+R=−2.5R2−0.5R=5R
Since v is positive, the image is real.
Substituting u=−2R3:
v=2.5R(−2R3)1.5(−2R3)+R=−5R23−R=5R3
Here, v is positive, indicating a real image.
The image changes from real to virtual when the object is at a distance of 2R3 from the surface. Thus, the correct option is that the image changes from real to virtual at u=2R3.
The image changes from real to virtual when the object is at a distance of 2R3 from the surface.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A concave spherical refractive surface with radius R separates a medium of refractive index 5/2 from air. As an object is approaching the surface from far away from the surface along the central axis, its image
View SolutionA concave spherical surface of radius of curvature 100 cm separates two media of refractive indices 1.50 and 43. An object is kept in the first medium at a distance of 30 cm from the surface. Calculate the position of the image.
View Solution
Knowledge Check
A convex refracting surface of radius of curvature 20cm separates two media of refractive indices 4/3and1.60. An object is placed in the first medium (μ=4/3) at a distance of 200cm from the refracting surface. Calculate the position of image formed.
A120cmB240cmC100cmD60cm
A convex surface of radius of curvature 40 cm separate two media of refractive indices 43 and 1.50. An object is kept in the first medium at a distance of 20 cm from the surface. Calculate the position of the image.
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
View SolutionA concave refractive surface of a medium having refractive index μ produces a real image of an object (located outside the medium) irrespective of its location. Choose the correct option from the following.
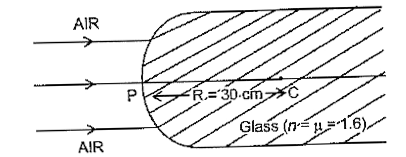
View SolutionFigure below shows a parallel beam of monochromatic light incident on a convex spherical surface, radius of curvature R = 30 cm, which separates glass (refractive index - 1 6) from air. Find the position of the image formed due to refraction of light at this single spherical surface.
View SolutionIf a ray of light is incident from rarer medium at an angle 45° on the surface which separates two medium having refractive indices 1 and √2 for rarer and denser medium, then angle of deviation of refractive ray with incident ray is
View SolutionA glass hemisphere of radius R and of material having refractive index 1.5 is silvered on its flat face as shown in figure., A small object of height h is located at a distance 2R from the surface of hemisphere as shown in the figure. The final image will form.
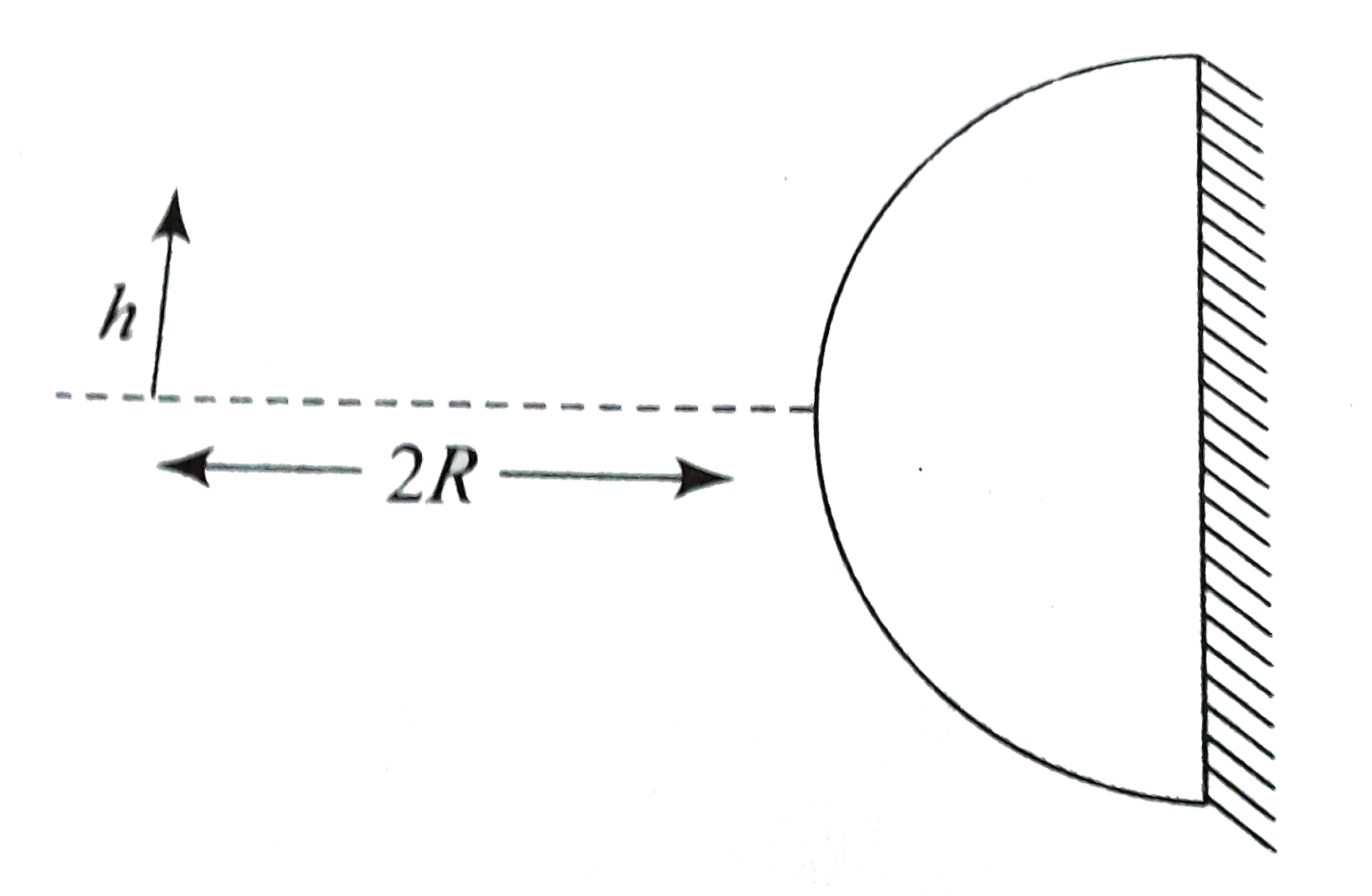
View Solution
