A concave spherical refractive surface with radius R separates a medium of refractive index 5/2 from air. As an object is approaching the surface from far away from the surface along the central axis, its image
always remains real
always remains virtual
changes from real to virtual at a distance 2R/3 from the surface
changes from virtual to real at a distance 2R/3 from the surface
The correct Answer is:b.
To solve the problem, we need to analyze the situation using the lens maker's formula for a spherical refractive surface. We will determine whether the image formed is real or virtual as the object approaches the surface.
1. Identify the Given Information:
- Refractive index of the medium (μ2) = 5/2 = 2.5
- Refractive index of air (μ1) = 1
- Radius of curvature of the concave surface (R) = R (given)
2. Sign Convention:
- Since the object is approaching the surface from far away, we consider the object distance (U) to be negative (U < 0).
- The radius of curvature (R) for a concave surface is also considered negative (R < 0).
3. Apply the Refraction Formula:
The formula relating the refractive indices and distances is given by:
μ2V−μ1U=μ2−μ1R
Substituting the values:
2.5V−1U=2.5−1R
Simplifying further:
2.5V−1U=1.5R
4. Rearranging the Equation:
Rearranging gives:
2.5V=1.5R+1U
Now, we can express V:
1V=1.52.5R+12.5U
Cross-multiplying gives:
V=−2.5RU1.5U+R
5. Analyzing the Sign of V:
Since both R and U are negative, the expression for V will also be negative:
V<0
A negative value for V indicates that the image is virtual.
6. Conclusion:
As the object approaches the concave surface from far away, the image formed is always virtual.
Final Answer:
The image is virtual.
---
- Refractive index of the medium (μ2) = 5/2 = 2.5
- Refractive index of air (μ1) = 1
- Radius of curvature of the concave surface (R) = R (given)
- Since the object is approaching the surface from far away, we consider the object distance (U) to be negative (U < 0).
- The radius of curvature (R) for a concave surface is also considered negative (R < 0).
The formula relating the refractive indices and distances is given by:
μ2V−μ1U=μ2−μ1R
Substituting the values:
2.5V−1U=2.5−1R
Simplifying further:
2.5V−1U=1.5R
Rearranging gives:
2.5V=1.5R+1U
Now, we can express V:
1V=1.52.5R+12.5U
Cross-multiplying gives:
V=−2.5RU1.5U+R
Since both R and U are negative, the expression for V will also be negative:
V<0
A negative value for V indicates that the image is virtual.
As the object approaches the concave surface from far away, the image formed is always virtual.
The image is virtual.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A convex spherical refracting surface with radius R separates a medium having refractive index 5/2 from air. As an object is moved towards the surface from far away from the surface along the principle axis, its image
View SolutionA concave spherical surface of radius of curvature 100 cm separates two media of refractive indices 1.50 and 43. An object is kept in the first medium at a distance of 30 cm from the surface. Calculate the position of the image.
View Solution
Knowledge Check
A convex refracting surface of radius of curvature 20cm separates two media of refractive indices 4/3and1.60. An object is placed in the first medium (μ=4/3) at a distance of 200cm from the refracting surface. Calculate the position of image formed.
A120cmB240cmC100cmD60cmA point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
A2h(μ2−1)1/2B2h(μ2−1)1/2Ch2(μ2−1)1/2Dh(μ2−1)1/2
A convex surface of radius of curvature 40 cm separate two media of refractive indices 43 and 1.50. An object is kept in the first medium at a distance of 20 cm from the surface. Calculate the position of the image.
View SolutionA concave spherical surface of radius of curvature 10cm separates two mediums X and Y of refractive indices 4/3 and 3/2 respectively. Centre of curvature of the surfaces lies in the medium X. An object is placed in medium X.
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
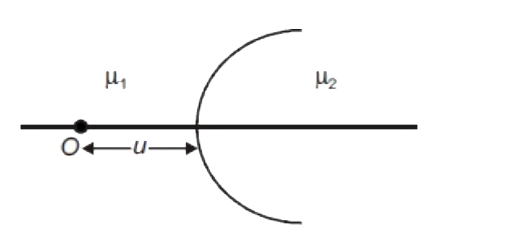
View SolutionThe diagram shows a spherical surface which separates two media of refractive index, μ1andμ2. Respectively. Now, a point object is placed on the principal axis as shown in the figure. Then
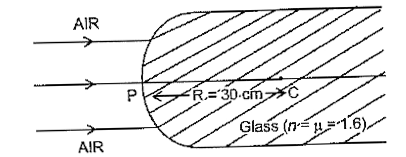
View SolutionFigure below shows a parallel beam of monochromatic light incident on a convex spherical surface, radius of curvature R = 30 cm, which separates glass (refractive index - 1 6) from air. Find the position of the image formed due to refraction of light at this single spherical surface.
View Solution
