A cricket fielder can throw the cricket ball with a speed $v_{0}$ . If he throws the ball while running with speed (u) at angle $θ$ to the horizontal, find
(b) what will be time of flight ?
(c ) what is the distance (horizontal range) form the point of projection at which the ball will land ?
(d) find $θ$ at which he should throw the ball that would maxmise the horizontal range range as found in (c ).
(e) how does $θ$ for maximum range change if $u > v_{0}, u = v_{0}, t - v_{0}$ ?
(f) how does $θ$ in (e) compare with that for u=0 (i.e., 45^@) ?

Video Solution

Text Solution

Verified by Experts

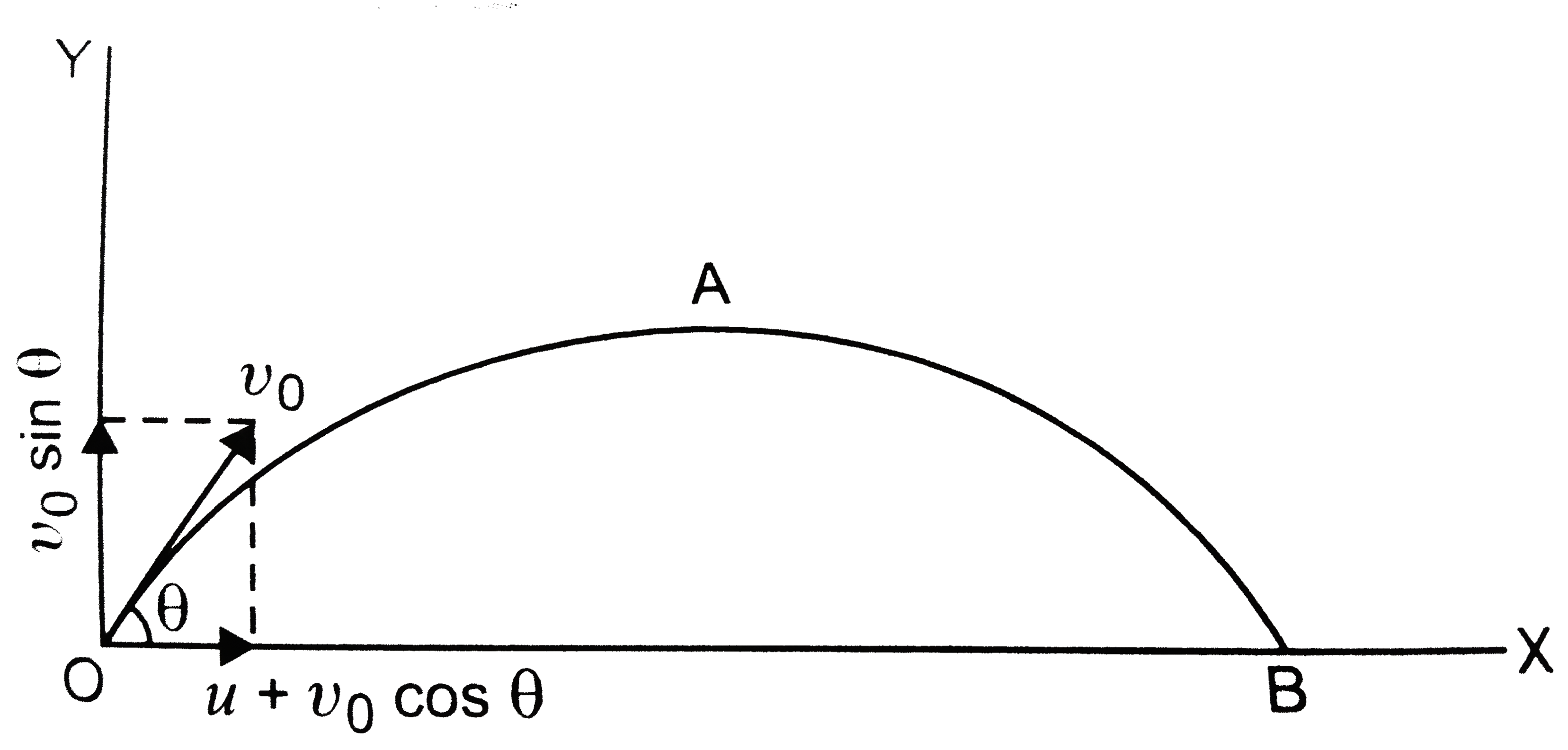
Refer Fig, 2 (EP) . 33 ,
(a) Here, at $O, u_{x} = u + v_{0} cos θ$ ltbRgt $u_{y} = v_{0} sin θ$
$tan θ = \frac{u_{y}}{u_{x}} = \frac{v_{0} sin θ}{u + v_{0} cos θ}$
or $θ = {tan}^{- 1} (\frac{v_{0} sin θ}{u + v_{0} cos θ})$
.
(b) Let (T) bne the time of flight of cricket ball from (O) to (B), then taking vertical moton of ball form (O) to (B), we have $y_{0}, = 0, u_{y} = v 0 sin θ, a_{y} = - g, t = T$
As, $y = y_{0} + u_{y} t + \frac{1}{2} a_{y} t^{2}$
:. $0 = 0 + v_{0} sin θ + \frac{1}{2} (- g) T^{2}$ or $T = \frac{2 v_{0} sin θ}{g}$
$(C) H or i z o n t a l r a n \geq,$ R= ( u + v_0 cos theta ) T = ( u + v_0 cos theta ) ( 2 v_0 sin theta)/g = v_0 /g [ 2 us sin theta + v_-0 sin 2 theta ] $< b r > (d) H or i z o n t a l r a n \geq w i l l b e max i μ if \frac{d T}{d θ} = 0$
:. $\frac{d R}{{(d θ)}_{=}} \frac{v_{0}}{g} [2 u cos θ + v_{0} cos 2 θ \times 2] = 0$
or $2 u cos θ + 2 v_{0} [2 {cos}^{2} θ - 1] = 0$ or $4 v_{0} {cos}^{2} θ + 2 u cos θ - 2 v_{0} = 0$ ltbr> or $o c s = ⎛ ⎜ ⎜ ⎝ - 2 u \pm \frac{\sqrt{4 U^{2} + 32 b_{0}^{2}}}{8 v_{0}} = (- u \pm \frac{\sqrt{u^{2} + 8 v - 0^{2}}}{4 v_{0}}$ or $θ_{max = {cos}^{- 1} [\frac{- u \pm {\sqrt{u}}^{2} + 8 v_{0}^{2}}{4 v_{0}}]}$
(e ) $(i) I (d$ u = v_0, ten cos theta = ( -v _0 +- sqrt ( v_0^2 + 8 v_0^2) /( 4 v_0) = (- 1 + 3)/4 = 1/2 cos 60^@ $or$ theta = 60^@ $< b r > (i i) I f$ $v_{0}$ , then $8 v_{0}^{2} + u^{2} \approx 8 v_{0}^{2}$
$θ_{max = {cos}^{- 1} [\frac{- u \pm 2 \sqrt{2} v_{0}}{4 v_{0}}] = {cos}^{- 1} [1 / (\sqrt{2}) - \frac{u}{4 v_{0}}]}$
If $u < < v_{0} t h e n$ theta _(max) = cos ^(-1) [ 1/(sqrt 2) ] = (pi)/4 $< b r > (i i) I f$ u gt v $and$ u gtgt v_0 $< b r >$ theta _max) = cos^(-1) [( 0 +- sqrt 8 v_0^2) /(4 v_0)] = cos^(-1) $(\frac{1}{\sqrt{2}}) = 45^{\circ}$ ltbr> (f) $I f$ u = 0, theta _(max) = cos ^(-1) [ (0+- sqrt 8 v_0^2)/( 4 v_90))] = cos ^(-1) (1/(sqrt 2) = 45 ^@.

Updated on:21/07/2023

A cricket fielder can throw the cricket ball with a speed $v_{0}$ .If the throws the ball while running with speed $u$ at an angle $θ$ to the horizontal.
The effective angle to the horizontal at which the ball is projected in air as seen by a spector is
A $θ = {tan}^{- 1} (\frac{v_{0} sin θ}{u + v_{0} cos θ})$
B $θ = {tan}^{- 1} (\frac{v_{0} cos θ}{u + v_{0} sin θ})$
C $θ = {cot}^{- 1} (\frac{v_{0} cos θ}{u + v_{0} sin θ})$
D $θ = {cot}^{- 1} (\frac{v_{0} cos θ}{u + v_{0} sin θ})$
A particle is projected with speed $u$ at angle $θ$ to the horizontal. Find the radius of curvature at highest point of its trajectory
A $\frac{u^{2} {cos}^{2} θ}{2 g}$
B $\frac{\sqrt{3} u^{2} {cos}^{2} θ}{2 g}$
C $\frac{u^{2} {cos}^{2} θ}{g}$
D $\frac{\sqrt{3} u^{2} {cos}^{2} θ}{g}$
A ball is projected from the ground at angle $θ$ with the horizontal. After $1 s$ , it is moving at angle $45^{\circ}$ with the horizontal and after $2 s$ it is moving horizontally. What is the velocity of projection of the ball ?
A $10 \sqrt{3} m s^{- 1}$
B $20 \sqrt{3} m s^{- 1}$
C $10 \sqrt{5} m s^{- 1}$
D $20 \sqrt{2} m s^{- 1}$