A cyclist rides along the circumference of a circular horizontal plane of radius $R$ , with the friction coefficient $μ = μ_{0} (1 - \frac{r}{R})$ , where $μ_{0}$ is constant and $r$ is distance from centre of plane $O$ . Find the radius of the circle along which the cyclist can ride with the maximum velocity, what is this valocity?

Video Solution

Text Solution

Verified by Experts

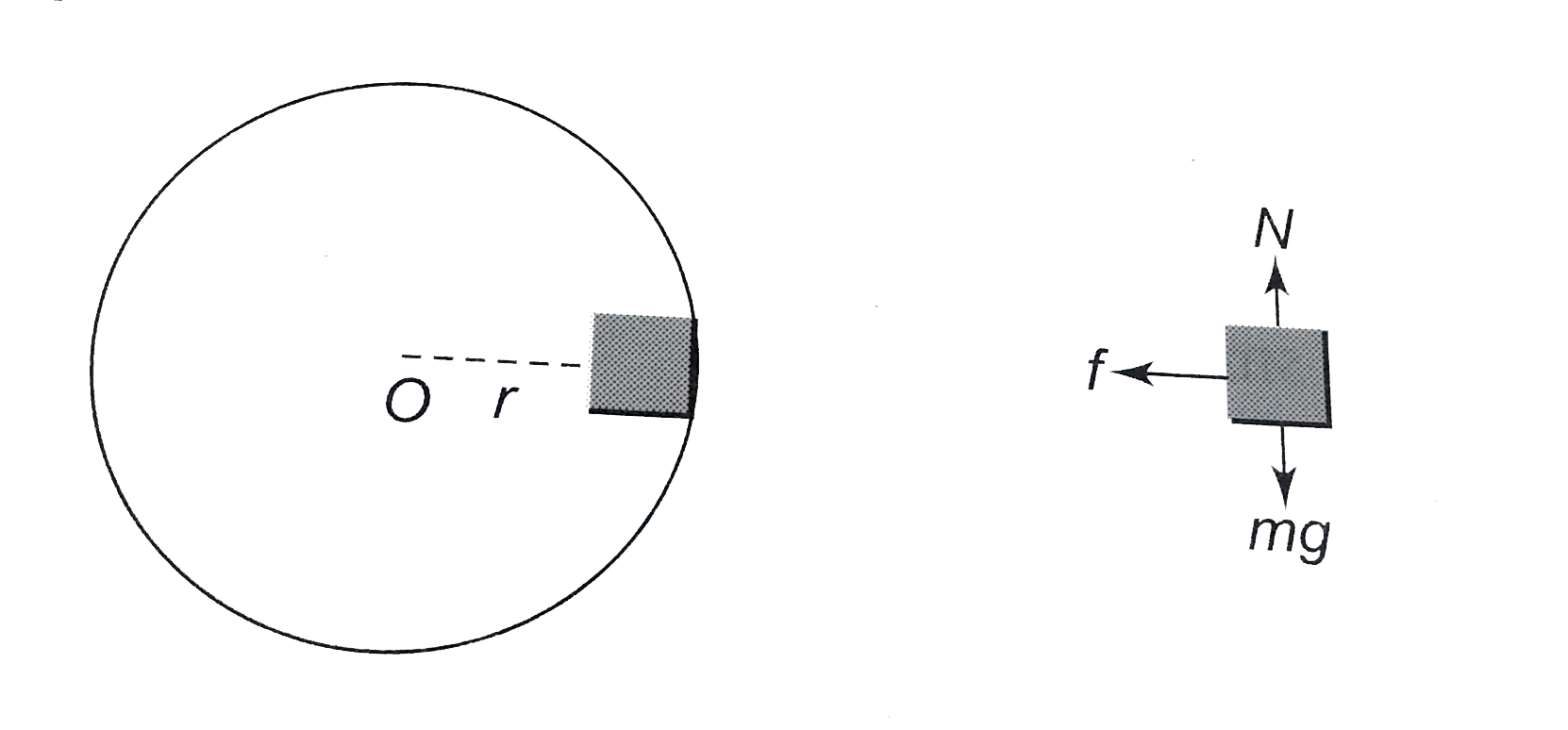
$f = \frac{m v^{2}}{r}$
$μ N = μ_{0} (1 - \frac{r}{R}) m g = \frac{m v^{2}}{r}$
$v^{2} = μ_{0} (r - \frac{r^{2}}{R}) g$
For $v$ to be maximum
$\frac{d v}{d r}$ or $\frac{d (v^{2})}{d r} = 0$
$μ_{0} g (1 - \frac{2 r}{R}) = 0 \Rightarrow r = \frac{R}{2}$
$v_{max} = \sqrt{μ_{0} [\frac{R}{2} - \frac{{(R / 2)}^{2}}{R}] g} = \frac{1}{2} \sqrt{μ_{0} g R}$

|

Updated on:21/07/2023

Class 11 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A cyclist rides along the circular path of a circular horizontal plane of radius R, the firctional coefficient being dependent only on distance r from the centre O of the plane as $μ = μ_{0} (1 - \frac{r}{R})$ , where $μ_{0}$ is a constant.
Find the radius of the circle such that the cyclist can ride with the maximum velocity.
AR
B $\frac{R}{2}$
C $\frac{R}{3}$
D $\frac{R}{4}$
Question 2 - Select One
A cyclist rides along the circular path of a circular horizontal plane of radius R, the firctional coefficient being dependent only on distance r from the centre O of the plane as $μ = μ_{0} (1 - \frac{r}{R})$ , where $μ_{0}$ is a constant.
What is the cyclist's maximum velocity ?
A $\sqrt{μ R g}$
B $2 \sqrt{μ_{0} R g}$
C $\frac{1}{2} \sqrt{μ_{0} R g}$
D $\sqrt{2 μ_{0} R g}$
Question 3 - Select One
The maximum speed of a car on a curved path of radius 'r' and the coefficient of friction $μ_{k}$ is
A $v = \sqrt{\frac{μ_{k}}{g r}}$
B $v = \sqrt{μ_{k} g r}$
C $v = \sqrt{\frac{g r}{μ_{k}}}$
D $v = \sqrt{\frac{1}{μ_{k} g r}}$

CP SINGH-CIRCULAR MOTION-Exercise

Home
Ask Doubt
Profile