
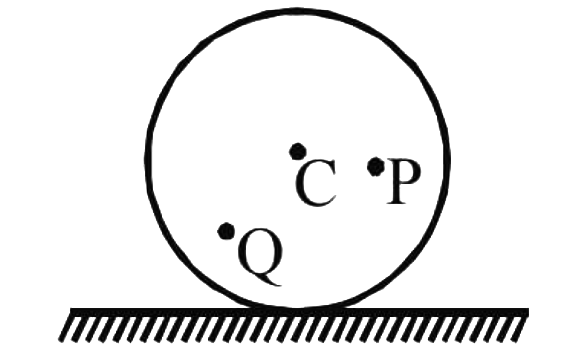
A disc is rolling (without slipping) on a horizontal surface. C is its centre and Q and P are two point equidistanec from C. let υp,υQ and υC be the magnitude of velocities of points P, Q, and C repsectively,
(a). υQ>υC>υP
(b). υQ<υC<υP
(c). υQ=υP,υC=12υP
(d). υQ<υC>υP
Video Solution
Text Solution

In case of pure rolling bottom most point in the instantaneous centre of zero velocity.
Velocity of any point on the disc, v=rω where r is the distance of point from O.
rQ>rC>rP⇒∴vQ>vC<Vp
Therefore, the correct option is (a).
|
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Examples
25 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples
2 Videos
- ROTATIONBOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
Knowledge Check
A disc is rolling (without slipping) on a horizontal surface. C is its center and Q and P are two points equidistant from C. Let VP,VQ and VC be the magnitude of velocities of points P,Q and C respectively, then
 .AVQ>VC>VPBVQ<VC<VPCVQ=VP,VC=12VPDVQ<VC>VP
.AVQ>VC>VPBVQ<VC<VPCVQ=VP,VC=12VPDVQ<VC>VPA disc is rolling without slipping on a horizontal surface with C, as its centre and Q and P the two points equidistant from C. Let vP,vQandvC be the magnitudes of velocities of point P,Q and C respectively, then
 AvQ>vC>vPBvQ<vC<vPCvQ=vP,vC=12vPDvQ<vC>vP
AvQ>vC>vPBvQ<vC<vPCvQ=vP,vC=12vPDvQ<vC>vPA disc is rolling without slipping with angular velocity ω. P and Q are two points equidistant from the centre C. the order of magnitude fo velocity is
 AvQ>vC>vPBvP>vC>vQCvP=vC,vQ=vC2DvP<vC>vQ
AvQ>vC>vPBvP>vC>vQCvP=vC,vQ=vC2DvP<vC>vQ
Sum of the first p, q and r terms of an A.P are a, b and c, respectively.Prove that ap(q−r)+bq(r−p)+cr(p−q)=0
View SolutionCheck the dimensional consistency of the relation υ=1I√Pρ where I is length, υ is velocity, P is pressure and ρ is density,
View SolutionIf the pth,qthandrth terms of an A.P. be a, b, c respectively, then
a(q−r)+b(r−p)+c(p−q)=
View SolutionThe molar specific heats of an ideal gas at constant pressure and volume are denotes by CP and Cυ respectively. If γ=CPCυ and R is the universal gas constant, then Cυ is equal to
View SolutionA resistor R,an inductor L, and a capacitor C are connected in series to an oscillator of freqency υ.if the resonant frequency is υr, then the current lags behind voltage, when:
View Solution
