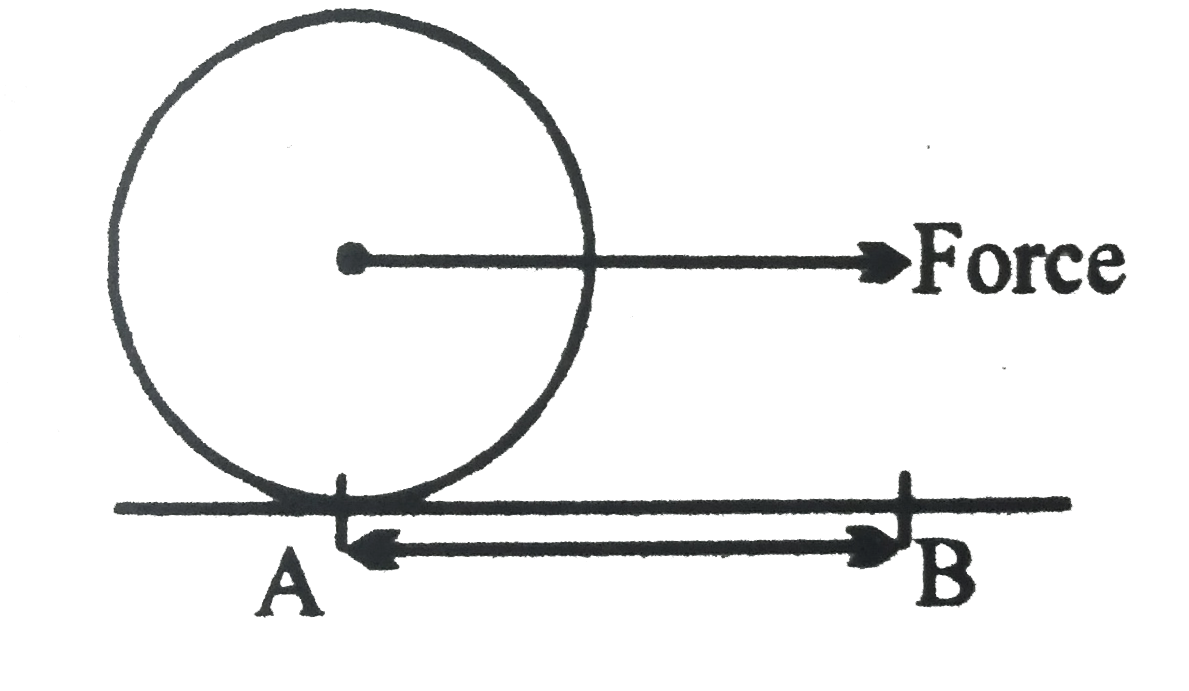
A disc of circumference s is at rest at a point A on a horizontal surface when a constant horizontal force begins to act on its centre. Between A and B there is sufficient friction toprevent slipping, and the surface is smooth to the right of B.AB=s. The disc moves from A to B in time T. To the right of B,
 .
.
the angular acceleration of the disc will disappear linear acceleration will remain unchanged
. linear acceleration of the disc will increase
the disc will make one rotation in time T/2
the disc will cover a distance greater than s in further time T
The correct Answer is:B, C, D
Let P=external force F= force of friction between A and B a1= acceleration between A and Ba2= acceleration beyond B
P−F=ma1
P=ma2
a2>a1
Let α=angualar acceleration between A and B
For one rotation θ=2π=12αT2
T=√4πα= time of travel from A to B
angular velocity at B=ω0=αT
For one rotation to the right of B
θ=2π=ωBt
t=2πaT=12αT2αT=T2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension71videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective19videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
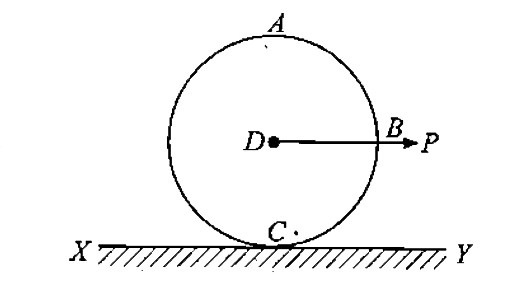
A disc of circumference S stands vertically on a horizontal surface as shown in figure-5.145, A horizontal force P acts on the centre of the disc. Half of the circumference {ABC) is rough and the friction is sufficient to prevent slipping when the disc rolls along X¥.The other half of the circumference (ADC) is smooth. The disc starts from rest when P begins to act and the point C is at the bottom. Find the distance moved by the disc along xy when it completes one rotation.
 View Solution
View SolutionA constant horizontal force F acts on a solid circular disc (M, R) as shown. The disc rolls without slipping, even on smooth surface for x=Rn . Value of n is?
View Solution
Knowledge Check
- Question 1 - Select One or More
A particle initially at rest on a smooth horizontal surface, is acted on by a constant horizontal force at time t=0 then if W= work, done t=time and v=speed of the particle, the nearly correct graph (s) is //are
A B
B C
C D
D
- Question 2 - Select One or More
A uniform disc is rolling on a horizontal surface. At a certain instant B is the point of contact and A is at height 2R from ground, where R is radius of disc -
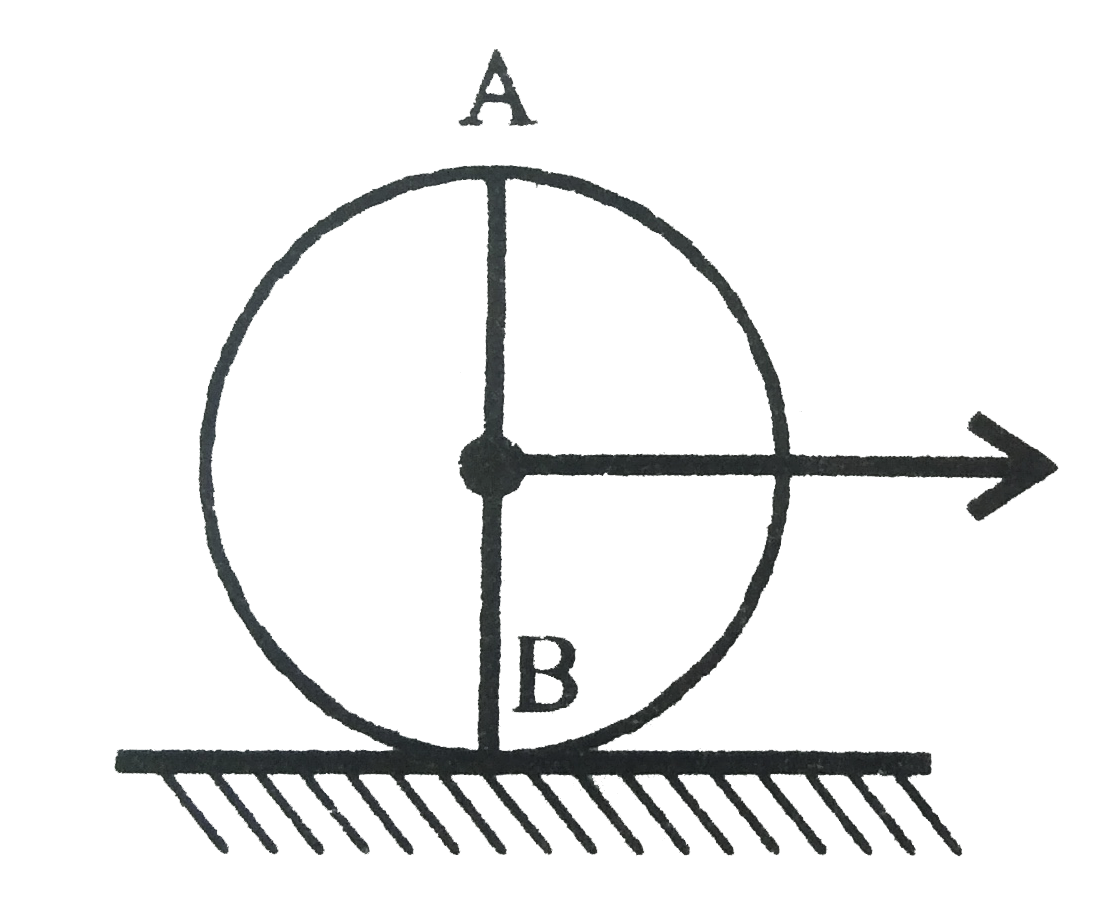 .AThe magnitude of the angular momentum of the disc about
.AThe magnitude of the angular momentum of the disc about
b is thrice that about ABThe angular momentum of the disc about A anticlockwiseCThe angular momentum of the disc about B is clockwiseDThe angular momentum of the disc about about A is equal to that about B. - Question 3 - Select One
A block 'B' rests on 'A' A rests on a horizontal surface 'C' which is frictionless. There is friction between A and B If 'B' pulled to the right .
AB moves forward and A to the leftB'B' only moves to the leftCB' does not moveD'A' and 'B' move together to the right .
A disc of mass 100 g is rolling without slipping on a horizontal surface with a velocity of 10cms−1. Calculate its total energy.
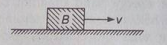
View SolutionA block B is pushed momentarily along a horizontal surface with an initial velocity v. If μ is the coefficient of sliding friction between B and the surface, block B will come to rest after a time
 View Solution
View SolutionA block B is pushed momentarily along a horizontal surface with an initial velocity v. If μ is the coefficient of sliding friction between B and the surface, block B will come to rest after a time
 View Solution
View SolutionA block B is pushed momentarily along a horizontal surface with an initial velocity v . If mu is the coefficient of sliding friction between B and the surface, block B will come to rest after a time:
 View Solution
View SolutionA disc of mass 2 kg is rolling on a horizontal surface without slipping with a velocity of 0.1 m/s. What is its rotational kinetic energy?
View Solution

