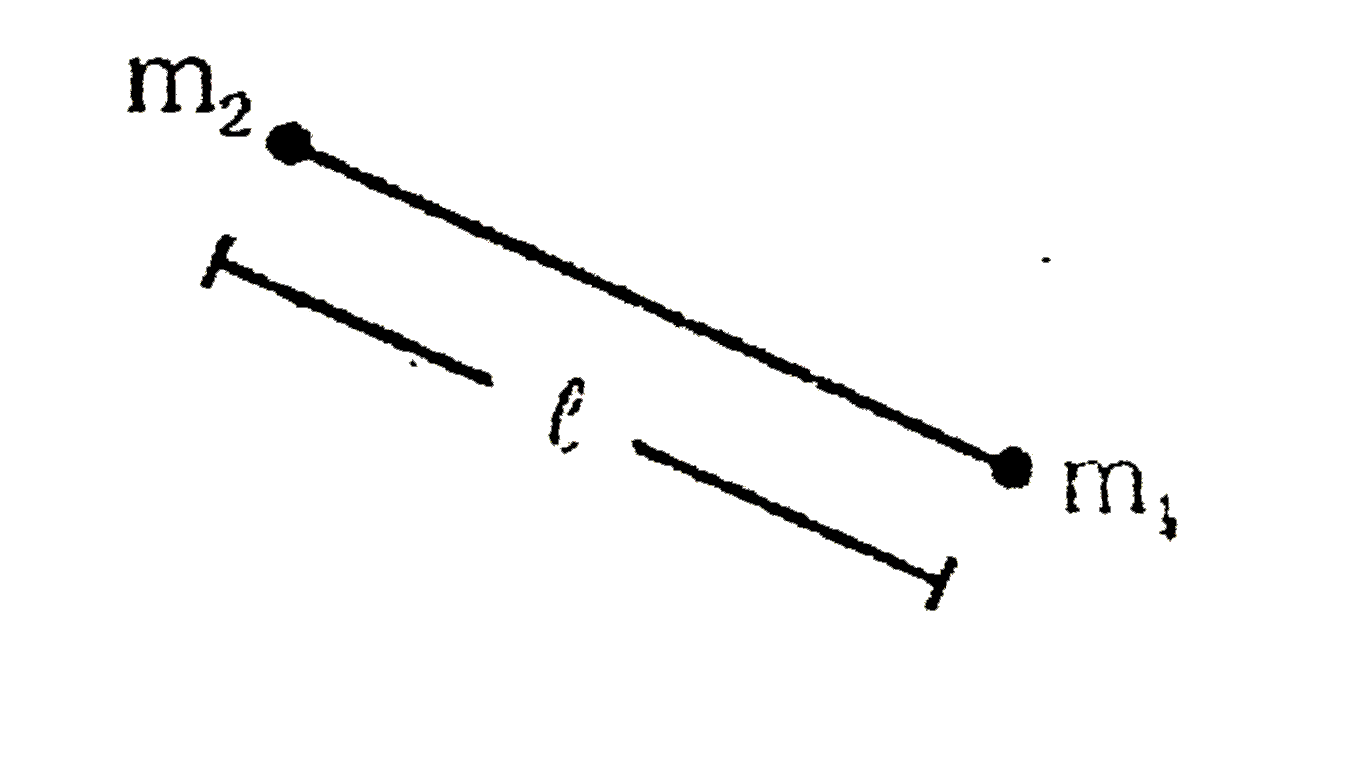
A drum major's baton consists of two masses m1 and m2 separated by a thin rod length l. The baton is thrown into the air. The problem is to find the baton's center of mass and the equation of motion for the center of mass.
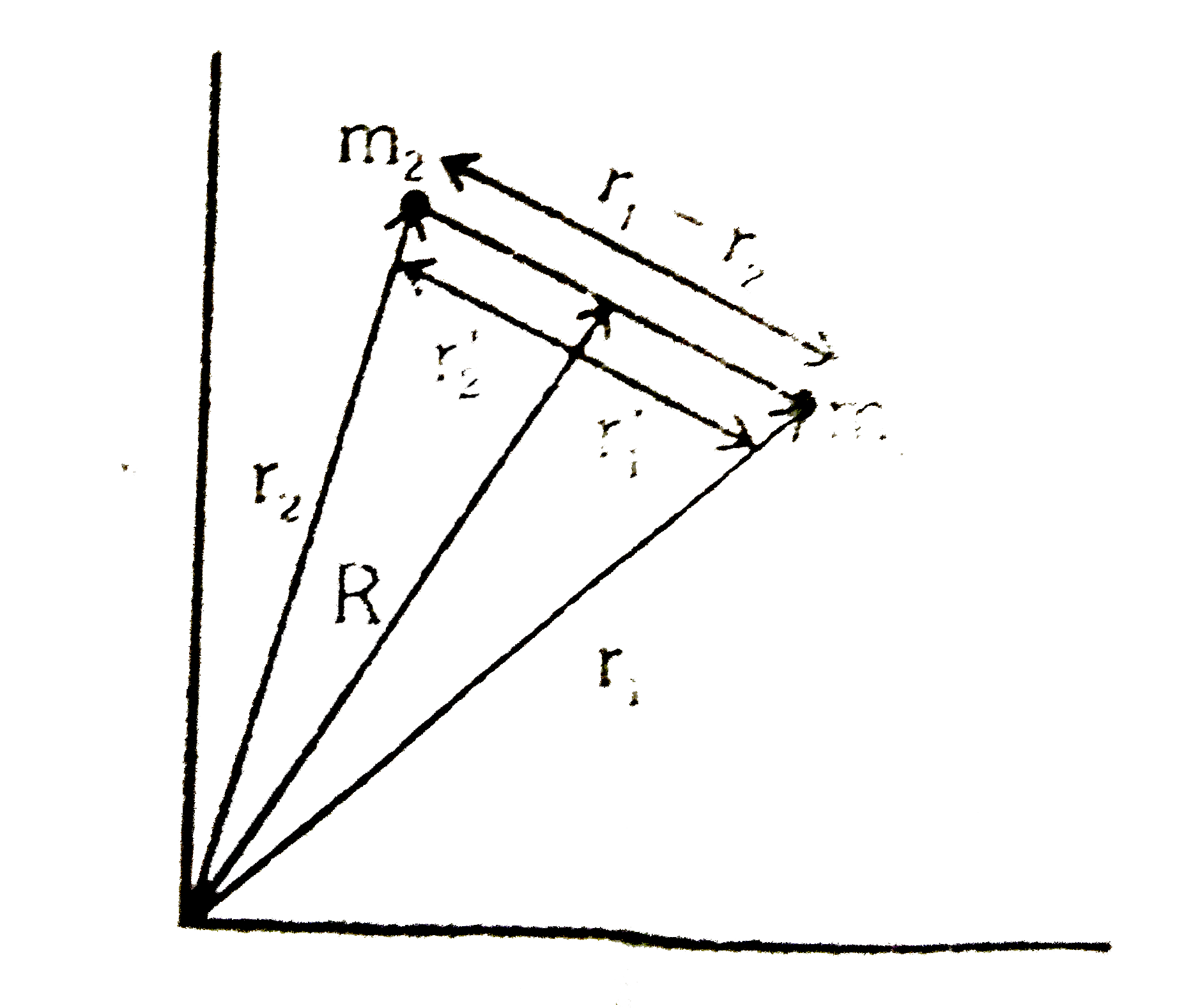
Let the position vectors of m1 and m2 be r1 and r2.
The position vector of the center of mass, measured from the same origin, is
R=m1r1+m2r2m1+m2
where we have neglected the mass of the thin rod. The center of mass lies on the line joining m1 and m2. To show this, suppose first that the tip of R dows not lie on the line, and consider the vectors r1r2 from the tip of R to m1 and m2
 From the sketch we see that r1=r1−R
From the sketch we see that r1=r1−R
r2=r2−R
Using equation (1) gives r1=r1−m1r1m1+m2−m2r2m1+m2=m2m1+m2(r1−r2)
r2=r2−m1r1m1+m2−m2r2m1+m2=−(m1m1+m2)(r1−r2)
r1 and r2 are propotional to r1−r2, the vector from m1 to m2. Hence r1 and r2 lie along the line jpining m1 and m2 as shown.
Furthermore, r1=m2m1+m2(r1−r2)=m2m1+m2
r2=m1m1+m2(r1−r2)=m1m1+m2l
Assuming that friction is negligible, the external force on the baton is F=m1g+m2g
The equation of motion of the center of mass is (m1+m2)R=(m1+m2)g or R=g
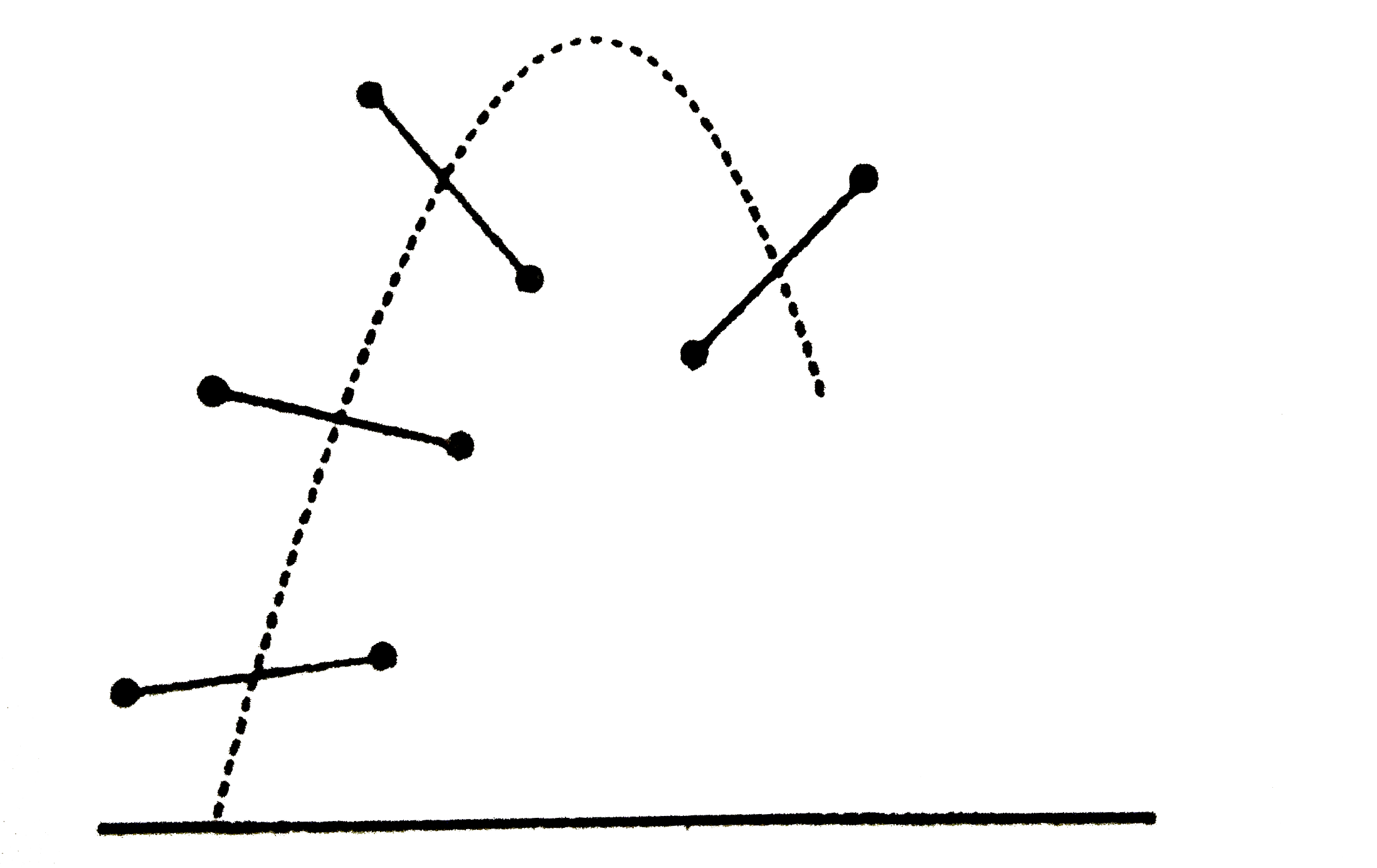 The center of mass follows the parabolic trajectory of single mass in a uniform gravitational field.
The center of mass follows the parabolic trajectory of single mass in a uniform gravitational field.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
Two point masses m1 and m2 are joined by a weightless rod of length r. Calculate the moment of inerrtia of the system about an axis passing through its centre of mass and perpendicular to the rod.
View SolutionTwo particles of masses m1 and m2 (m1>m2) are separated by a distance d. The shift in the centre of mass when the two particles are interchanged.
View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O

View SolutionTwo point masses m and 4m are connected by a light rigid rod of length l at the opposite ends. Moment of inertia of the system about an axis perpendicular to the length and passing through the centre of mass is
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View SolutionTwo particles of mass m1 and m2 are approaching towards each other under their mutual gravitational field. If the speed of the particle is v, the speed of the center of mass of the system is equal to :
View SolutionTwo particles of masses m1andm2 are connected to a string and the system is rotated in a horizontal plane with 'P' as center. The ratio of tension in the two parts of string is

View SolutionThe linear density of a thin rod of length 1m lies as λ=(1+2x), where x is the distance from its one end. Find the distance of its center of mass from this end.
View SolutionA thin of length L is bent to form a semicircle. The mass of rod is M. What will be the gravitational potential at the centre of the circle ?
View SolutionIn a double star system one of mass m1 and another of mass m2 with a separation d rotate about their common centre of mass. Then rate of sweeps of area of star of mass m1 to star of mass m2 about their common centre of mass is
View Solution
