A : For interference fringes to be seen, the condition sS<λd should be satisfied. Where .s. be the size of source slit, S is its distance from plane of two slits and .d. is the distance between two slits.
R : In Y.D.S.E, if distance of source slit from the two slits (s) decreases, the interference pattern gets more sharp.
Both A and R are true and R is the correct explanation of A
Both A and R are true and R is not the correct explanation of A
A is true and R is false
Both A and R are false
The correct Answer is:C
To solve the given problem, we need to analyze the two statements provided: Assertion (A) and Reason (R).
Step 1: Analyze the Assertion (A)
The assertion states that for interference fringes to be seen, the condition sS<λd must be satisfied, where:
- s is the size of the source slit,
- S is the distance from the source slit to the plane of the two slits,
- d is the distance between the two slits,
- λ is the wavelength of the light used.
This condition implies that the size of the source slit relative to its distance from the slits must be small compared to the wavelength divided by the distance between the slits. If this condition is not satisfied, the interference pattern will not be clearly observable.
Conclusion for Assertion: The assertion is True.
Step 2: Analyze the Reason (R)
The reason states that in Young's Double Slit Experiment (Y.D.S.E), if the distance of the source slit from the two slits (S) decreases, the interference pattern gets sharper.
To analyze this, we note that if S decreases, the ratio sS increases (since s remains constant). If sS increases, it may violate the condition sS<λd. This means that the interference pattern may become less distinct or even disappear if the condition is not satisfied.
Conclusion for Reason: The reason is False because decreasing S does not lead to a sharper interference pattern; instead, it can lead to a failure to observe the interference pattern altogether.
Final Conclusion
- Assertion (A) is True.
- Reason (R) is False.
Thus, the correct option is that the assertion is true, but the reason is false.
The assertion states that for interference fringes to be seen, the condition sS<λd must be satisfied, where:
- s is the size of the source slit,
- S is the distance from the source slit to the plane of the two slits,
- d is the distance between the two slits,
- λ is the wavelength of the light used.
The reason states that in Young's Double Slit Experiment (Y.D.S.E), if the distance of the source slit from the two slits (S) decreases, the interference pattern gets sharper.
- Assertion (A) is True.
- Reason (R) is False.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (INTERFERENCE)26videos
WAVES OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (DIFFRACTION)17videos
WAVES OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -IA (ASCENDING AND DSCENDING ORDER TYPE QUESTIONS)6videos
WAVES OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)15videos
WAVES
Similar Questions
In Young's double slit experiment, if the two slits are illuminated with separate sources, no interference pattern is observed because
View SolutionA : Y.D.S.E, as the source slit width increases, fringe pattern gets less and less sharp.
R : When the source slit is so wide that the condition sS<λd is not satisfied, the interference pattern is appears.View Solution
Knowledge Check
- Question 1 - Select One
In a double slit experiment, the distance between the slits is d. The screen is at a distance D from the slits. If a bright fringe is formed opposite to one of the slits, its order is
AdλBλ2dDCD22λDDd22Dλ - Question 2 - Select One
Consider a two slit interference arrangements (figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
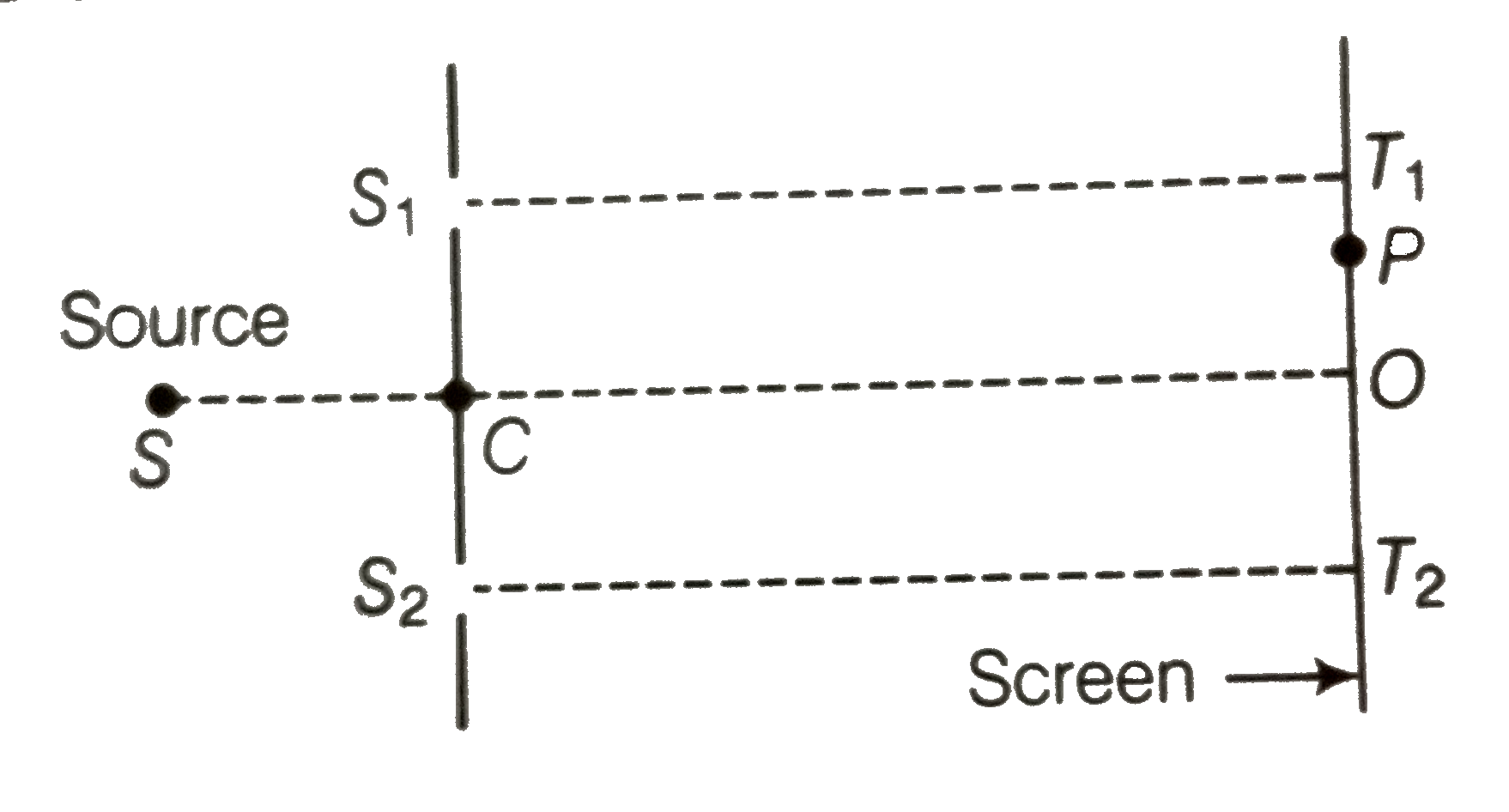 Aλ2.472Bλ2.236Cλ1.227Dλ3.412
Aλ2.472Bλ2.236Cλ1.227Dλ3.412
In a Young’s double slit experiment, the separation of the two slits is doubled. To keep the same spacing of fringes, the distance D of the screen from the slits should be made
View SolutionIn a Young’s double slit experiment, the source illuminating the slits is changed from blue to violet. The width of the fringes
View SolutionIn Young's double slit experiment, the distance between two slits is made three times then the fringe width will becomes
View SolutionAnswer the following:
(a) what are coherent sources of light ? Two slits in Young's double slit experiments are illuminated by two different sodium lamps emitting light of the same wavelength. Why is no interference pattern observed?
(b) Obtain the condition for getting dark and bright fringes in Young's experiment. Hence write the expression for the fringe width.
(c) If s is the size of the source and its distance from the plane of the two slits, what should be the criterion for the interference fringes to be seen?View SolutionConsider a two slit interference arrangements (figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
 View Solution
View SolutionIn young's double slit experiment, distance between the slit S1andS2 is d and the distance between slit and screen is D . Then longest wavelength that will be missing on the screen in front of S1 is
View Solution

