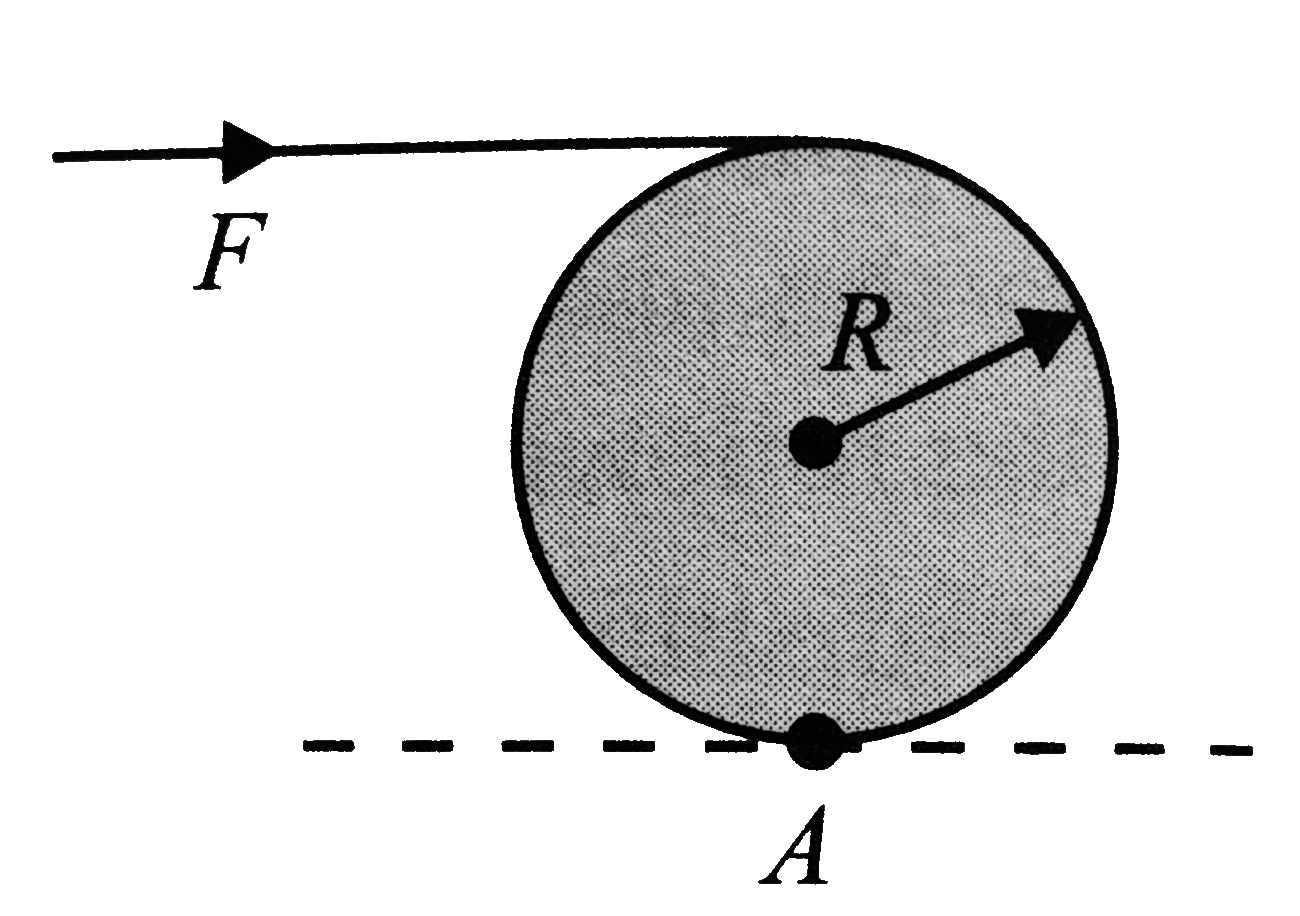
A force of magnitude F is acting on rolling body of mass m and radius R as show in figure. What happens when it enters on a smooth surface?

When the body is rolling on the rough surface, the friction force acting on the surface acts in the backward direction. The force F increases acceleration of centre of mass while friction force provides torque for providing angular acceleration. The velocity of centre of mass is always such that vc=ωR, i.e, rolling is present. when the body enters on the smooth surface, the pulling force F is still acting i.e., F≠0

When the rolling body enters on a smooth surface, the force of friction disappears, i.e, f=0. As a result angular acceleration of the body about its centre of mass becomes zero.
What ever is the angular speed attained by the body, it continues to rotate with that. On the conntrary, the linear acceleration of the centre of mass suddenly increases to Fm
As a result its linear speed starts increasing and the condition vc=ωR does not hold true and the body will not roll on the smooth surface. The point of contact slips forward (v>ω0R).
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples
12 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.1
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A force of magnitude F is acting on a rolling body of mass m and radius R as shown in figure. What happens if the pulling force F is removed ? Discuss the different posibilities when the pulling acts on the body.

a. At centre, b. Above centre, c. Below centre
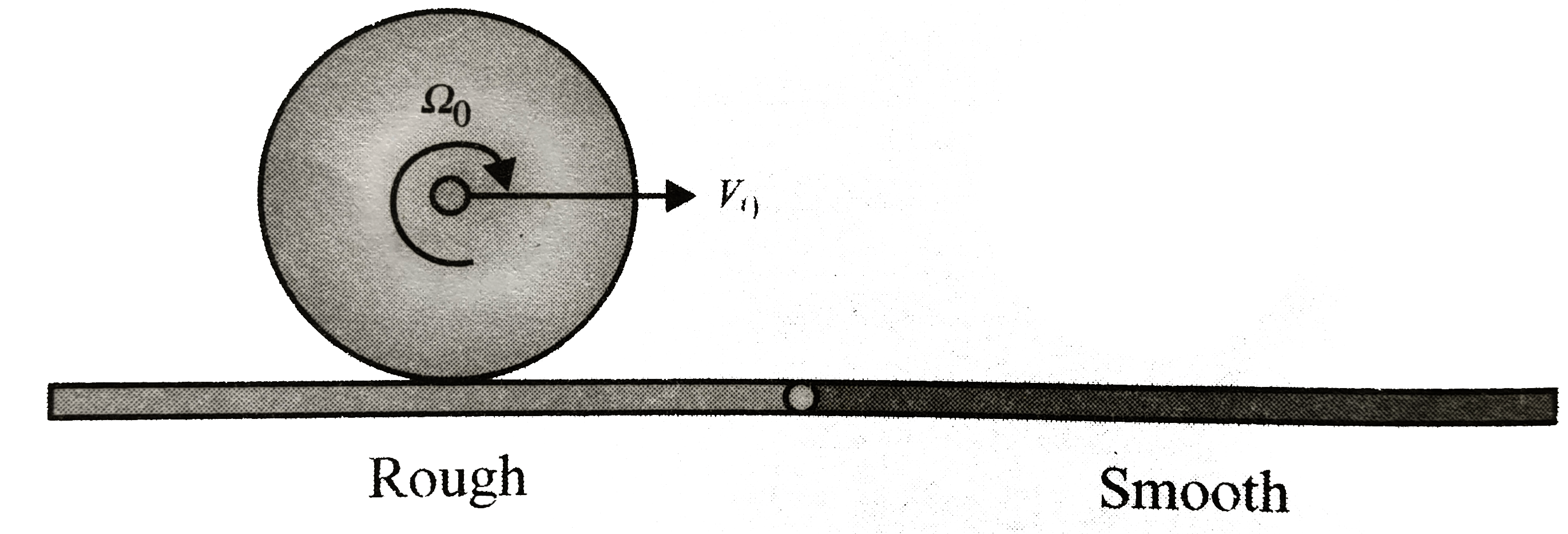
View SolutionA rolling body of mass m and radius R is rolling on a rough surface without any pulling force as shown in figure. What happens when it enters on a smooth surface?
View SolutionThree forces of magnitude F, F and √2F are acting on the periphery of a disc of mass m and radius R as shown in the figure. The net torque about the centre of the disc is
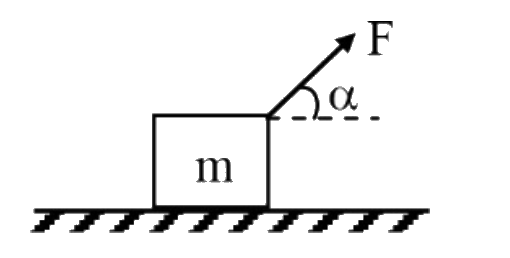
View SolutionAt the instant t=0, a force F=kt (k is a constant) acts on a small body of mass m resting on a smooth horizontal surface. The time, when the body leaves the surface is
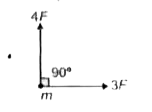
View SolutionWhen a force F acts on a particle of mass m, the acceleration of particle becomes a. now if two forces of magnitude 3F and 4F acts on the particle simultaneously as shown in figure, then the acceleration of the particle is
View SolutionWhen a rolling body enters onto a smooth horizontal surface, it will
View SolutionA force F is applied at the top of a ring of mass M and radius R placed on a rough horizontal surface as shown in figure. Friction is sufficient to prevent slipping. The friction force acting on the ring is:

View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
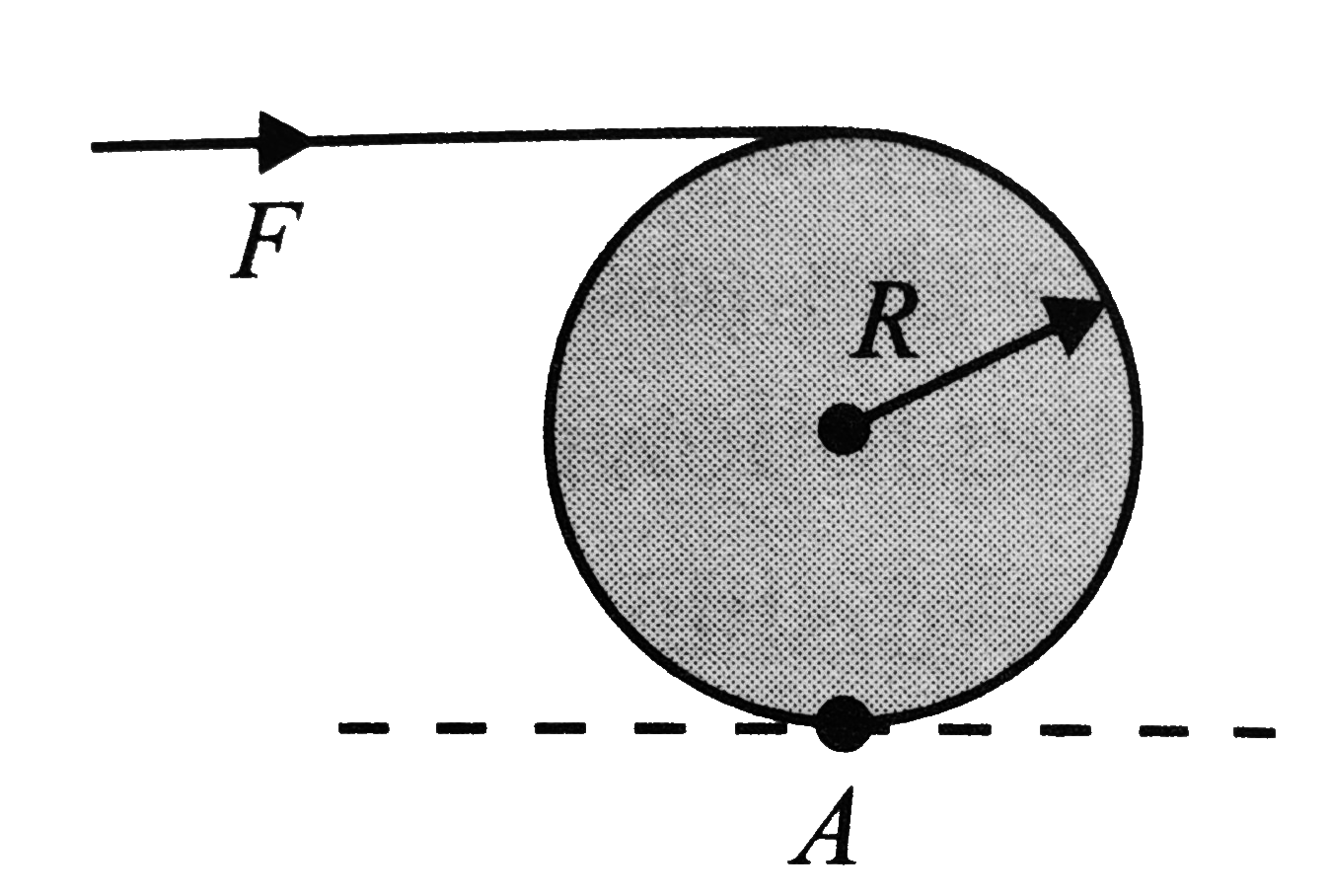
Tangential acceleration of the centre of mass is
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
Component of reaction at the hinge in the horizontal direction is
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
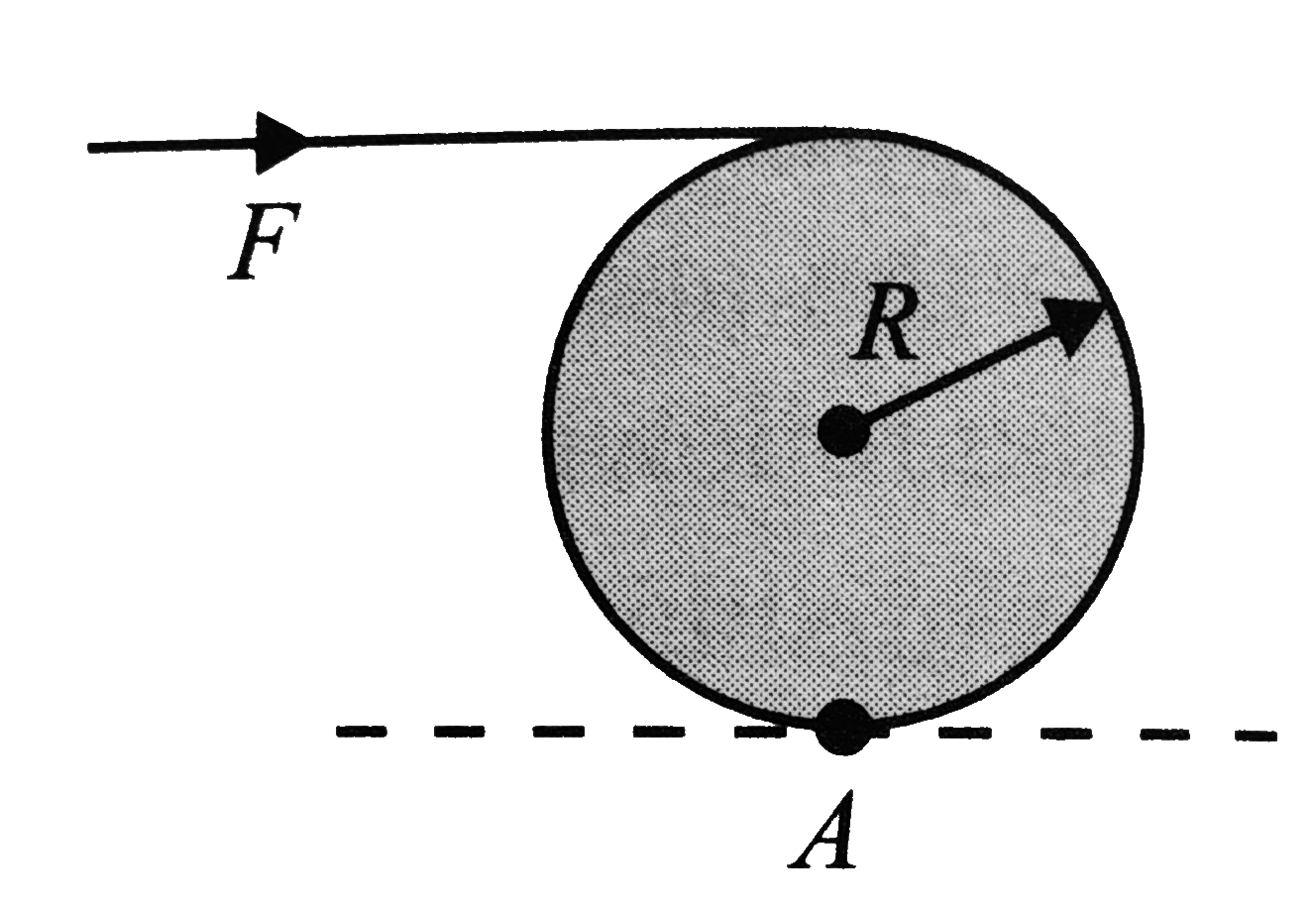
Component of reaction at the hinge in the vertical direction is
View Solution
