A force $\vec{F} = 2 x \hat{j}$ newton acts in a region where a particle moves annticlockwise in a square loop of 2m in x $-$ y plane as shown in figure . Calculate the total amount of work done. Is this force a conservative force or a non-conservative force?

Video Solution

Text Solution

Verified by Experts

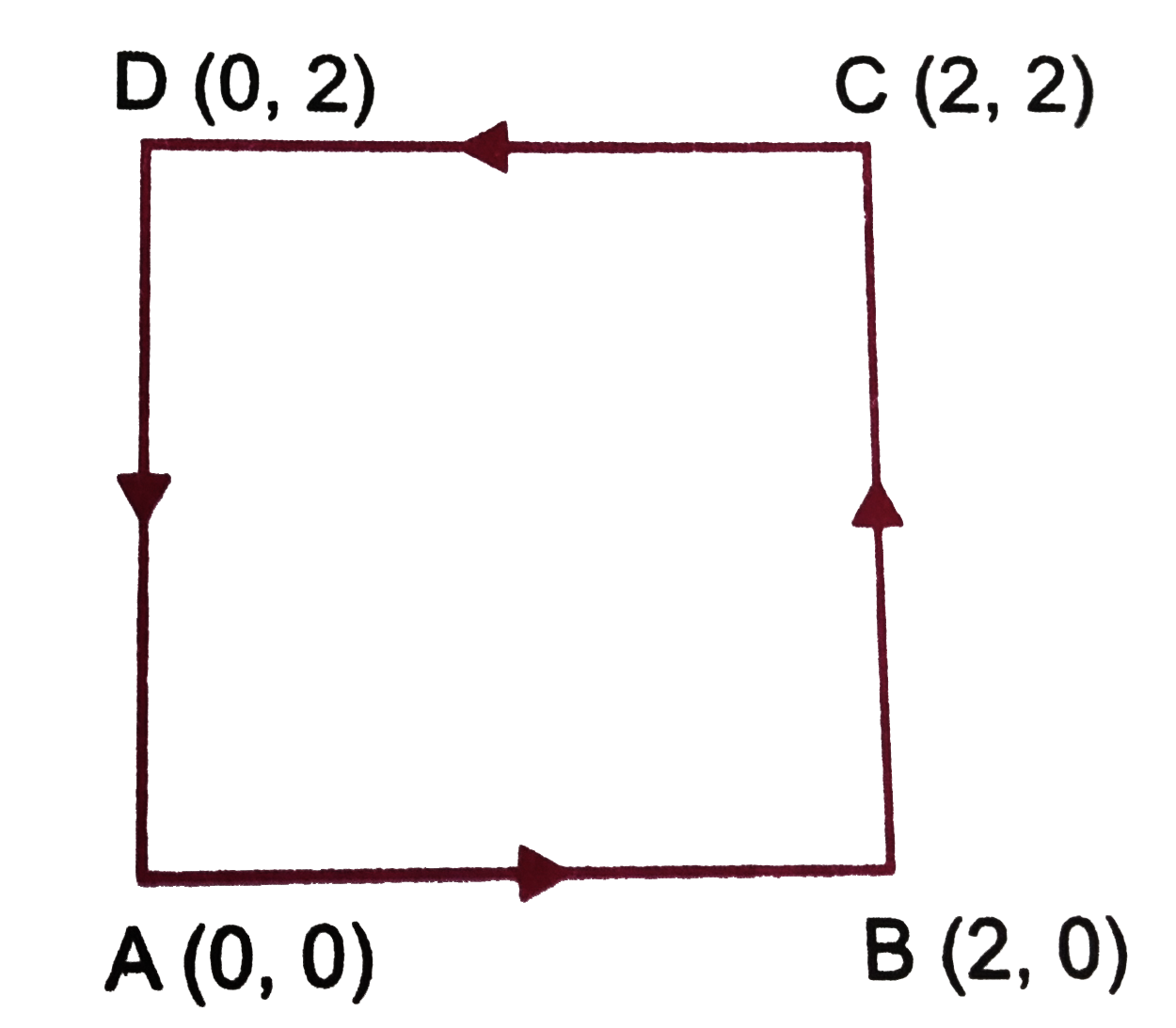
Here, $\vec{F} = 2 x \hat{j}$ newton
Total work done in moving the particle
$W = \int_{A B C D A} \vec{F} . d \vec{x}$
$= \int_{0}^{2} (2 x \hat{j}) . (d x) i + \int_{0}^{2} (2 x \hat{j}) . (d x) \hat{j}$
$+ \int_{2}^{0} (2 x \hat{j}) . d x (- \hat{i}) + \int_{2}^{0} (2 x \hat{j}) . d x (- \hat{j})$
$= 0 + 2 {[\frac{x^{2}}{2}]}_{0}^{2} + 0 - 2 {[\frac{x^{2}}{2}]}_{0}^{2}$
$= 4 - (0 - 4) = 8 J$
As work done by the force around a round trip is not zero, therefore, the force must be non-conservative.

Updated on:21/07/2023