a. Given n resistors each of resistance R. How will you combine them to get the (1) maximum (ii) minimum effective resistance? What is the ratio of the maximum to minimum effective resistance?
b. Given the resistances of 1Ω,2Ω,3Ω.. How will you combine them to get an equivalent resistance of (i) (11/3) Ω(ii) (11/5)Ω, (iii) 6Ω, (iv) (6/11) Ω?
c. Determine the equivalent resistance of networks shown in the figure. 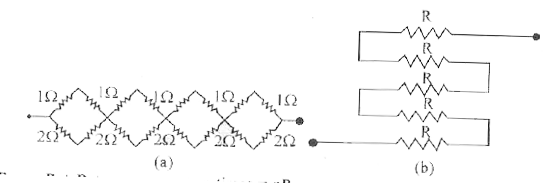
Rmax=R+R+…………….ntimes=nR
1Rmin=1R+1R+…………..ntimes=nR ∴Rmax=Rn
RmaxRmin=nRnR=n2
b.R1Ω,R2=2Ω,R3Ω
I(R1||R2)series withR3
R=R1R2R1+R2+R3=1×21+2+3=113Ω
(ii)(R2|||R2) series with R1 R=R2R3R2+R3+R1=2×32+3+1=115Ω
(iii) R=R1+R2+R3=1+2+3=6Ω
(iv) 1R=1R1+1R2+1R3=11+12+13=6+3+26=116 ∴R=611Ω
c. The networks takes the from
(i) R=(1+2)||(2+2)+(1+1)||(2+2)+(1+1)||(2+2)=(1+1)||(2+2)
=(2×42×4)×4=86×4=163Ω
(ii) Being series R=R+R+R+R+R=5R
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - NEW JOYTHI PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE PROBLEMS FOR SELF ASSESSMENT
25 Videos
- CURRENT ELECTRICITYBOOK - NEW JOYTHI PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - EVALUATION QUESTIONS AND ANSWERS
72 Videos
- CURRENT ELECTRICITYBOOK - NEW JOYTHI PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - COMPETITIVE EXAM CORNER
37 Videos
- ATOMSBOOK - NEW JOYTHI PUBLICATIONCHAPTER - ATOMSEXERCISE - COMPETITIVE EXAM CORNER
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NEW JOYTHI PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - COMPETITIVE EXAM CORNER
15 Videos
Similar Questions
(a) Given n resistors each of resistance R. How will you combine them to get the (i) maximum (ii) minimum effective resistance ? What is the ratio of the maximum to minimum resistance ?
(b) Given the resistances of 1Ω,2Ω,3Ω, how will you combine them to get an equivalent resistance of (i) (11/3)Ω (ii) (11/5)Ω (iii) 6Ω (iv) (6/11)Ω ?
(c) Determine the equivalent resistance of networks shown in Figure.
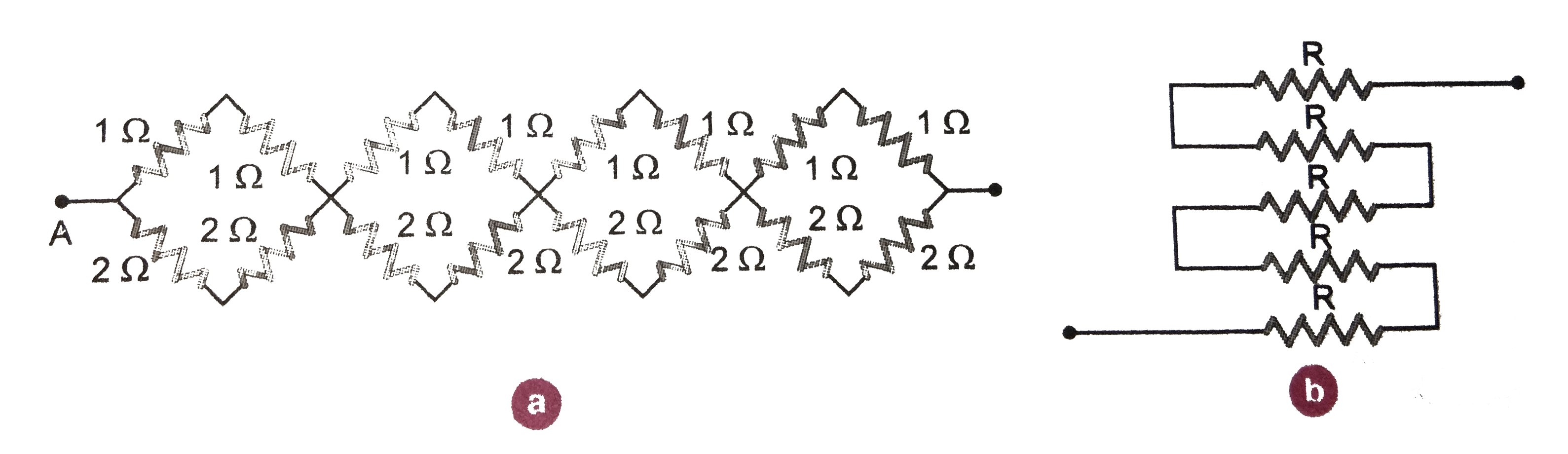
View Solution(a) Given n resistors each of resistance R. How will you combine them to get the (i) maximum (ii) minimum effective resistance ? What is the ratio of the maximum to minimum resistance ?
(b) Given the resistances of 1Ω,2Ω,3Ω, how will you combine them to get an equivalent resistance of (i) (11/3)Ω (ii) (11/5)Ω (iii) 6Ω (iv) (6/11)Ω ?
(c) Determine the equivalent resistance of networks shown in Figure.
View Solution
Knowledge Check
If you are provided three resistance 2Ω,3Ω and 6Ω. How will you connect them so as to obtain the equivalent resistance of 4Ω
A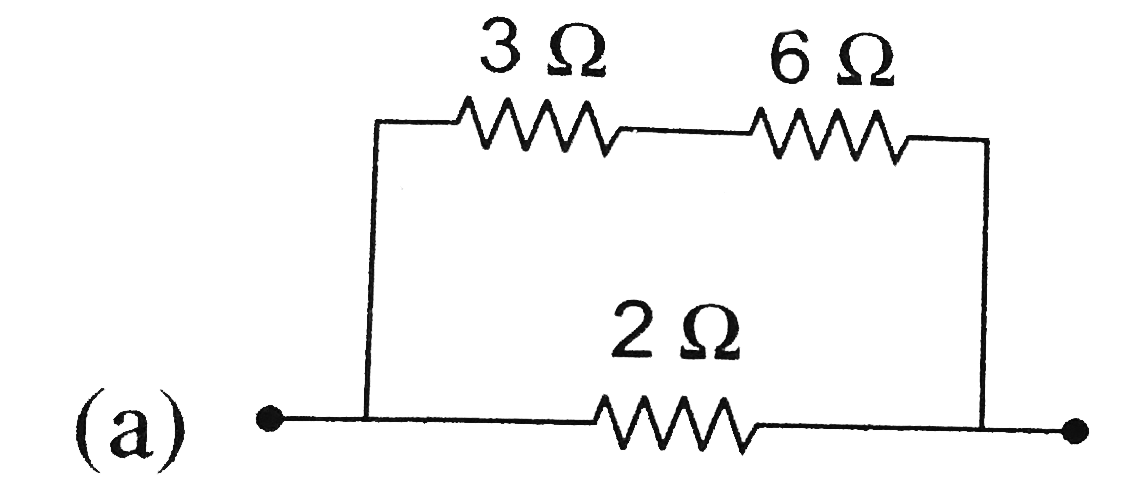 B
B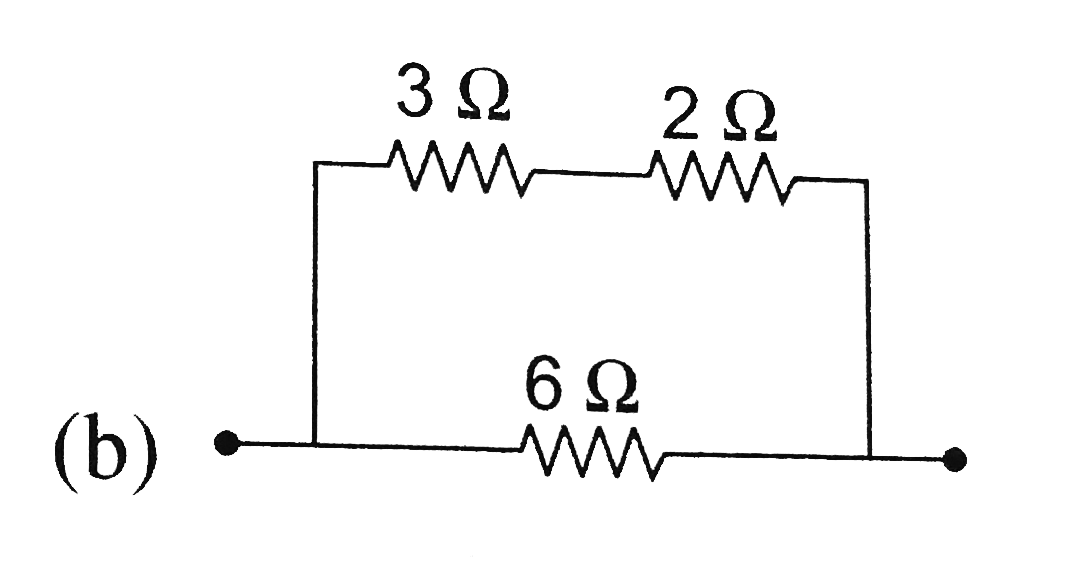 C
C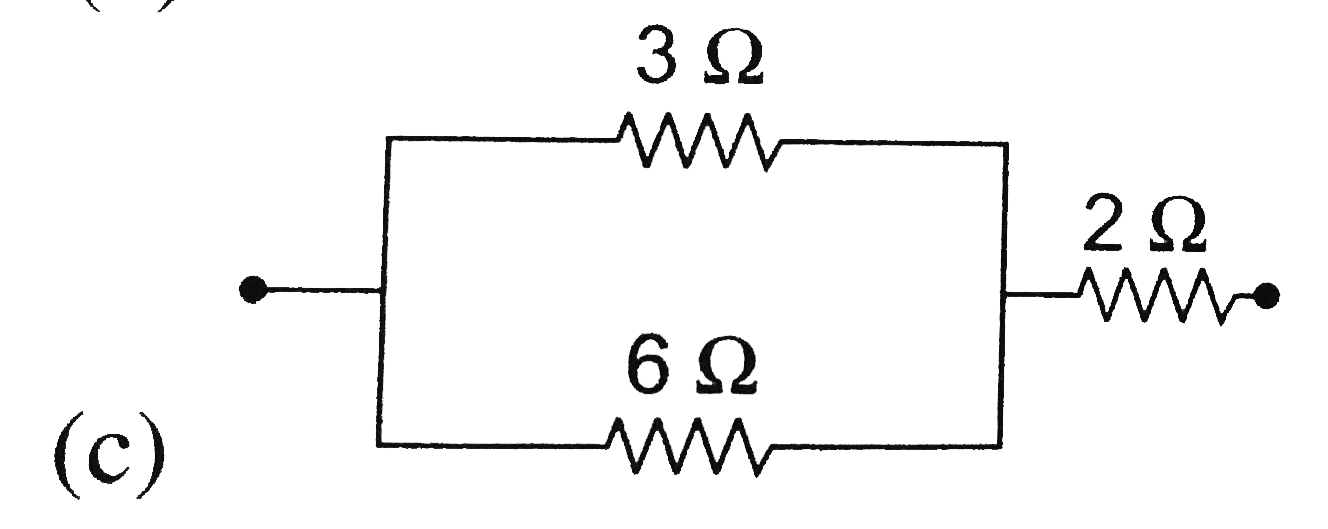 Dnone
Dnone
Given n resistors each of resistance R, how will you combine them to get the (i) maximum (ii) minimum effective resistance ? What is the ratio of the maximum to minimum resistance ?
View SolutionGiven n resistors each of resistance R, how will you combine them to get the (i) maximum, and (ii) minimum effective resistance? What is the ratio of the maximum to minimum resistance?
View SolutionGiven n resistors each of resistane R, how will you combine them to get the (i) maximum, (ii) minimum effective resistance ? What is the ratio of the maximum to minimum resistance ?
View SolutionGiven the resistances of 1Ω,2Ω,3Ω how will we combine them to get an equivalent resistance of (i) (113)Ω,(ii)(115)Ω,(iii)6Ω,(iv)(611)Ω ?
View SolutionGiven the resistances of 1Ω,2Ωand3Ω. How will you combine them to get an equivalent resistance of
(i) 113Ω ?
(ii) 115Ω ?
View SolutionGiven the resistance of 1Ω,2Ω,3Ω, how will you combine them to get an e3quivalent resistance of :
113Ω (ii)115Ω (iii)6Ω 611Ω ?
View SolutionGiven three resistors of resistances 1Ω,2Ωand3Ω. How will you combine them to get an equivalent resistance of 115Ω ?
View Solution
