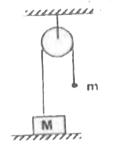
A heavy mass M resting on the ground is attached to a small mass m via massless inextensible string passing over a pulley.The string connected to M is loose.The smaller mass falls freely through a height h and the string becomes tight. Obtain the time from this instant when the heavier mass again makes contact with the ground. Also obtain the loss in K.E. when M is jerked in to motion.
The correct Answer is:[2m(M−m)g√2hg,MmghM+m]
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Advance MCQs
17 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.3
3 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
Similar Questions
A heavy mass M resting on the ground is connected to a lighter mass m through a light inextensible string passing over an ideal pulley. The string connected to mass M is loose. Let lighter mass m be allowed to fall freely through a height h such that the string becomes taut. If t is the time from this instant onward when the heavier mass again makes contact with the ground and ΔE is change in kinetic energy then
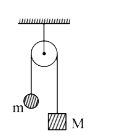
View SolutionA mass m rests on a horizontal table. It is attached to a light inextensible string which passes over a smooth pulley and carries a mass m at the other end. If the mass m is raised vertically through a distance h and is then dropped, what is the speed with which the mass 2m begins to rise?

View Solution
Knowledge Check
A heavy mass M resting on the ground is connected to a lighter mass m through a light inextensible string passing over an ideal pulley. The string connected to mass M is loose. Let lighter mass m be allowed to fall freely through a height h such that the string becomes taut. If t is the time from this instant onward when the heavier mass again makes contact with the ground and ΔE is change in kinetic energy then
 At=2mM+m√2hgBt=2mM−m√2hgCΔE=−(Mmm+m)ghDΔE=−Mgh
At=2mM+m√2hgBt=2mM−m√2hgCΔE=−(Mmm+m)ghDΔE=−MghTwo masses ,each equal to m ,are arrached to one another by a massless string passing over a smooth pulley the tension in the string is :
AmgB2mgCmg/2DzeroTwo masses M and m(M>m) are joined by a light string passing over a smooth light pulley.
 AThe acceleration of each block is (M−mM+m)gBThe tension in the string is 2MmgM+mCThe centre of mass of the 'M plus m' system moves down with an acceleration of g(M−mM+m)2DThe tension in the string by which the pulley is attached to the rodd is (M+m)g
AThe acceleration of each block is (M−mM+m)gBThe tension in the string is 2MmgM+mCThe centre of mass of the 'M plus m' system moves down with an acceleration of g(M−mM+m)2DThe tension in the string by which the pulley is attached to the rodd is (M+m)g
A mass m rests on a horizontal table. It is attached to a light inextensible string which passes over a smooth pulley and carries a mass m at the other end. If the mass m is raised vertically through a distance h and is then dropped, what is the speed with which the mass 2m begins to rise?

View SolutionIn the figure shown, a particle of small mass m is joined to a very heavy mass M (M >> m) by a light string passing over a light pulley. The total downward force on the pulley by the string is :
View SolutionTwo masses M and M/ 2 are joint together by means of a light inextensible string passes over a frictionless pulley as shown in figure. When bigger mass is released the small one will ascend with an acceleration of
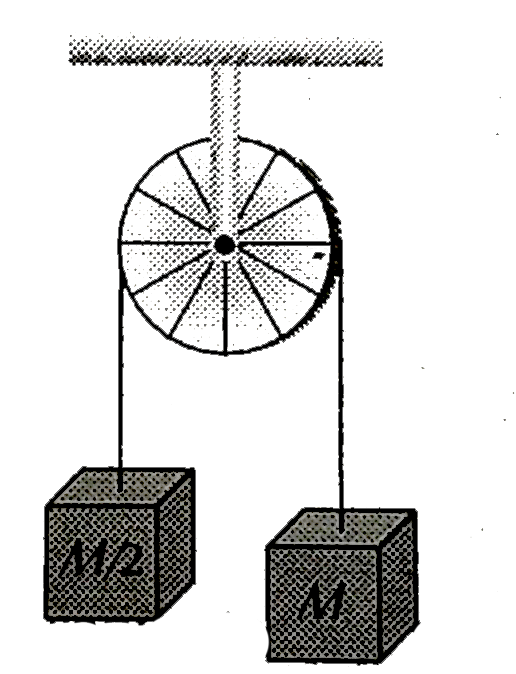
View SolutionIn the figure shown, a particle of small mass m is joined to a very heavy mass M (M >> m) by a light string passing over a light pulley. The total downward force on the pulley by the string is :

View SolutionTwo masses M and M/ 2 are joint together by means of a light inextensible string passes over a frictionless pulley as shown in figure. When bigger mass is released the small one will ascend with an acceleration of
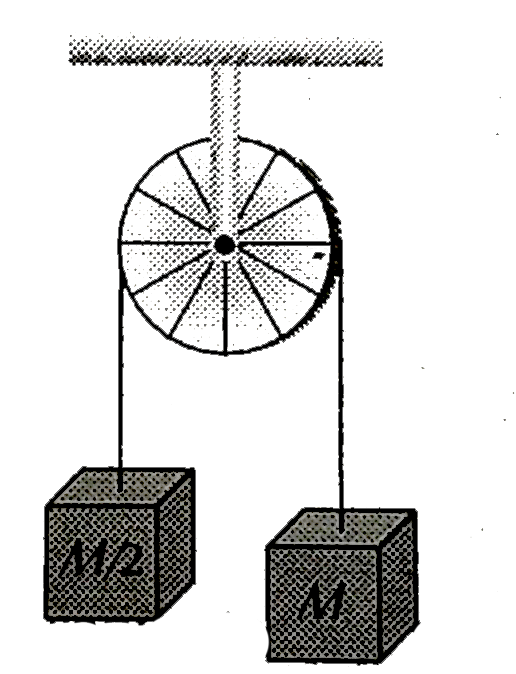
View Solution
