A heavy nucleous of masss M kept at rest in laboratory rejects a light particle of mass m .
Linear momentum of particle is more than linear momentum of remaining nucleus
Velocity of particle is more than velocity of nucleus .
Kinetic energy of the partcle is more than kinetic energy of the nucleus .
Kinetic energy of the nucleus is more than that of the particle .
The correct Answer is:B, C
To solve the problem, we will analyze the situation using the principles of conservation of momentum and kinetic energy.
1. Understanding the System:
- We have a heavy nucleus of mass M at rest.
- It ejects a light particle of mass m.
- After the ejection, the nucleus will have a reduced mass of M−m.
2. Applying Conservation of Momentum:
- Initially, the total momentum of the system is zero (since the nucleus is at rest).
- After the ejection, let the velocity of the ejected particle be v1 and the velocity of the remaining nucleus be v2.
- According to the conservation of momentum:
0=mv1+(M−m)v2
- Rearranging gives:
mv1=−(M−m)v2
- This implies:
mv1=(m−M)v2
3. Finding the Relationship Between Velocities:
- From the momentum equation, we can express v1 in terms of v2:
v1=−(M−m)mv2
- Since M is much larger than m, the absolute value of v1 will be greater than v2:
|v1|>|v2|
4. Kinetic Energy Calculation:
- The kinetic energy of the ejected particle KE1 is given by:
KE1=12mv21
- The kinetic energy of the remaining nucleus KE2 is:
KE2=12(M−m)v22
- We need to compare KE1 and KE2.
5. Substituting for v1:
- Substitute v1 into the kinetic energy equation:
KE1=12m(−(M−m)mv2)2=12m(M−m)2m2v22=(M−m)22mv22
- Now, for KE2:
KE2=12(M−m)v22
6. Comparing Kinetic Energies:
- To compare KE1 and KE2:
KE1=(M−m)22mv22andKE2=12(M−m)v22
- Dividing KE1 by KE2:
KE1KE2=(M−m)m
- Since M is much larger than m, (M−m)m>1, thus:
KE1>KE2
Conclusion:
- The correct options based on our analysis are:
- B: The velocity of the particle is greater than the velocity of the nucleus.
- C: The kinetic energy of the particle is greater than the kinetic energy of the nucleus.
- We have a heavy nucleus of mass M at rest.
- It ejects a light particle of mass m.
- After the ejection, the nucleus will have a reduced mass of M−m.
- Initially, the total momentum of the system is zero (since the nucleus is at rest).
- After the ejection, let the velocity of the ejected particle be v1 and the velocity of the remaining nucleus be v2.
- According to the conservation of momentum:
0=mv1+(M−m)v2
- Rearranging gives:
mv1=−(M−m)v2
- This implies:
mv1=(m−M)v2
- From the momentum equation, we can express v1 in terms of v2:
v1=−(M−m)mv2
- Since M is much larger than m, the absolute value of v1 will be greater than v2:
|v1|>|v2|
- The kinetic energy of the ejected particle KE1 is given by:
KE1=12mv21
- The kinetic energy of the remaining nucleus KE2 is:
KE2=12(M−m)v22
- We need to compare KE1 and KE2.
- Substitute v1 into the kinetic energy equation:
KE1=12m(−(M−m)mv2)2=12m(M−m)2m2v22=(M−m)22mv22
- Now, for KE2:
KE2=12(M−m)v22
- To compare KE1 and KE2:
KE1=(M−m)22mv22andKE2=12(M−m)v22
- Dividing KE1 by KE2:
KE1KE2=(M−m)m
- Since M is much larger than m, (M−m)m>1, thus:
KE1>KE2
- The correct options based on our analysis are:
- B: The velocity of the particle is greater than the velocity of the nucleus.
- C: The kinetic energy of the particle is greater than the kinetic energy of the nucleus.
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (D. Multiple Choice Questions)
10 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (Assertion Reason Type Questions)
13 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (JEE (Advanced) for IIT Entrance)
7 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A smooth wedge of mass M is kept at rest on a smooth horizontal surface . Inclined face of the wedge make an angle θ with the horizontal . A particle of mass m collides normal to inclined face of wedge . If speed of the particle just before collision is u and coefficient of restitution is e then find velocity of wedge after collision .
View SolutionFive particles each of mass 'm' are kept at five verties of a regular pentagon . A sixth particles of mass 'M' is kept at center of the pentagon 'O'.Distance between'M' and is 'm' is 'a'. Find
(a) net force on 'M'
(b) magnitude of net force on 'M' if any one particle is removed from one of the verties.
View Solution
Knowledge Check
Two bodies of mass M and 4M kept at a distance y apart. Where should a small particle of mass m be placed from M so that the net gravitational force on it is zero
Ay5By2Cy4Dy3A nucleus of mass M+Δm is at rest and decays into two daughter nuclei of equal mass M2 each. Speed of light is C.
The speed of deughter nuclei is :-Ac√ΔmM+mΔmBcΔmM+ΔmCc√2ΔmMDc√ΔmMA nucleus of mass M+Δm is at rest and decays into two daughter nuclei of equal mass M/2 each. Speed of light is C.
The speed of daughter nuclei isAcΔmM+ΔmBc√2ΔmMCc√ΔmMDc√ΔmM+Δm
A heavy ball of mass M is suspended from the ceiling of a car by a light string of mass m(m << M). The speed of transverse waves in the string is 50 ms -1 when the car is at rest. But if the car is imparted an acceleration of a, the wave speed increases to 51 ms−1. What is the value of a? [Take g=10ms−2]
View SolutionTwo interacting particles from a closed system whose centre of inertia is at rest. Figure illustrates the positions of both particles at a certain moment and the trajectory of the particle of mass m2. Draw the trajectory of the particle of mass m2 if m2=m1/2.
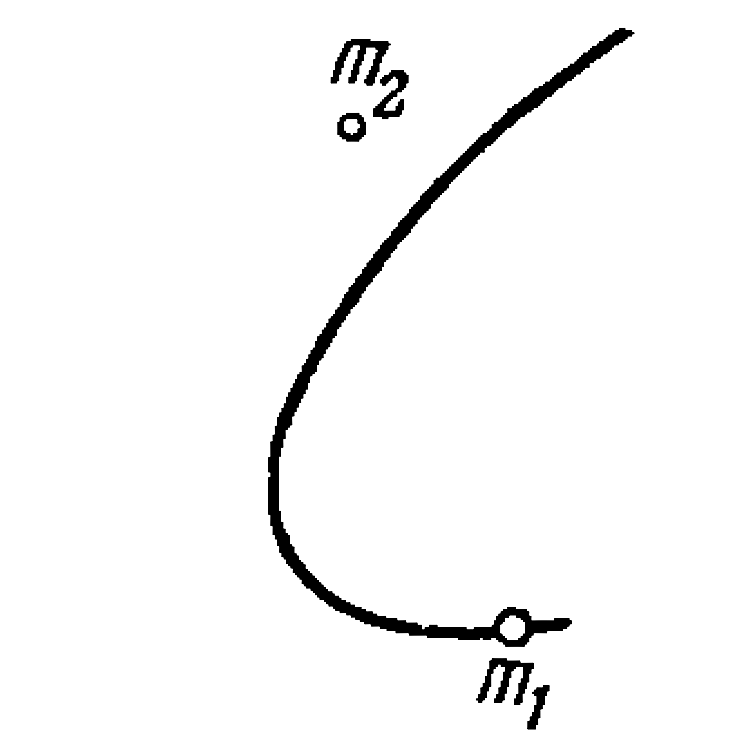
View SolutionA moving particle of mass m' makes head-on elastic collision with a particle of mass '2m' which is initially at rest.The fraction of K.E.lost by colliding particle is
View SolutionA particle of mass m travelling with velocity v and kinetic energy E collides elastically to another particle of mass nm, at rest. What is the fraction of total energy retained by the particle of mass m?
View SolutionTwo interacting particles form a closed system whose centre of mass is at rest. Fig. illustrates the positions of both particles at a certain moment and the trajectory of the particle of mass m1. Select the trajectory of the particle of mass m2 if m2=m1.

View Solution
