A homogeneous chain of length L lie on a table. If the coefficent of friction is μ , find the maximum length L1 of the chain which can hang from the edge of the table without the chain sliding down.
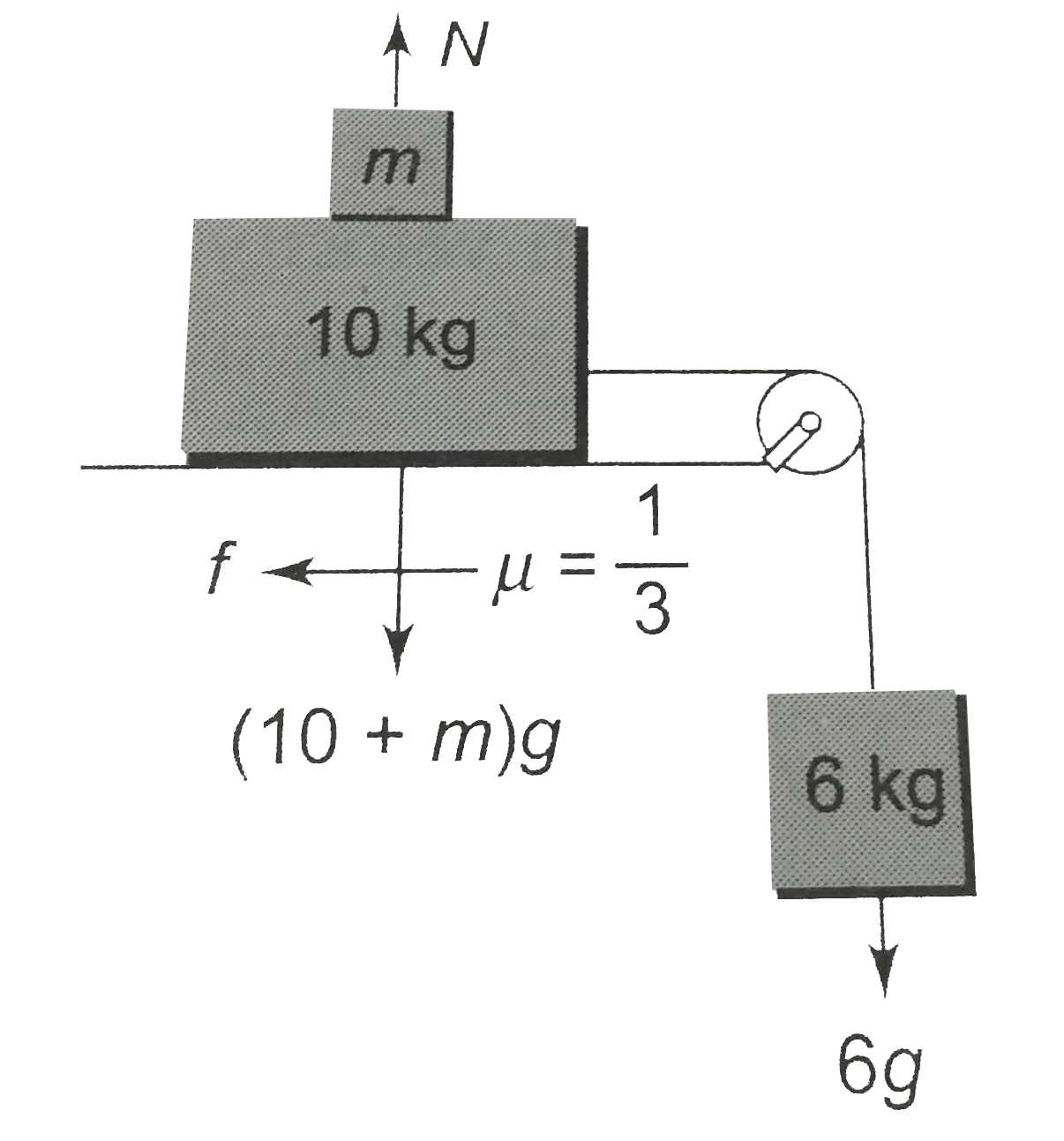
(b) Consider the situation as shown in the figure. Find teh minimum value of m so that the blocks do not move.
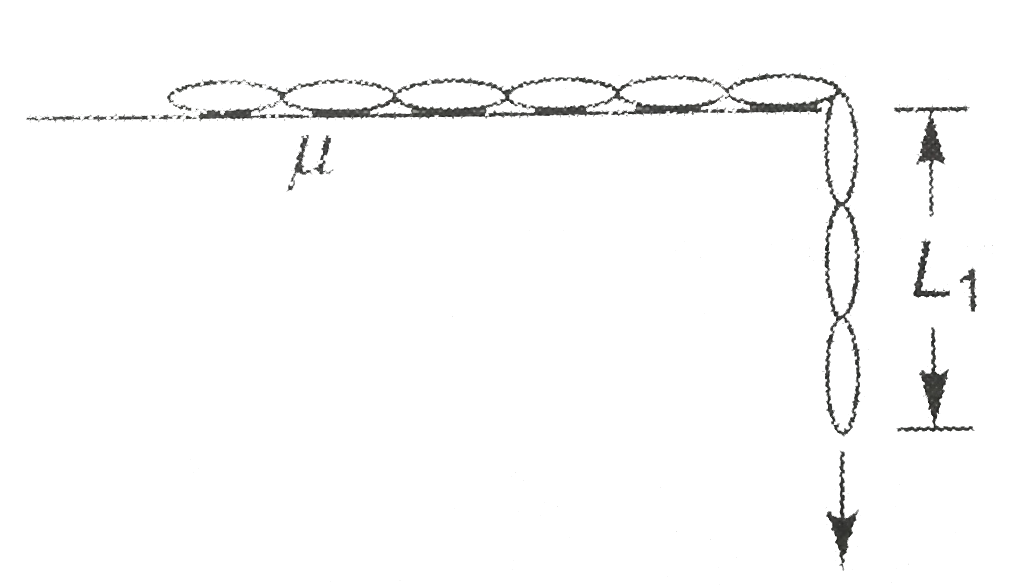

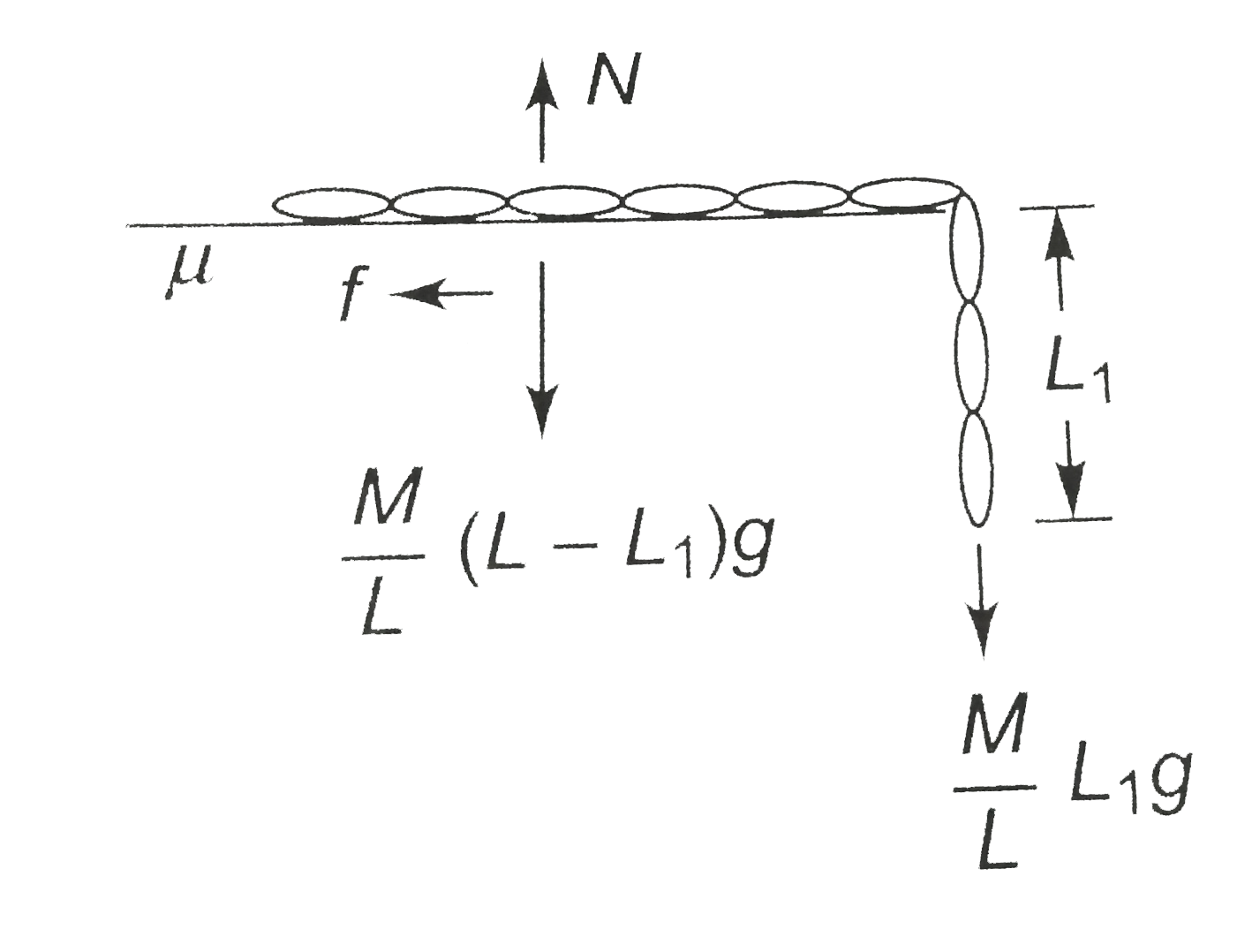
(a) Mass of chain on the table
=ML(L−L1)
N=ML(L−L1)g
f=μN=μM(L−L1)gL
Since the chain is in equilibrium
MLL1g≤f⇒MLL1g≤μM(L−L1g)L
L1≤μ(L−L1)⇒(1+μ)L1≤μL
L1≤μLμ+1⇒(L1)max=μLμ+1
(b) N=(10+m)g
f=μN=13(10+m)g
For the blocks to remain at rest
6g≤13(10+m)g
⇒≤(10+m)
m≥8,mmin=8kg.
Topper's Solved these Questions
Similar Questions
Assuming the length of a chain to be L and coefficient of static friction μ. Compute the maximum length of the chain which can be held outside a table without sliding.
View SolutionAssuming the length of a chain to be L and coefficient of static friction μ. Compute the maximum length of the chain which can be held outside a table without sliding.
View Solution
Knowledge Check
- Question 1 - Select One
Consider the situation as shown in the figure.
The maximum value of m so that the blocks do not move is
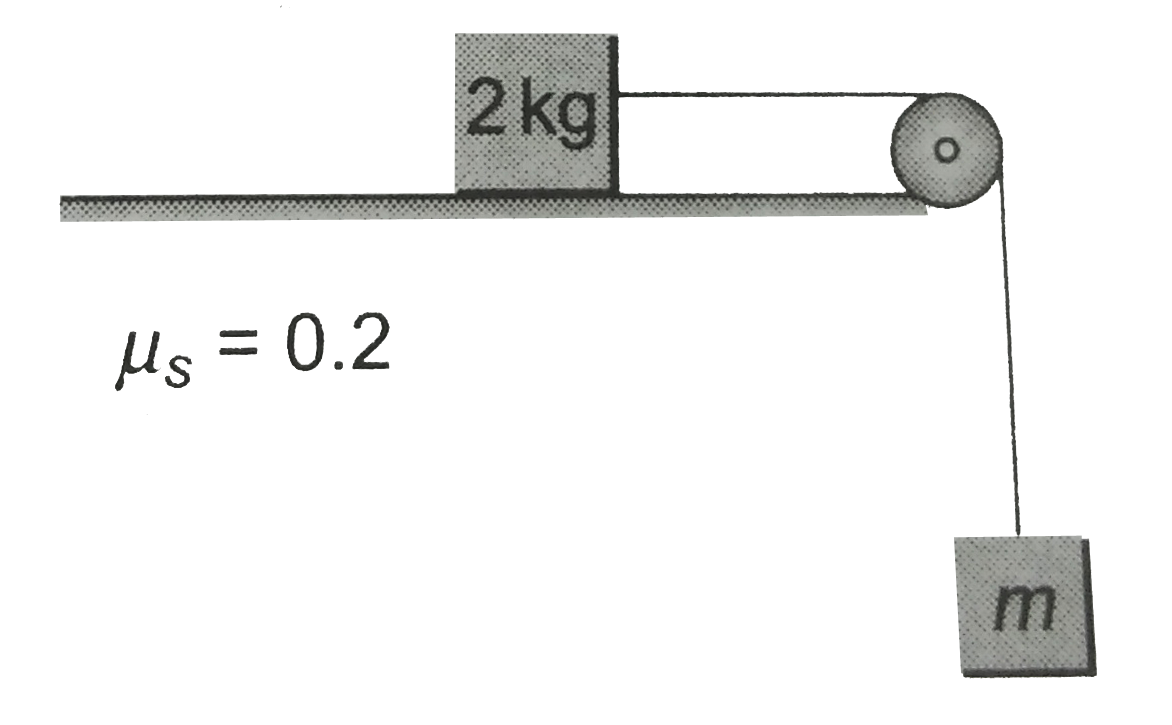 A0.2kgB0.4kgC0.6kgD0.8kg
A0.2kgB0.4kgC0.6kgD0.8kg - Question 2 - Select One
A uniform rope of length l lies on a table . If the coefficient of friction is μ , then the maximum length l1 of the part of this rope which can overhang from the edge of the table without sliding down is :
A1μB1μ+1Cμlμ+1Dμlμ−1 - Question 3 - Select One
A uniform rope of length l lies on a table. If the coefficient of friction is μ, then the maximum length L of the part of this rope which can overhang from the edge of the table without sliding down is
AlμBlμ+1Cμl1+μDμlμ−1
A uniform rope of length l lies on a table if the coefficient of friction is , then the maximum length l, of the part of this rope which can over hang from the edge of the table without sliding down is -
View SolutionA uniform rope of ≤n>hLmeters is lying over a table. If the coefficient of friction be μ, then the maximum length L1 of the part of the rope which can overhang the edge without sliding is
View SolutionA heavy uniform chain lies on horizontal table top. If the coeficient of friction 0.25, then the maximum friction of length of the chain that can hang over on edge of the table is
View SolutionA homogoneous chain of length L lies on a table. The coefficient of friction between the chain and the table is μ. The maximum length which can hang over the table in equilibrium is
View SolutionA heavy uniform chain lies on a horizontal table-top. If the coefficient of friction between the chain and table surface is 0.25, then the maximum fraction of length of the chain, that can hang over one edge of the table is
View Solution

