A horizontal constant force F pulls a block of mass m placed on a horizontal surface. If the coefficient of kinetic friction between the block and ground is μ, find the power delivered by the external agent after a time agent after a time t measured from the beginning of action of the force.
To solve the problem, we need to find the power delivered by the external agent after a time t when a constant force F is applied to a block of mass m on a horizontal surface with a coefficient of kinetic friction μ.
1. Identify Forces Acting on the Block:
- The gravitational force acting downwards: mg
- The normal force acting upwards: N=mg
- The applied force F acting horizontally to the right.
- The frictional force acting horizontally to the left, which is given by fk=μN=μmg.
2. Write the Equation of Motion:
- According to Newton's second law, the net force acting on the block is equal to the mass times its acceleration:
F−fk=ma
- Substituting the expression for friction:
F−μmg=ma
- Rearranging gives:
a=F−μmgm
3. Calculate the Velocity After Time t:
- Since the initial velocity u=0, the velocity v after time t can be calculated using the formula:
v=u+at=0+(F−μmgm)t
- Thus, we have:
v=(F−μmg)tm
4. Calculate the Power Delivered by the External Agent:
- Power P is defined as the rate at which work is done, which can also be expressed as the dot product of force and velocity:
P=F⋅v
- Since the force F and velocity v are in the same direction, we can simplify this to:
P=F⋅v=F⋅((F−μmg)tm)
- Therefore, the power delivered by the external agent is:
P=F(F−μmg)tm
Final Answer:
The power delivered by the external agent after a time t is:
P=F(F−μmg)tm
- The gravitational force acting downwards: mg
- The normal force acting upwards: N=mg
- The applied force F acting horizontally to the right.
- The frictional force acting horizontally to the left, which is given by fk=μN=μmg.
- According to Newton's second law, the net force acting on the block is equal to the mass times its acceleration:
F−fk=ma
- Substituting the expression for friction:
F−μmg=ma
- Rearranging gives:
a=F−μmgm
- Since the initial velocity u=0, the velocity v after time t can be calculated using the formula:
v=u+at=0+(F−μmgm)t
- Thus, we have:
v=(F−μmg)tm
- Power P is defined as the rate at which work is done, which can also be expressed as the dot product of force and velocity:
P=F⋅v
- Since the force F and velocity v are in the same direction, we can simplify this to:
P=F⋅v=F⋅((F−μmg)tm)
- Therefore, the power delivered by the external agent is:
P=F(F−μmg)tm
The power delivered by the external agent after a time t is:
P=F(F−μmg)tm
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples15videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.125videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A block of mass m is moving with a constant acceleration a on a rough horizontal plane. If the coefficient of friction between the block and ground is μ, the power delivered by the external agent in a time interval t from the beginning is equal to:
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A block of mass m is slowly pulled along a curved surface from position 1 and 2. If the coefficient of kinetic friction between the block and surface is μ, find the work done by the applied force.
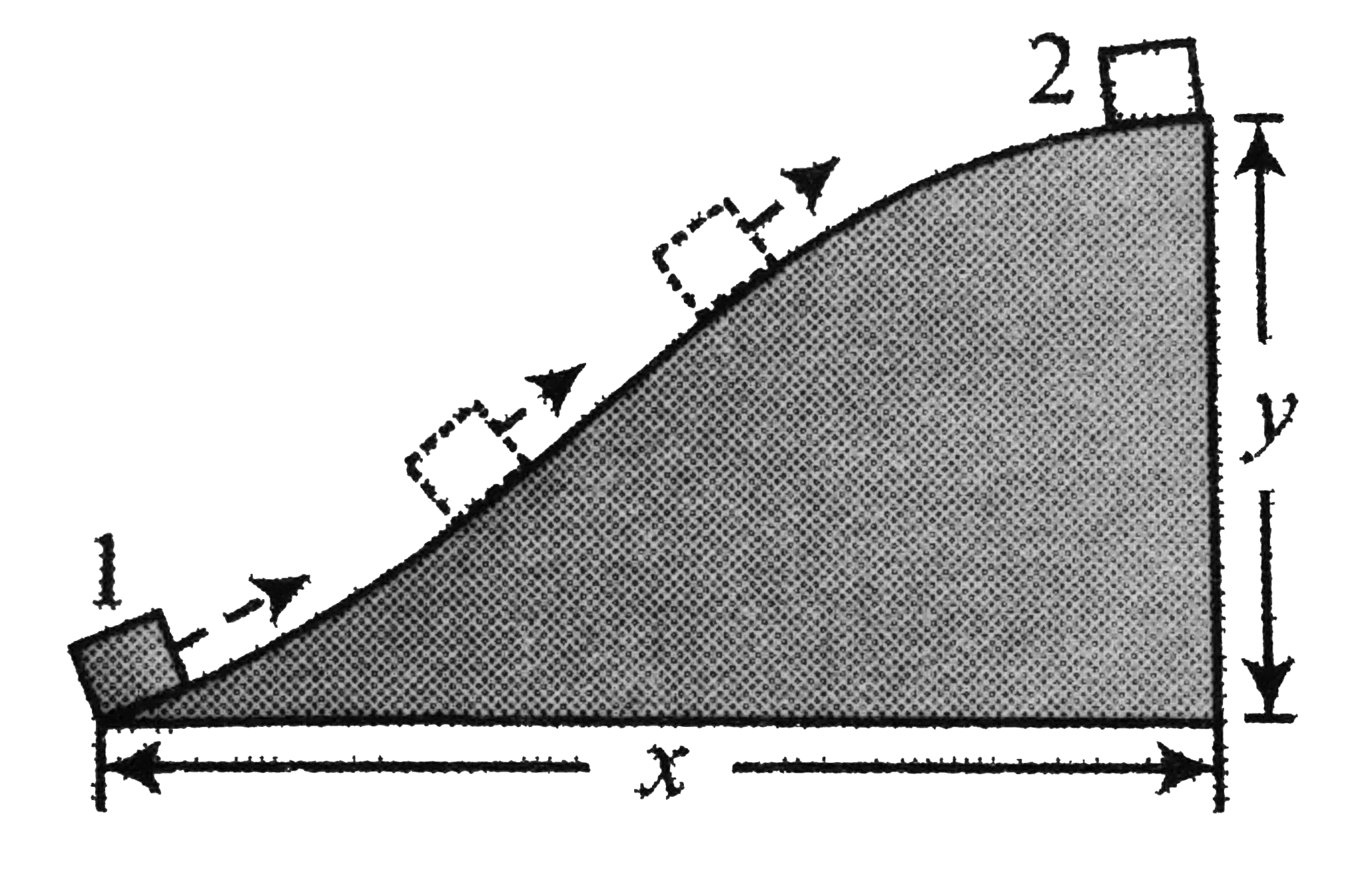 View Solution
View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal. If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View SolutionA block of mass M is kept on as rough horizontal surface. The coefficient of static friction between the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
View SolutionA block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass M is kept on as rough horizontal surface. The coefficient of static frictionbetween the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA horizontal force F pulls a 20 kg box at a constant speed along a rough horizotal floor. The coefficient of friction between the box and the floor is 0.25. The work done by force F on the block in displacing it by 2 m is
View Solution

