A hot body placed in a surrounding of temperature θ0 obeys Newtons's law of cooling dθ/dt=−k(θ−θ0). Its temperature at t=0 is θ1. The specific heat capacity of the body is s and its mass ism.
Find
(a) the maximum heat that the body can lose and
(b) the time starting from t=0 in which it will lose 90% of this maximum heat.
(a) The body will lose heat until its temperature equals that of surrounding
(ΔQ)max=mx(θ1−θ0)
(b) Let in time t, body loses 90% of (ΔQ)max. It will lose 10% of (ΔQ)max further.
110(ΔQ)max=ms(θ−θ0)
110(θ1−θ0)max=ms(θ−θ0)
(θ−θ0)=110(θ1−θ0)
The temperatuure difference of body and surrouding after time t
(θ−θ0)=(θ1−θ0)e−kt
110(θ1−θ0)=(θ1−θ0)e−kt
ekt=10
kt1ne=10
t=1n10k
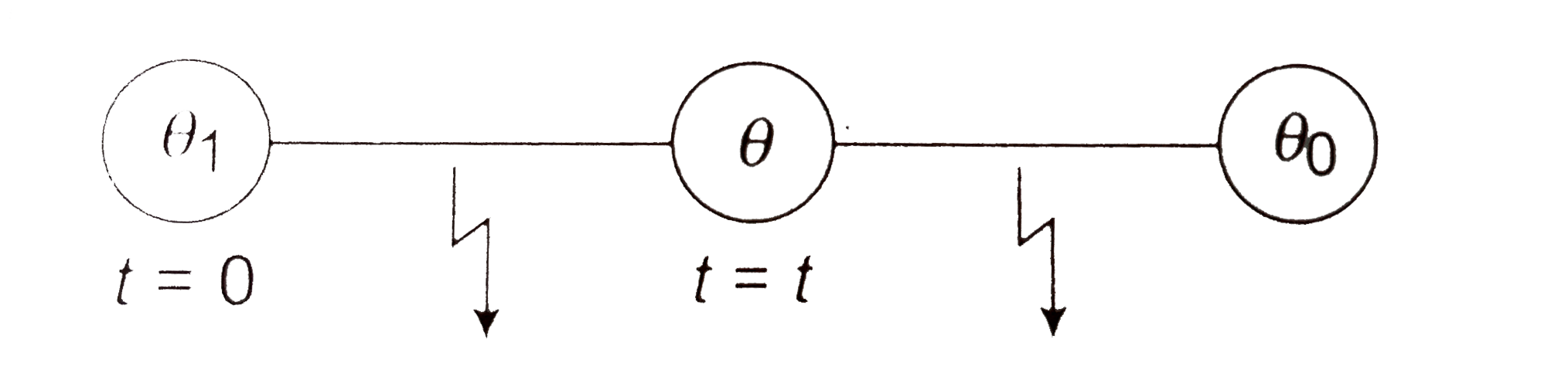
Topper's Solved these Questions
Similar Questions
In Newton's law of cooling dθdt=−k(θ−θ0) the constant k is proportional to .
View SolutionA hot body placed in air is cooled down according to Newton's law of cooling, the rate of decrease of temperature being k times the temperature difference from the surrounding. Starting from t=0 , find the time in which the body will lose half the maximum heat it can lose.
View Solution
Knowledge Check
In Newton's law of cooling dθdt=−k(θ−θ0) the constant k is proportional to .
AA: surface of the bodyBS in the specific heat of the bodyC1m being the mass of the bodyDe is the emissivity of the bodyIn Newton's law of cooling experiment, we plot a graph of (dθdt) against the temperature difference (θ−θ0). The graph is
Aa curve passing through the originBa straight line passing through the originCa straight line having an intercept on the y axisDa straight line having an intercept on the temperature axisAccording to Newton’s law of cooling ms dθdt=−K(θ−θ0). If σ is Stefan’s constant, A is surface area of the body and T∘ is temperature of surroundings in K, then the value of K on the basis of Stefan-Boltzmann law is –
AσAT40BσAT30C4σAT40D4σAT30
A hot body placed in air is cooled down according to Newton's law of cooling, the rate of decrease of temperature being k times the temperature difference from the surrounding. Starting from t=0 , find the time in which the body will loss half the maximum heat it can lose.
View SolutionA hot fbody placed in air is cooled down according to Newton's law of cooling, the rate of decrease of temperature being k times the temperature difference from the surrounding. Starting from t=0 , find the time in which the body will loss half the maximum heat it can lose.
View SolutionNewton's law of cooling applies when a body is losing heat to its surroundings by
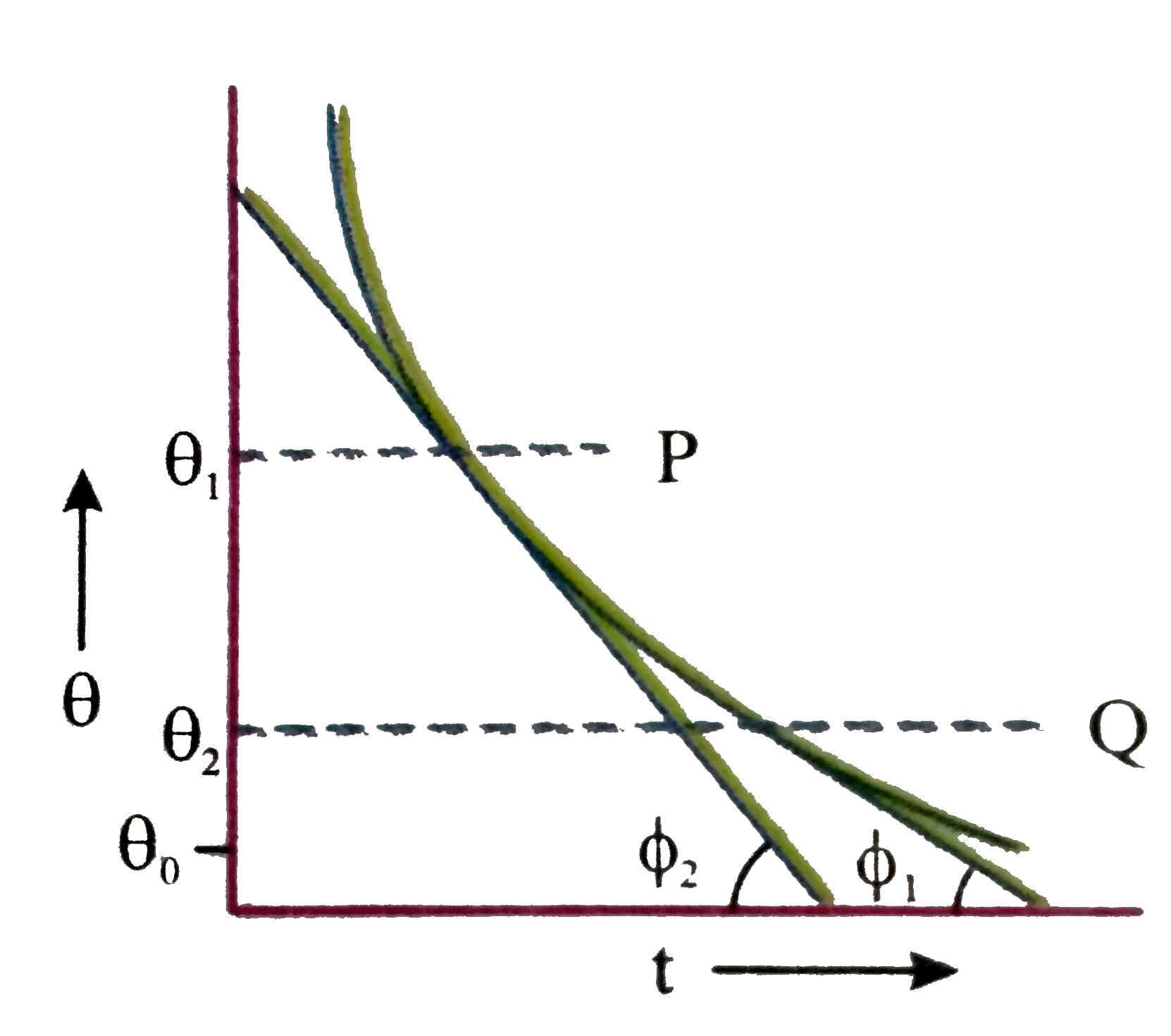
View SolutionA body cools in a surrounding which is at a constant temperature of θ0 Assume that it obeys Newton's law of cooling Its temperature θ is plotted against time t Tangents are drawn to the curve at the points P(θ=θ1) and Q(θ=θ2) These tangents meet the time axis at angle of ϕ2 andϕ1 as shown
 .
.
View SolutionAccording to Newton’s law of cooling. The rate of loss of heat, −dθ/dtof the body is
View Solution
