A hydraulic automobile lift is designed to lift cars with a maximum mass of 300 kg. the area of cross-section of the piston carrying the load is 425cm3. What maximum pressure would smaller piston have to bear?
To solve the problem, we need to calculate the maximum pressure that the smaller piston must bear when lifting a car with a maximum mass of 300 kg. Here are the steps to find the solution:
Step 1: Calculate the Force Exerted by the Car
The force exerted by the car can be calculated using the formula:
F=m⋅g
where:
- F is the force,
- m is the mass of the car (300 kg),
- g is the acceleration due to gravity (approximately 9.8m/s2).
Substituting the values:
F=300kg⋅9.8m/s2=2940N
Step 2: Convert the Area of Cross-Section from cm² to m²
The area of the cross-section of the piston is given as 425cm2. To convert this to square meters:
A=425cm2⋅(1m210000cm2)=425×10−4m2
Step 3: Calculate the Pressure
Pressure can be calculated using the formula:
P=FA
Substituting the values we have:
P=2940N425×10−4m2
Calculating this gives:
P=29400.0425≈69,058.82Pa
or in scientific notation:
P≈6.91×105Pa
Final Answer
The maximum pressure that the smaller piston has to bear is approximately:
P≈6.92×105Pa
---
The force exerted by the car can be calculated using the formula:
F=m⋅g
where:
- F is the force,
- m is the mass of the car (300 kg),
- g is the acceleration due to gravity (approximately 9.8m/s2).
F=300kg⋅9.8m/s2=2940N
The area of the cross-section of the piston is given as 425cm2. To convert this to square meters:
A=425cm2⋅(1m210000cm2)=425×10−4m2
Pressure can be calculated using the formula:
P=FA
Substituting the values we have:
P=2940N425×10−4m2
P=29400.0425≈69,058.82Pa
or in scientific notation:
P≈6.91×105Pa
The maximum pressure that the smaller piston has to bear is approximately:
P≈6.92×105Pa
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE
31 Videos
- LAWS OF MOTION BOOK - NCERT ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - EXERCISE
40 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE
21 Videos
Similar Questions
A hydraulic automobile lift is designed to lift cars with a maximum mass of 3000 kg. The area of cross-section of the piston carrying the load is 425 cm^(2). What maximum pressure would the small piston have to bear?
View SolutionA hydraulic automobile lift is designed to lift cars with a maximum mass of 3000Kg. The area of cross section of the piston carrying the load is 425cm2. What maximum pressures would the smaller piston have to bear?
View SolutionWhen a pressure measuring device is immersed in a liquid, a restoring force of 2 N is exerted by the spring. If the area of cross-section of the pistion is 10 cm2, what pressure does the fluid exert on the piston?
View Solution2.00 mole of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston. The piston is connected to a vertical spring of spring constant 200Nm−1 as shown in .the area of cross section of the cylinder is 20.0cm2. initially, the spring is at its natural length and the temperature of the gas is 300K at its natural length. the atmosphere pressure is 100 kPa. the gas is heated slowly for some time by means of an electric heater so as to move the position up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
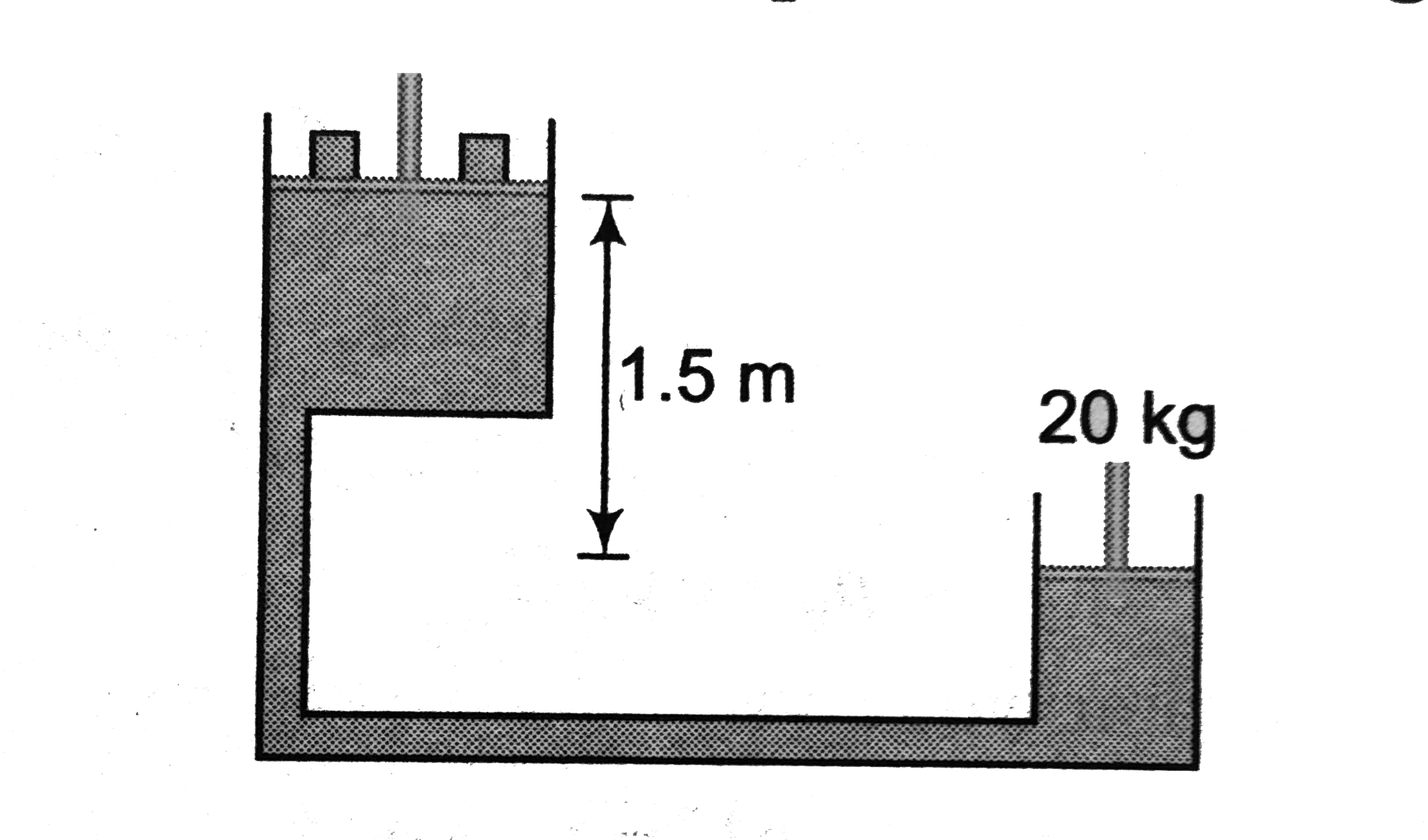
View SolutionFigure shown a hydraulic press with the larger piston if diameter 35 cm at a height of 1.5 cm at a height of 1.5 m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20 kg. What is the force exerted on the load by the larger piston? The density of oil in the press is 750kh/m3 . (Take g=9.8m/s2)
 .
.
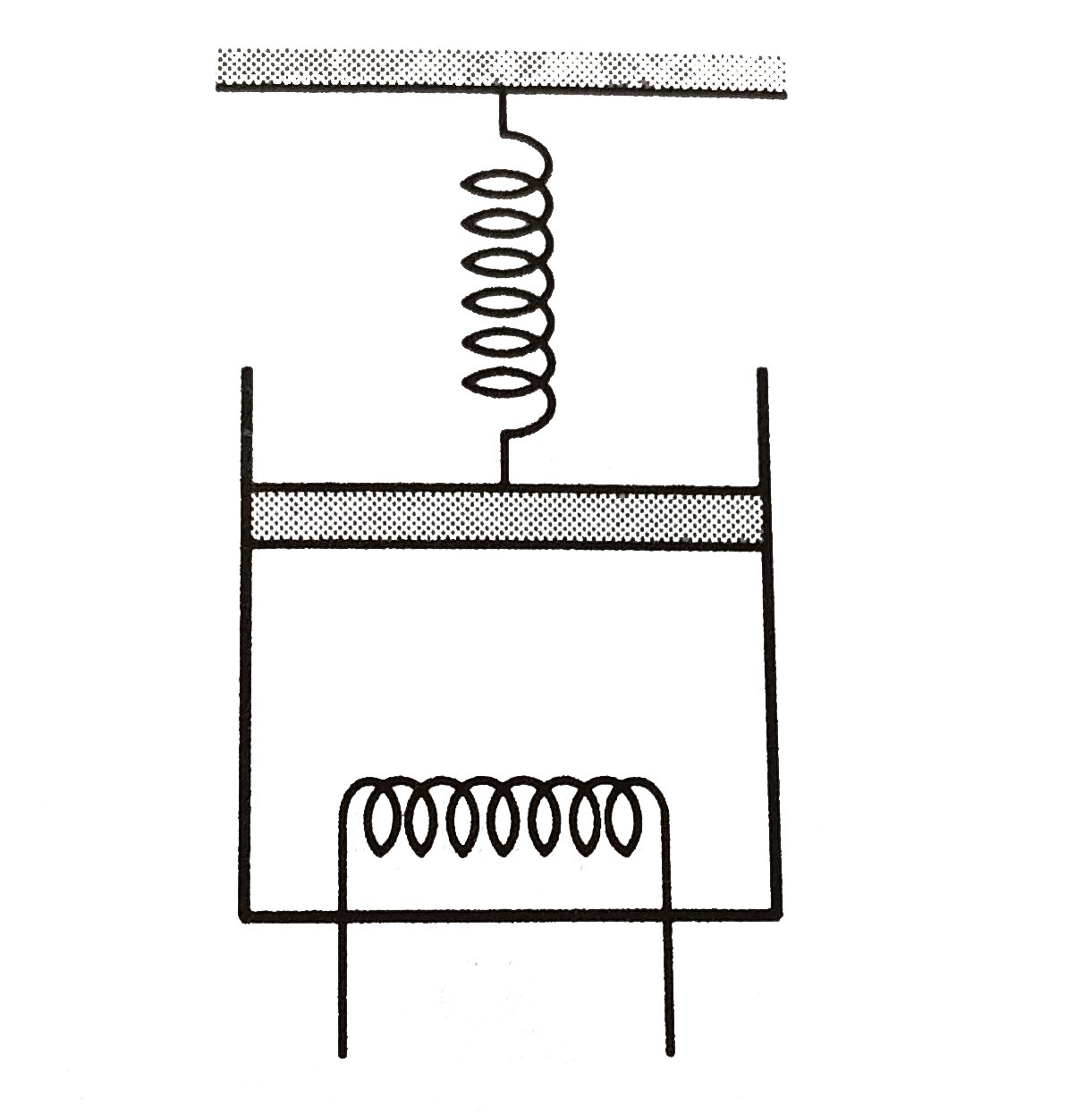
View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionA bob of mass 10kg is attached to a wire 0.3m long. Its breaking stress is 4.8×107N/m2. Then area of cross-section of the wire is 10−6m2. What is the maximum angular velocity with which it can be rotated in a horizontal circle?
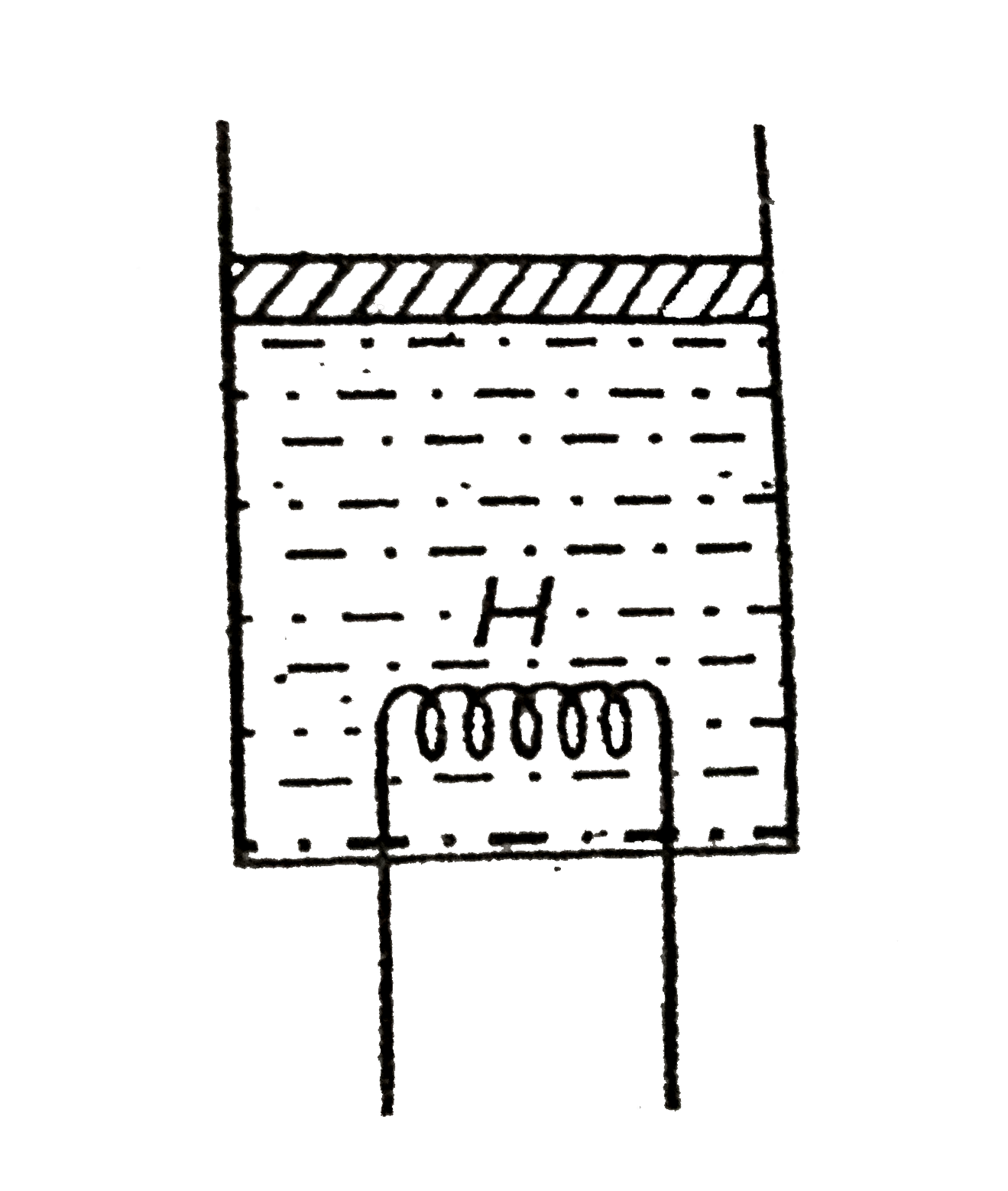
View Solution2 moles of a diatomic gas are enclosed in a cylinder piston arrangment. The area of cross section and mass of the piston are 1cm2 and 1 kg respectively. A heater is supplying heat to the gas very slowly. Find heat supplied (in joule) by the heater is the piston moves through a distance of 10 cm.
 .
.
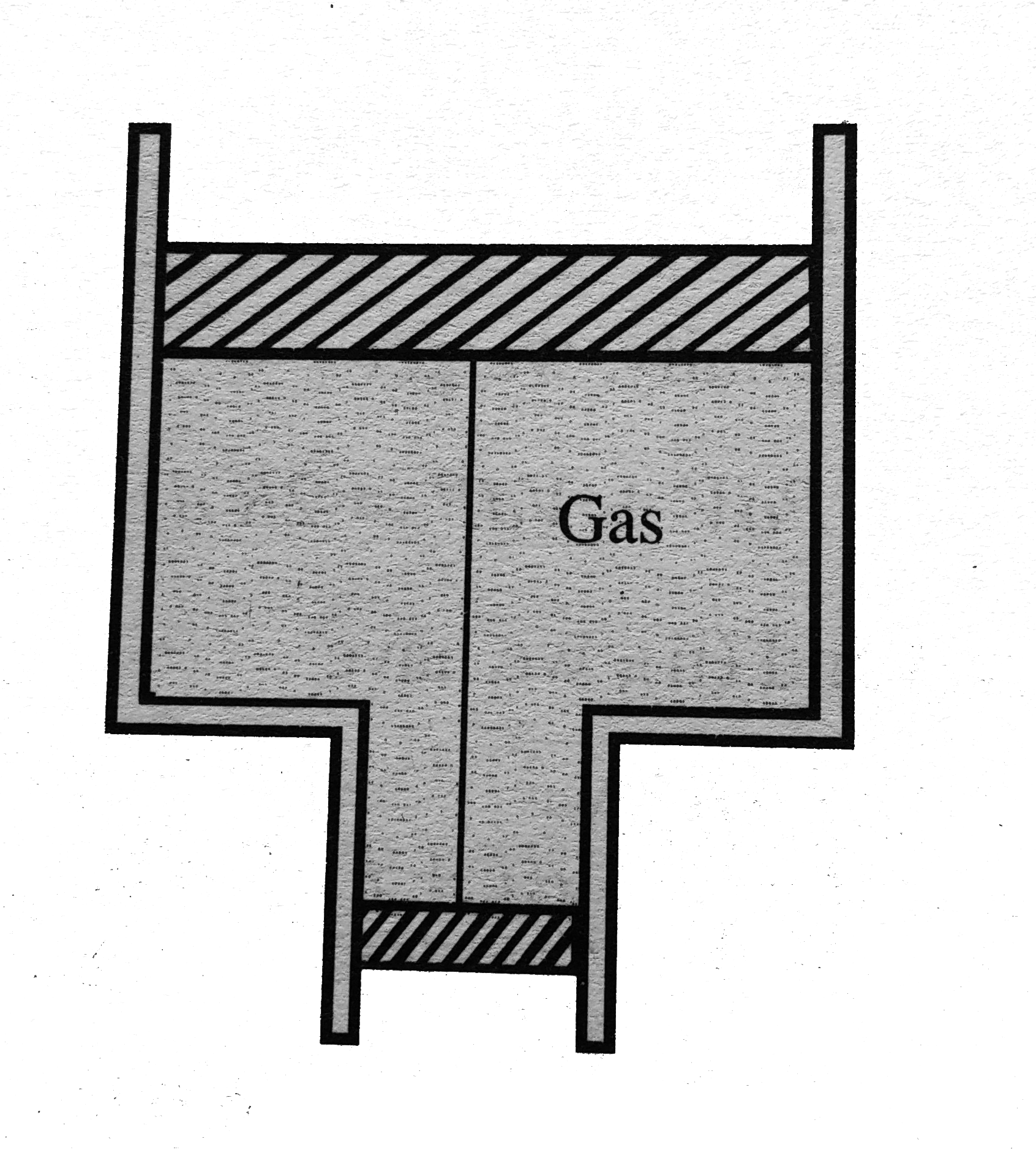
View SolutionA smooth vertical tube having two different cross sections is open from both the ends but closed by two sliding pistions as shown in Fig. and tied with an inextensible string. One mole of an ideal gas is enclosed between the piston The difference in cross-sectional areas of the two pistons is given ΔS. The masses of piston are m1 and m2 for larger and smaller one, respectively. Find the temperature by which tube is raised so that the pistons will be displaced by a distance l. Take atmospheric pressure equal to P0
View Solution
