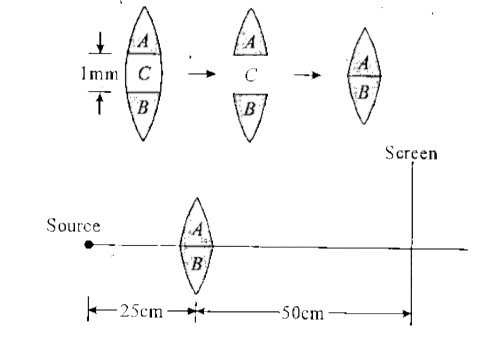
A lens of diameter $5.0 c m$ and focal length $f = 25.0 c m$ was cut along the diameter into two identical halves. In the process, the layer of the lens $α = 1.00 m m$ in thieckness was lost. Then the halves were put together to form a composite lens. In this focal plane a narrow slit was placed, emitting monochromatic light with wavelength $λ = 0.60 μ m$ . Behind the lens a screen was located at a distance $b = 50 c m$ from it. Find:
(a) the which of a fringe on the screen and the number of possible maxima,
(b) the maximum width of the slit $\partial_{max}$ at which the fringes on the screen will be still observed sufficiently sharp.

Video Solution

Text Solution

Verified by Experts

(a) We show the upper half o fthe lens. The emergent light is at an angle $\frac{a}{2 f}$ from the axis.
Thus the divergence angle of the two incident light beams is
$ψ = \frac{a}{f}$
When they interfere the fringes produced have a width
$Δ x = \frac{λ}{ψ} = \frac{f λ}{a} = 0.15 m m$ .
The patch on the screen illuminated by both light has s width $b ψ$ and this contains
$\frac{b ψ}{Δ x} = \frac{b a^{2}}{f^{2} λ}$ fringes $= 13$ fringes
(if we ignore $1$ in comparies on to $\frac{b ψ}{Δ x}$ (if 5.71(a))
(b) We follow the logic of. From one edge of the slit to the edge the distance is of magnitude $\partial$ (i.e $\frac{a}{2}$ to $\frac{a}{2} + \partial)$ .
If we imagine teh edge to shift by this distance, the angle $ψ / 2$ will increase by $\frac{Δ ψ}{2} = \frac{\partial}{2 f}$
and the light will shift $\pm b \frac{\partial}{2 f}$
The fringe patten will therefore shift by $\frac{\partial . b}{f}$
Equating this to $\frac{δ x}{2} = \frac{f λ}{2 a}$ we get $\partial_{max} = \frac{f^{2} λ}{2 a b} = 37.5 μ m$ .

A lens of focal length f is cut along the diameter into two identical halevs. In this process, a layer of the lens t in thickness is lost, then the halves are put together to form a composite lens. In between the focal plane and the composite lens, a narrow slit is placed near the focal plane. The slit is emitting monochromatic $λ$ . Behind the lens, a screen is located at a distance L front it.

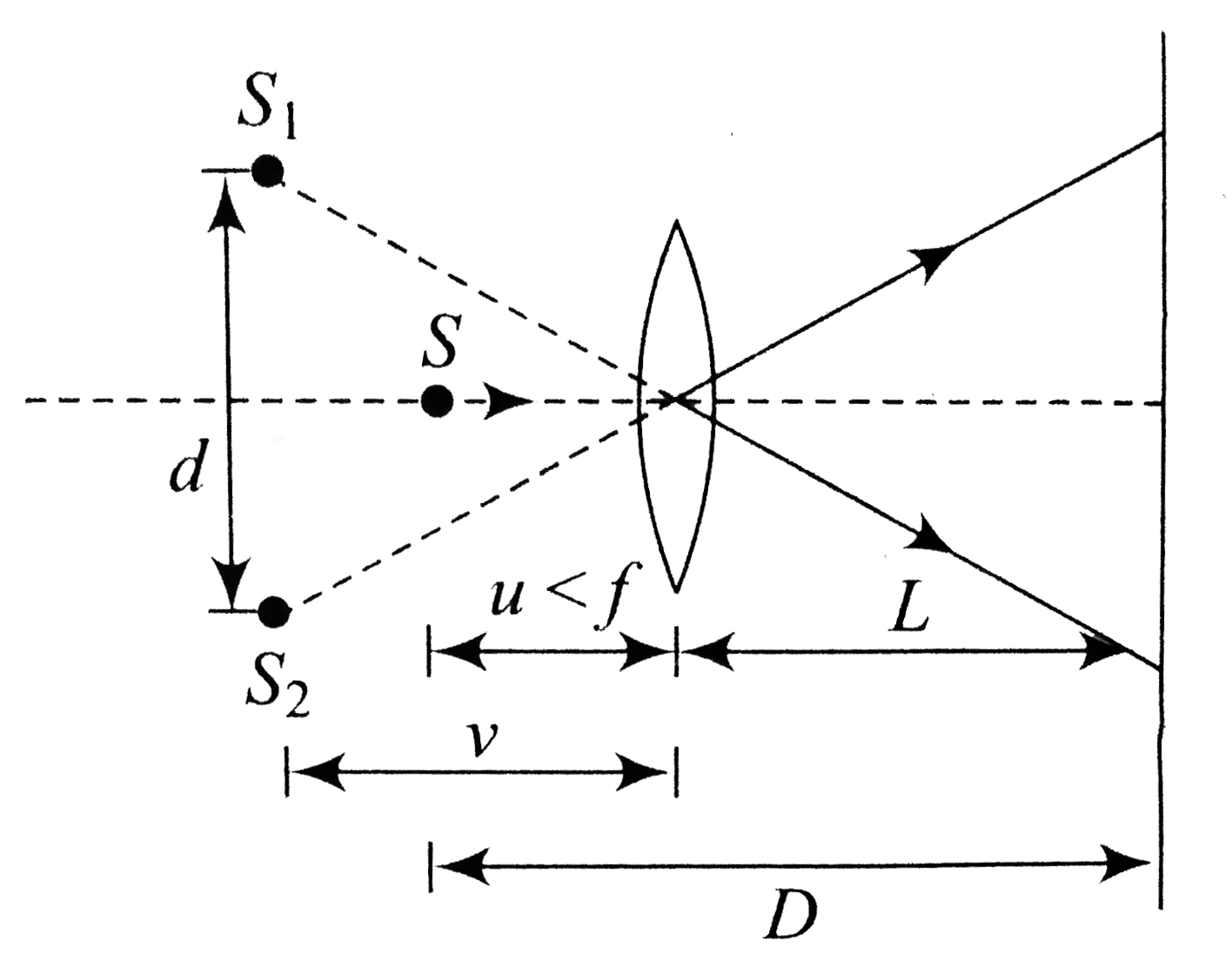
Find the fringe width for the pattern obtained under given arrangement on the screen.
A $\frac{λ f}{2 t}$
B $\frac{λ f}{t}$
C $\frac{t f}{λ}$
D $\frac{t f}{λ 2}$
A lens of focal length f is cut along the diameter into two identical halevs. In this process, a layer of the lens t in thickness is lost, then the halves are put together to form a composite lens. In between the focal plane and the composite lens, a narrow slit is placed near the focal plane. The slit is emitting monochromatic $λ$ . Behind the lens, a screen is located at a distance L front it.

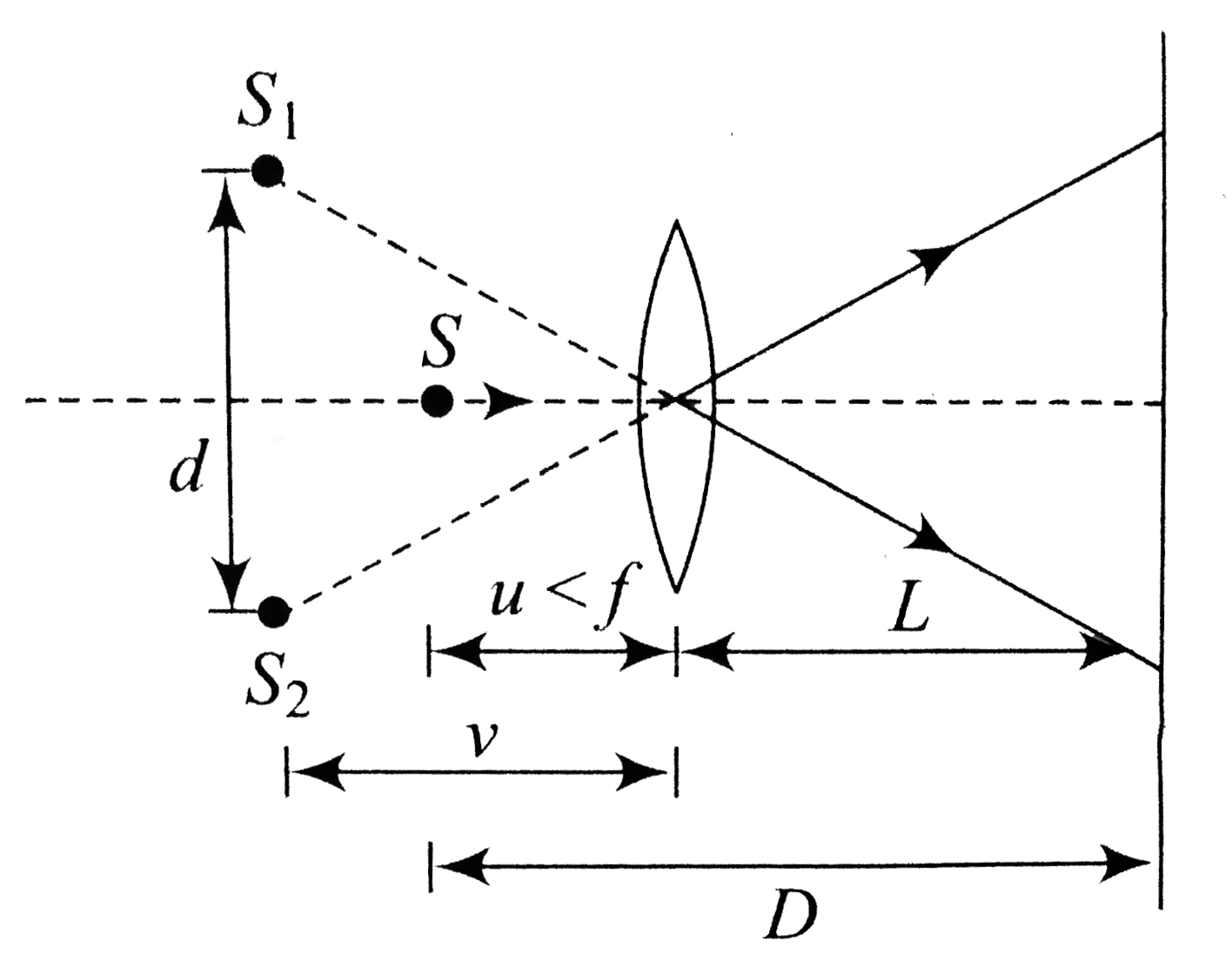
The expression for the number of visible maxima which are obtained through above said arrangement will turn out to be
A $\frac{L t^{2}}{λ f^{2}}$
B $\frac{2 L t^{2}}{λ f^{2}}$
C $\frac{L t}{2 λ f^{2}}$
D $\frac{L t^{2}}{2 λ f^{2}}$
The focal length of a lens is 0.1 m. Then the lens must be
Aconvex
Bconcave
Ccylindrical
Dnone of these