A Long straight conductor carrying a current of 2 A is in parallel to another conductor of length 5 cm. and carrying a current 3A. They are separated by a distance of 10 cm. Calculate (a) B due to first conductor at second conductor (b) the force on the short conductor.
To solve the problem, we will follow these steps:
Step 1: Calculate the Magnetic Field (B) due to the first conductor at the location of the second conductor.
The formula for the magnetic field B at a distance D from a long straight conductor carrying a current I is given by:
B=μ0I2πD
Where:
- μ0=4π×10−7T m/A (permeability of free space)
- I=2A (current in the first conductor)
- D=0.1m (distance between the two conductors)
Substituting the values into the formula:
B=4π×10−7×22π×0.1
The 2π cancels out:
B=4×10−7×20.1=8×10−70.1=8×10−6T
Step 2: Calculate the Force (F) on the second conductor.
The force F on a current-carrying conductor in a magnetic field is given by:
F=I⋅L⋅B⋅sin(θ)
Where:
- I=3A (current in the second conductor)
- L=0.05m (length of the second conductor, converted from 5 cm)
- B=8×10−6T (magnetic field calculated in Step 1)
- θ=90∘ (angle between the current direction and magnetic field direction, hence sin(90∘)=1)
Substituting the values into the formula:
F=3⋅0.05⋅8×10−6⋅1
Calculating the force:
F=3⋅0.05⋅8×10−6=1.2×10−6N
Final Answers:
(a) The magnetic field B due to the first conductor at the second conductor is 8×10−6T.
(b) The force on the short conductor is 1.2×10−6N.
---
- μ0=4π×10−7T m/A (permeability of free space)
- I=2A (current in the first conductor)
- D=0.1m (distance between the two conductors)
- I=3A (current in the second conductor)
- L=0.05m (length of the second conductor, converted from 5 cm)
- B=8×10−6T (magnetic field calculated in Step 1)
- θ=90∘ (angle between the current direction and magnetic field direction, hence sin(90∘)=1)
(a) The magnetic field B due to the first conductor at the second conductor is 8×10−6T.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IA
142 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IB
71 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED) Integer Type Questions
3 Videos
Similar Questions
Knowledge Check
A conductor of length 2 m carrying current 2 A is held parallel to an infinitely long conductor carrying current of 12 A at a distance of 100 mm, the force on small conductor is
A8.6×10−5NB6.6×10−5NC7.6×10−5ND9.6×10−5N
Find the magnetic induction due to a straight conductor of length 16cm carrying current of 5A at a distance of 6cm from the midpoint of conductor
View SolutionA conductor AB of length l carrying current i is placed perpendicular to a long straight conductor carrying a current I as shown. Force on AB will be
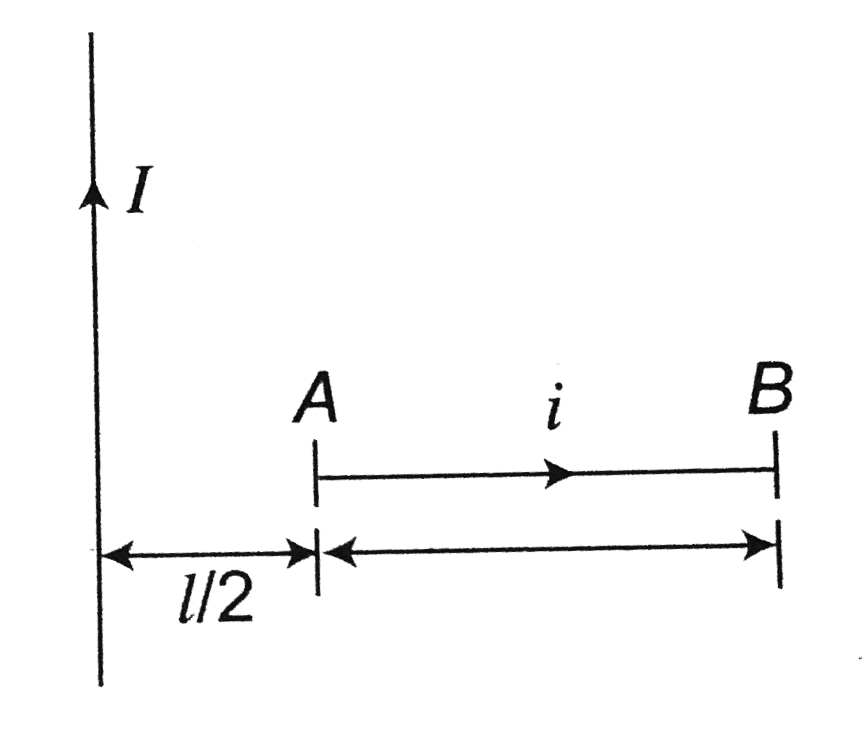
View SolutionAB and CD are long straight conductor, distance d apart, carrying a current l. The magnetic field at the midpoint of BC is

View SolutionA long straight conductor carrying current I1 is placed in the plane of a ribbon carrying current I2 parallel to the previous one. The width of the ribbon is b and the straight conductor is at a distance a from the near endge. Find the force of attraction between the two.

View SolutionA straight conductor of length 32 cm carries a current of 30A. Magnetic induction at a point which is in air at a perpendicular distance of 12cm from the mid point of the conductor is
View SolutionThree long straight conductors are arranged parallel to each other in the same plane and carryin currents of 1A,2A and3A all in the same direction. The distance betweent eh first two conductros is x and the distance between the second and third conductorsy. If the middle conductor is in equilibrium, the ratio x:y is
View SolutionA conductor-carries a current of 50μA If the area of cross-section of the conductor is 50mm2, then value of the current density in Am−2
View Solution


