A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1.2×10−1T. If the dipole comes to stable equilibrium at an angle of 30∘ with this field, then the magnitude of the field (in tesla) is x×102T. Find x,
To solve the problem, we need to analyze the magnetic dipole under the influence of two magnetic fields. Let's denote the two magnetic fields as B1 and B2.
1. Identify Given Values:
- The magnitude of one magnetic field B1=1.2×10−1T.
- The angle between the two magnetic fields θ=60∘.
- The angle at which the dipole is in stable equilibrium with B1 is ϕ=30∘.
2. Torque Equilibrium Condition:
In stable equilibrium, the torque due to the two magnetic fields must balance each other. The torque τ on a magnetic dipole in a magnetic field is given by:
τ=mBsin(ϕ)
where m is the magnetic moment of the dipole.
3. Set Up the Torque Equations:
- For the magnetic field B1:
τ1=mB1sin(30∘)
- For the magnetic field B2:
τ2=mB2sin(30∘)
Since the dipole is in stable equilibrium, we have:
τ1=τ2
4. Equate the Torques:
mB1sin(30∘)=mB2sin(30∘)
The m and sin(30∘) terms cancel out, leading to:
B1=B2
5. Calculate B2:
We know that:
B1=1.2×10−1T
Therefore, we can express B2 in terms of B1:
B2=B1=1.2×10−1T
6. Express B2 in Required Form:
The problem states that the magnitude of the field B2 can be expressed as x×102T. Thus:
B2=x×102T
Setting the two expressions for B2 equal gives:
x×102=1.2×10−1
7. Solve for x:
Rearranging the equation:
x=1.2×10−1102=1.2×10−3
Final Answer:
The value of x is 1.2.
- The magnitude of one magnetic field B1=1.2×10−1T.
- The angle between the two magnetic fields θ=60∘.
- The angle at which the dipole is in stable equilibrium with B1 is ϕ=30∘.
In stable equilibrium, the torque due to the two magnetic fields must balance each other. The torque τ on a magnetic dipole in a magnetic field is given by:
τ=mBsin(ϕ)
where m is the magnetic moment of the dipole.
- For the magnetic field B1:
τ1=mB1sin(30∘)
- For the magnetic field B2:
τ2=mB2sin(30∘)
τ1=τ2
mB1sin(30∘)=mB2sin(30∘)
The m and sin(30∘) terms cancel out, leading to:
B1=B2
We know that:
B1=1.2×10−1T
Therefore, we can express B2 in terms of B1:
B2=B1=1.2×10−1T
The problem states that the magnitude of the field B2 can be expressed as x×102T. Thus:
B2=x×102T
Setting the two expressions for B2 equal gives:
x×102=1.2×10−1
Rearranging the equation:
x=1.2×10−1102=1.2×10−3
The value of x is 1.2.
Topper's Solved these Questions
Similar Questions
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1.2×10−1T. If the dipole comes to stable equilibrium at an angle of 30∘ with this field, then the magnitude of the field (in tesla) is x×102T. Find x,
View SolutionA magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘, and one of the fields has a magnitude of 1.2×10−2T. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, what is the magnitude of the other field?
View Solution
Knowledge Check
- Question 1 - Select One
A magnetic dipole is under the influence of two magnetic fields. The angle between the field direction is 60∘ and one of the fields has magnitude of 1.2×10−2T. If the dipole comes to stable equilibrium at an angle of 30∘ with this Held, then the magnitude of the field is
A1.2×10−4TB2.4×10−4TC1.2×10−2TD2.4×10−2T - Question 2 - Select One
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1.2×10−2 T. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, what is the magnitude of the other field?
A3(√3−1)×10−3TB(√3−1)×10−3TC6(√3−1)×10−3TD2(sqrt3-1)xx10^(-3)T - Question 3 - Select One
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1⋅2×10−2 tesla. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, figure, what is the magnitude of the other field?
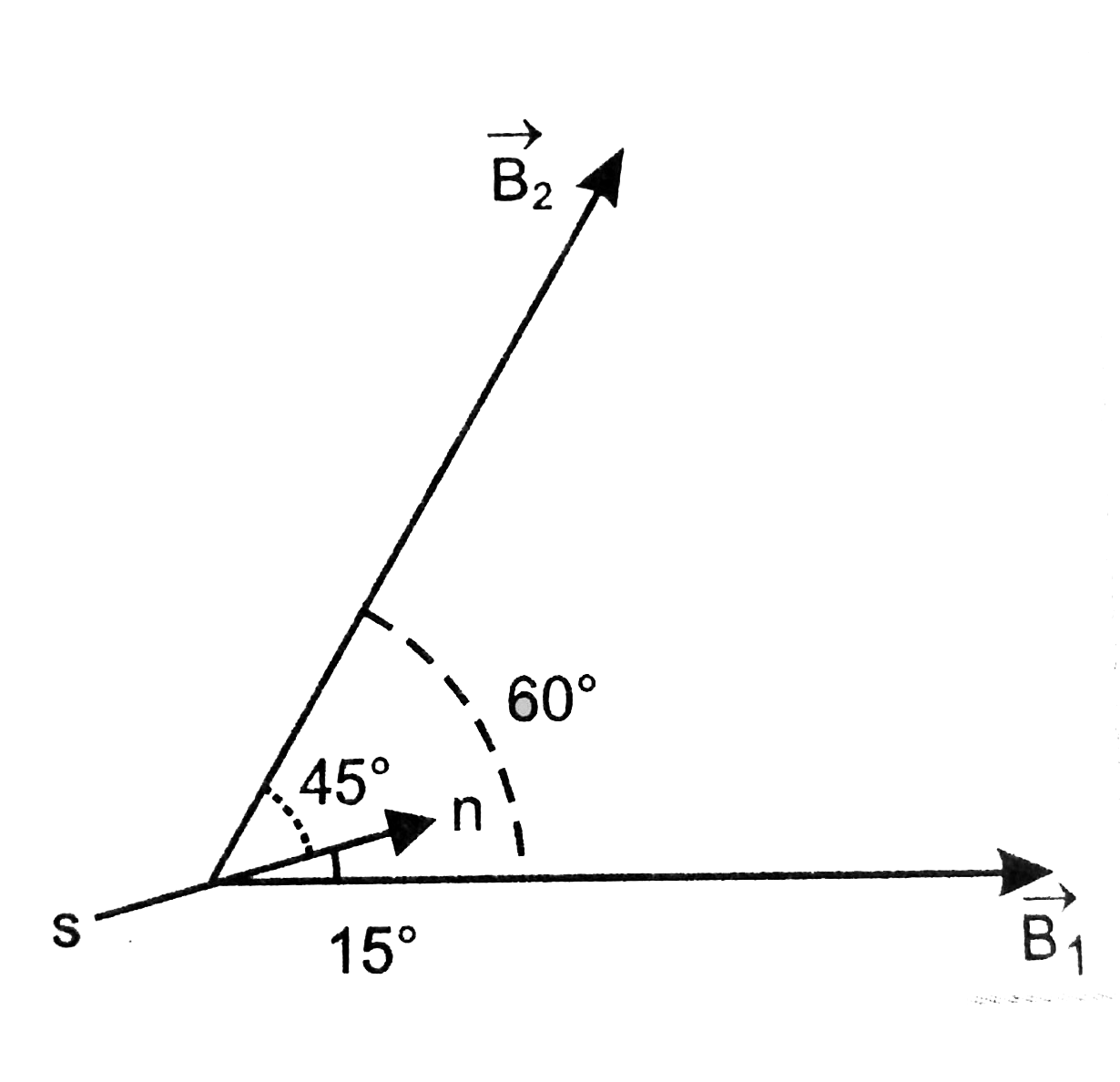 A4.4×10−3B4.4×10−4C4.8×10−3D4.4×103
A4.4×10−3B4.4×10−4C4.8×10−3D4.4×103
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ , and one of the fields has a magnitude of 1.2×10−2 T. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, what is the magnitude of the other field ?
View SolutionA magnetic dipole is under the influence of two magnetic fields. The angle between the two field directions is 60∘ and one of the fields has a magnitude of 1.2×10−2T. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, what is the magnitude of the other field?
View SolutionA magnetic dipole is under the influence of trwo magnetic fields. The angle between the two field directionss is 60∘ and one of the fields has a magnitudeo f1.2×10−2T. Of the dipole comes to stable equilibrium at an angle of 150 with this field, what is the magnitude of the other field?
View SolutionA magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1⋅25×10−2T. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, find the magnitude of other magnetic field.
View SolutionA magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1⋅2×10−2 tesla. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, figure, what is the magnitude of the other field?
 View Solution
View Solution

