A magnetic field in a certain region is given by $B = B_{0} cos (ω t) ˆ k$ and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field, Fig. Find the magnitude and the direction of the current at (a,0,0) $a t t = π / 2 ω, t = π / ω$ and $t = 3 π / 2 ω$ .

Video Solution

Text Solution

Verified by Experts

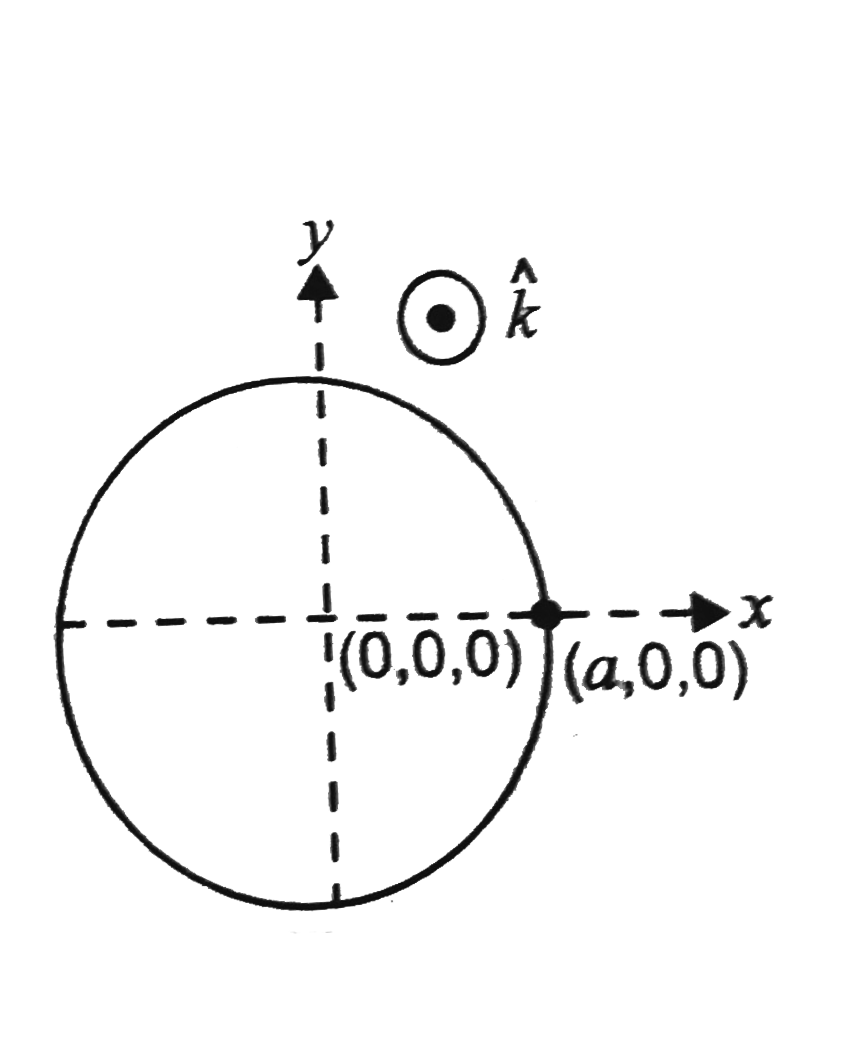
At any instant, flux passes through the ring is given by $ϕ = B . A = B a cos θ = B A$
or $ϕ = B_{0} (π a^{2}) cos ω t$
By Faraday's law of electromagnetic induction, Magnitude of induced emf is given by $ε = B_{0} (π a^{2}) ω sin ω t$
This causes flow of induced current, which is given by
$I = B_{0} (π a^{2}) ω sin ω t / R^{2}$
Now,finding the value of current at different instants, so we have current at
$t = \frac{π}{2 ω}$
$I = \frac{B_{0} (π a^{2}) ω}{R} a l o n g ˆ j$
Because $sin ω t = sin (ω \frac{π}{2 ω}) = sin, \frac{π}{2} = 1$
$= \frac{π}{ω} I = \frac{B (π a^{2}) ω}{R}$
Here, $sin ω t = sin (ω = \frac{π}{ω}) = sin π = 0$
$t = \frac{3}{2} \frac{π}{ω}$
$I = \frac{B (π a^{2}) ω}{R} a l o n g - ˆ j$
$sin ω t = sin (ω \frac{3 π}{2 ω}) = sin, \frac{3 π}{2} = 1$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

NCERT EXEMPLAR ENGLISH-ELECTROMAGNETIC INDUCTION -Short answer type questions

Home
Ask Doubt
Profile