A man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant , the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘ (θ is known as 'Parallax') estimate the distance of the tower C from his original position A.
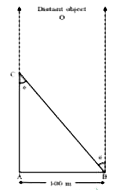
We have, parallax angle θ=40∘ From fig.2.3. AB=AC tanθ
AC=AB/tanθ = 100m/tan40∘=100m/0.8391=119
Topper's Solved these Questions
- UNITS AND MEASUREMENTBOOK - NCERT BANGLISHCHAPTER - UNITS AND MEASUREMENTEXERCISE - Exercise (Fill in the blanks)
8 Videos
- UNITS AND MEASUREMENTBOOK - NCERT BANGLISHCHAPTER - UNITS AND MEASUREMENTEXERCISE - EXERCISE
23 Videos
- THERMODYNAMICS BOOK - NCERT BANGLISHCHAPTER - THERMODYNAMICS EXERCISE - EXERCISES
7 Videos
- WAVESBOOK - NCERT BANGLISHCHAPTER - WAVESEXERCISE - Exercises
26 Videos
Similar Questions
A man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicualr to AC upto B, a distaance of 100m and looks at O and C again. Since O is very distant, the direction of BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘(θis known as parallax). Estimate the distance fo the tower C from his original position A.
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicualr to AC upto B, a distaance of 100m and looks at O and C again. Since O is very distant, the direction of BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘(θ is known as parallax). Estimate the distance fo the tower C from his original position A.
View Solution
Knowledge Check
The angles of elevation of e top of an inaccessible tower from two points on the same straight line from the base of the tower are 30∘and60∘, respectively. If the points are separated at a distance of 100 m, then the height of the tower is close to
A86.5mB80.6mC82.6mD80.6mThe angle of elevation of the top of a tower from the point P and Q at distance of a and b respectively from the base of the tower and in the same straight line with it are complementary .The height of the tower is
A√abBabCabDa2b2A person standing at the foot of a tower walks a distance of 3 meters from the tower and observes that the angle of elevation of the top of the tower is 30∘. He then walks a distance 4 meters perpendicular to the previous direction and observes the angle of elevation to be β. Then, cos2β is equal to
A√32B1√3C2√3D1114
The angles of elevation of the top of an inaccessible tower from two points on the same straight line from the base of the tower are 30° and 60° respectively. If the points are separated at a distance of 100 m, then the height of the tower is close to :
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
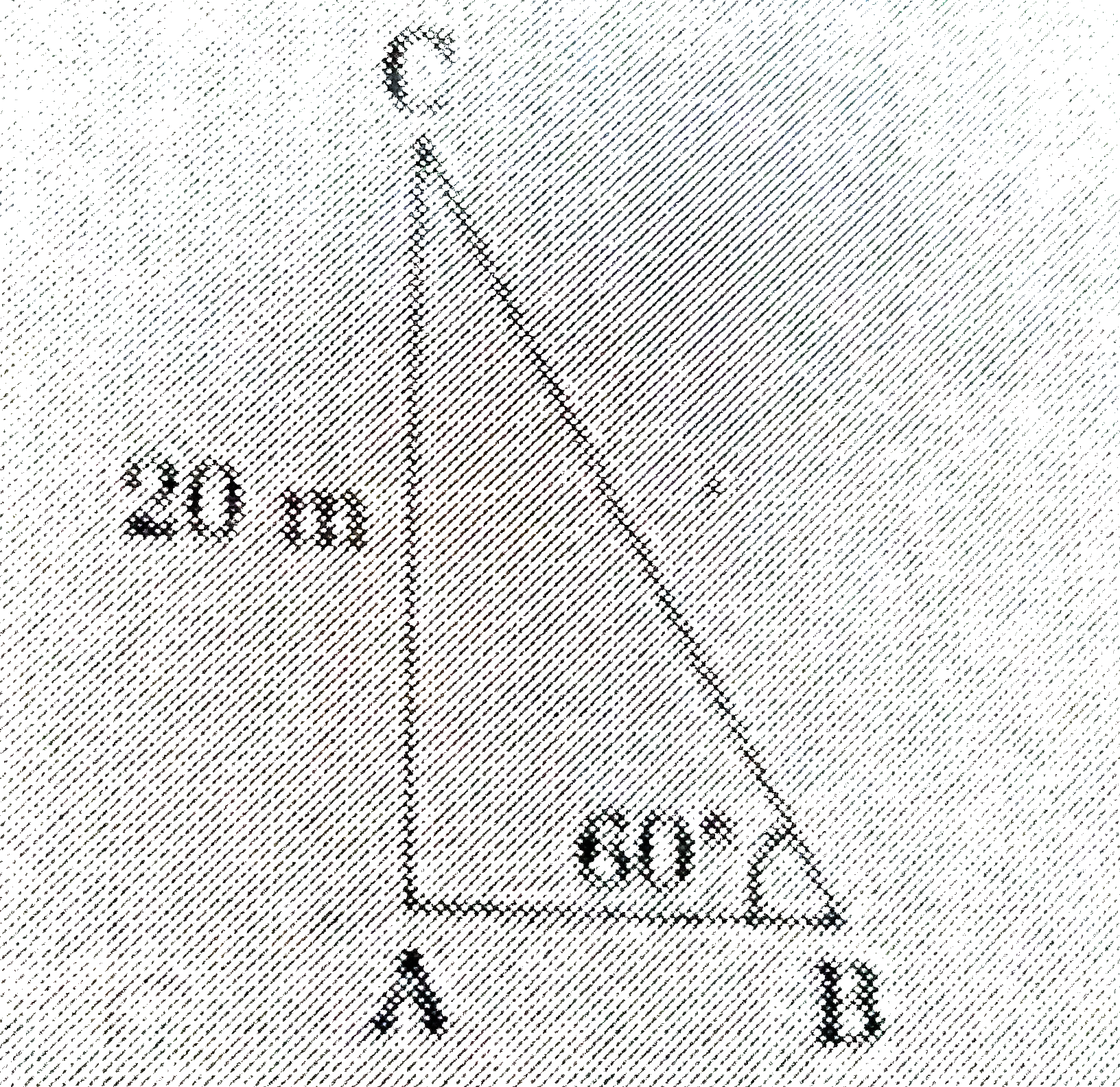
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
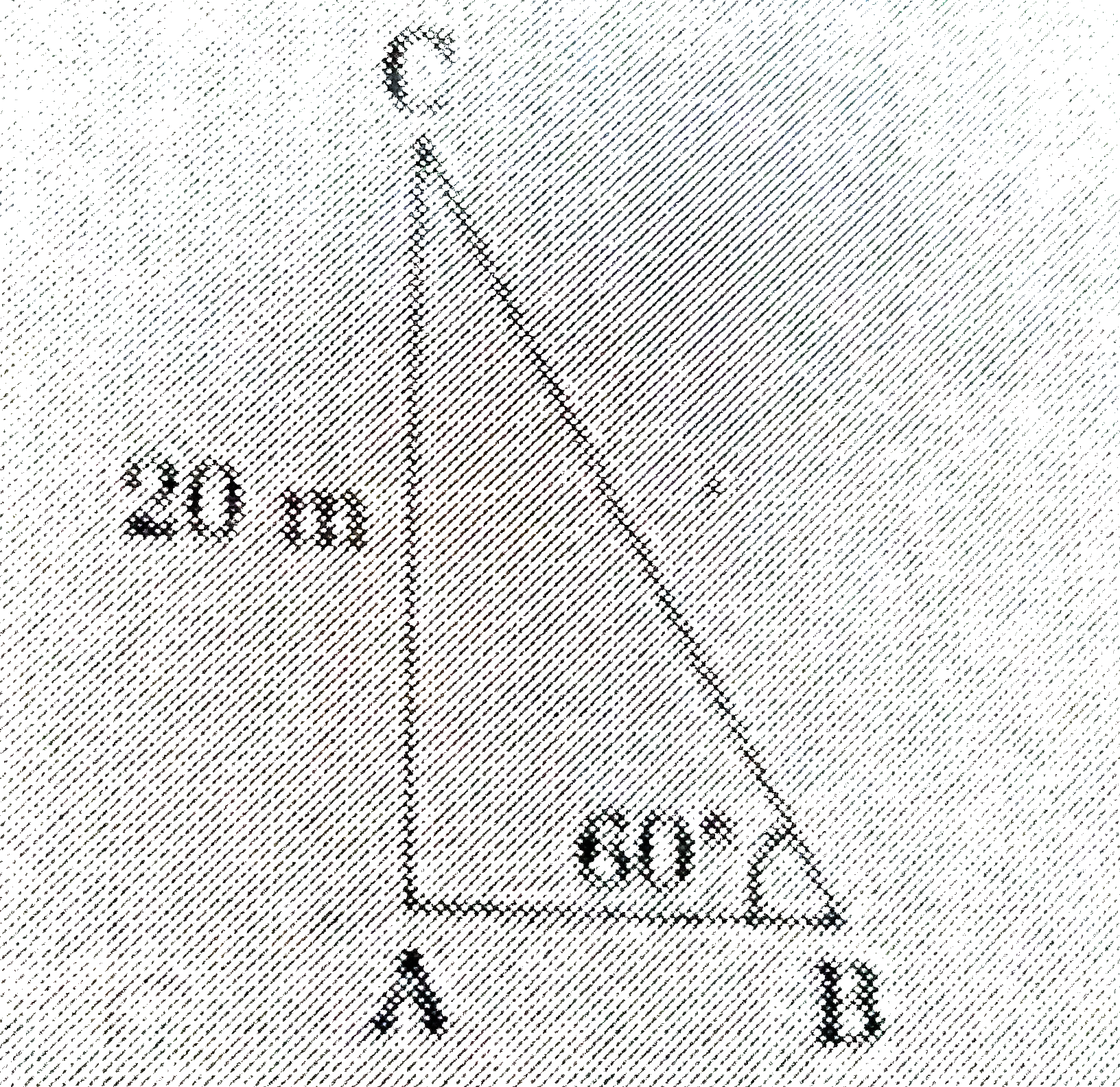
View SolutionA vertical tower is 20 m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower ?
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View Solution
