A mass of 10 kg is suspended using two strings. One string makes an angle of with the vertical. What should be the angle made by the other string so that the tension in the string will be minimum? Find the tension in each wire.
As the mass is in equilibrium, from the force diagram Fig. we get,
or,
Also
From (2) and (1) we get,
or, mg
As m, g and are constants the minimum value of corresponds to the maximum value of sin i.e., sin
From equation (3) we get,
N
From equation (2) we get,
N.

Topper's Solved these Questions
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
18 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXERCISE (Multiple Choice Questions )
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER
16 Videos
- SUPERPOSITION OF WAVESBOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner
14 Videos
Similar Questions
A mass of 10 kg is suspended from a spring balance. It is pulled aside by a horizontal string so that it makes an angle of 60∘ with the vertical. The new rading of the balance is
View SolutionA body of mass m is suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
View Solution
Knowledge Check
A mass of 10 kg is suspended from a spring balance. It is pulled aside by a horizontal string so that it makes an angle of 60∘ with the vertical. The new rading of the balance is
A20 kg-wtB10 kg-wtC10√3 kg-wtD20√3 kg-wtA ball of mass 0.1 kg is suspended by a string. It is displaced through an angle of 60∘ and left. When the ball passes through the mean position, the tension in the string is
A19.6NB1.96NC9.8NDZeroA mass of 10 kg is suspended from a spring balance, it is pulled aside by a horizontal string so that it makes an angle 60∘ with the vertical. The new reading of the balance is:
A10√3 kg wtB20√3 kg wtC20 kg wtD10 kg wt
For the arrangement shown in figure, angle θ for which tension T1 in the left string will be minimum is (Pulley and strings are ideal)
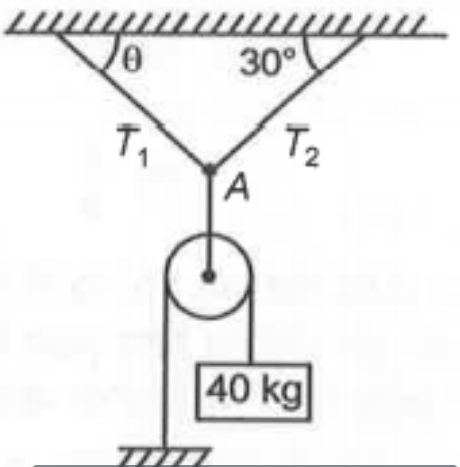
View SolutionFor the arrangement shown in figure, angle θ for which tension T1 in the left string will be minimum is (Pulley and strings are ideal)

View SolutionA body of mass mis suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.

View SolutionA body of mass √3 kg is suspended by a string to rigid support.The body is pulled horizontally by a force F until the string makes an angle of 30∘ with the vertical.The value of F and tension in the string are
View Solution
