A Merry -go-round, made of a ring-like plarfrom of radius RandmassM, is revolving with angular speed ω. A person of mass M is standing on it. At one instant, the person jumps off the round, radially awaay from the centre of the round (as see from the round). The speed of the round after wards is
2ω
ω
ω/2
0
The correct Answer is:b
To solve the problem, we will apply the principle of conservation of angular momentum. Here’s the step-by-step solution:
Step 1: Understand the System
We have a merry-go-round (ring-like platform) with:
- Mass of the platform (ring) = M
- Radius of the platform = R
- Initial angular speed = ω
A person of mass M is standing on the platform and jumps off radially outward.
Step 2: Initial Angular Momentum
The total initial angular momentum Li of the system (merry-go-round + person) can be calculated as follows:
- Moment of inertia of the ring about its vertical axis:
Iring=MR2
- Moment of inertia of the person (considered as a point mass) at distance R from the center:
Iperson=MR2
- Therefore, the total initial moment of inertia Ii is:
Ii=Iring+Iperson=MR2+MR2=2MR2
- The initial angular momentum Li is:
Li=Ii⋅ω=(2MR2)⋅ω=2MR2ω
Step 3: Final Angular Momentum
After the person jumps off, only the ring is rotating. The moment of inertia of the ring remains the same, but the person no longer contributes to the moment of inertia:
- The final moment of inertia If is:
If=MR2
- Let the final angular speed be ωf. The final angular momentum Lf is:
Lf=If⋅ωf=(MR2)⋅ωf
Step 4: Apply Conservation of Angular Momentum
According to the conservation of angular momentum:
Li=Lf
Substituting the expressions we derived:
2MR2ω=MR2ωf
Step 5: Solve for Final Angular Speed
We can simplify this equation:
2ω=ωf
Thus, we find:
ωf=2ω
Conclusion
The speed of the merry-go-round after the person jumps off is 2ω.
We have a merry-go-round (ring-like platform) with:
- Mass of the platform (ring) = M
- Radius of the platform = R
- Initial angular speed = ω
The total initial angular momentum Li of the system (merry-go-round + person) can be calculated as follows:
- Moment of inertia of the ring about its vertical axis:
Iring=MR2
- Moment of inertia of the person (considered as a point mass) at distance R from the center:
Iperson=MR2
- Therefore, the total initial moment of inertia Ii is:
Ii=Iring+Iperson=MR2+MR2=2MR2
- The initial angular momentum Li is:
Li=Ii⋅ω=(2MR2)⋅ω=2MR2ω
After the person jumps off, only the ring is rotating. The moment of inertia of the ring remains the same, but the person no longer contributes to the moment of inertia:
- The final moment of inertia If is:
If=MR2
- Let the final angular speed be ωf. The final angular momentum Lf is:
Lf=If⋅ωf=(MR2)⋅ωf
According to the conservation of angular momentum:
Li=Lf
Substituting the expressions we derived:
2MR2ω=MR2ωf
We can simplify this equation:
2ω=ωf
Thus, we find:
ωf=2ω
The speed of the merry-go-round after the person jumps off is 2ω.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - ASSERTION AND REASON
15 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Kinematics Of Rotational Motion About A Fixed Axis
3 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - HOTS HIGHER ORDER THINKING SKILL
8 Videos
- PRACTICE PAPERSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPERSEXERCISE - All Questions
150 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
Similar Questions
A disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionConsidering a body of mass m, radius R rotating with angular speed ω about the centre of the mass and with a velocity v of centre of mass, the most appropriate definition of rolling motion will be
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with an angular speed ω0 two particles each of mass m are now attached at diametrically opposite points. Find new angular speed of the ring.
View SolutionA thin circular ring of mass M and radius r is rotating about its axis with an angular speed ω. Two particles having mass m each are now attached at diametrically opposite points. The angular speed of the ring will become
View SolutionA thin circular ring of mass M and radius r is rotating about its axis with an angular speed ω. Two particles having mass m each are now attached at diametrically opposite points. The angular speed of the ring will become
View SolutionA uniform brass disc of radius a and mass m is set into spinning with angular speed ω0 about an axis passing through centre of disc and perpendicular to the palne of disc. If its temperature increases from θ∘1C to θ∘2C with out disturbing the disc, what will be its new angular speed ?
View SolutionA satellite of mass m is in a circular orbit of radius r round the Earth. Calculate its angular momentum with respect to the centre of the orbit in terms of the mass M of the Earth and G.
View SolutionA cord is wound round the circumference of a solid cylinder radius R and mass M. The axis of the cylinder is horizontal. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h.
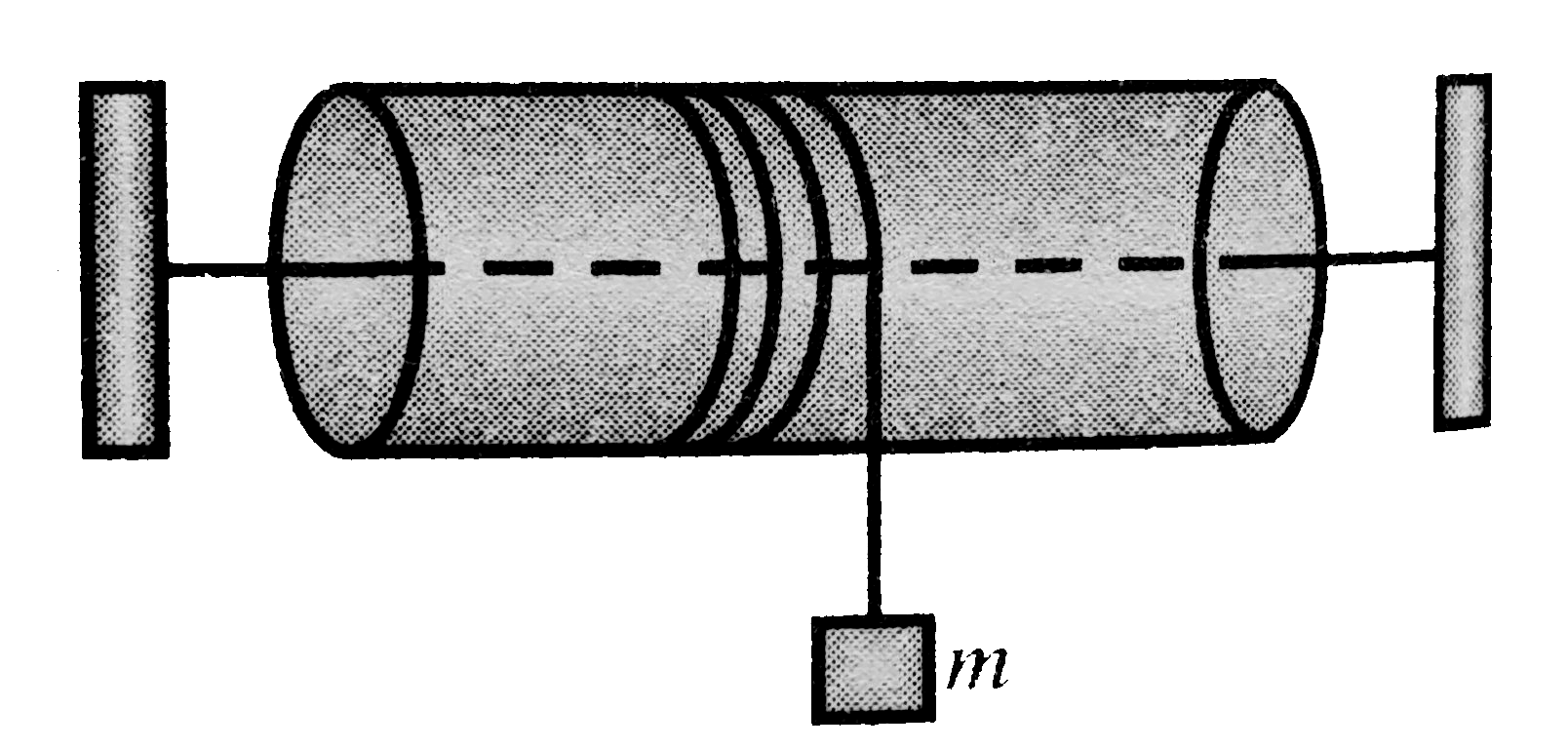
If the mass starts from rest and falls a distance h, then its speed at that instant is:
View SolutionA uniform round body of radius R and mass m and its moment of inertia about centre of mass O is Icm is given. A force F is applied at a height h above the centre of the round body. Find the height h at which force should be applied so that it rolls without friction.
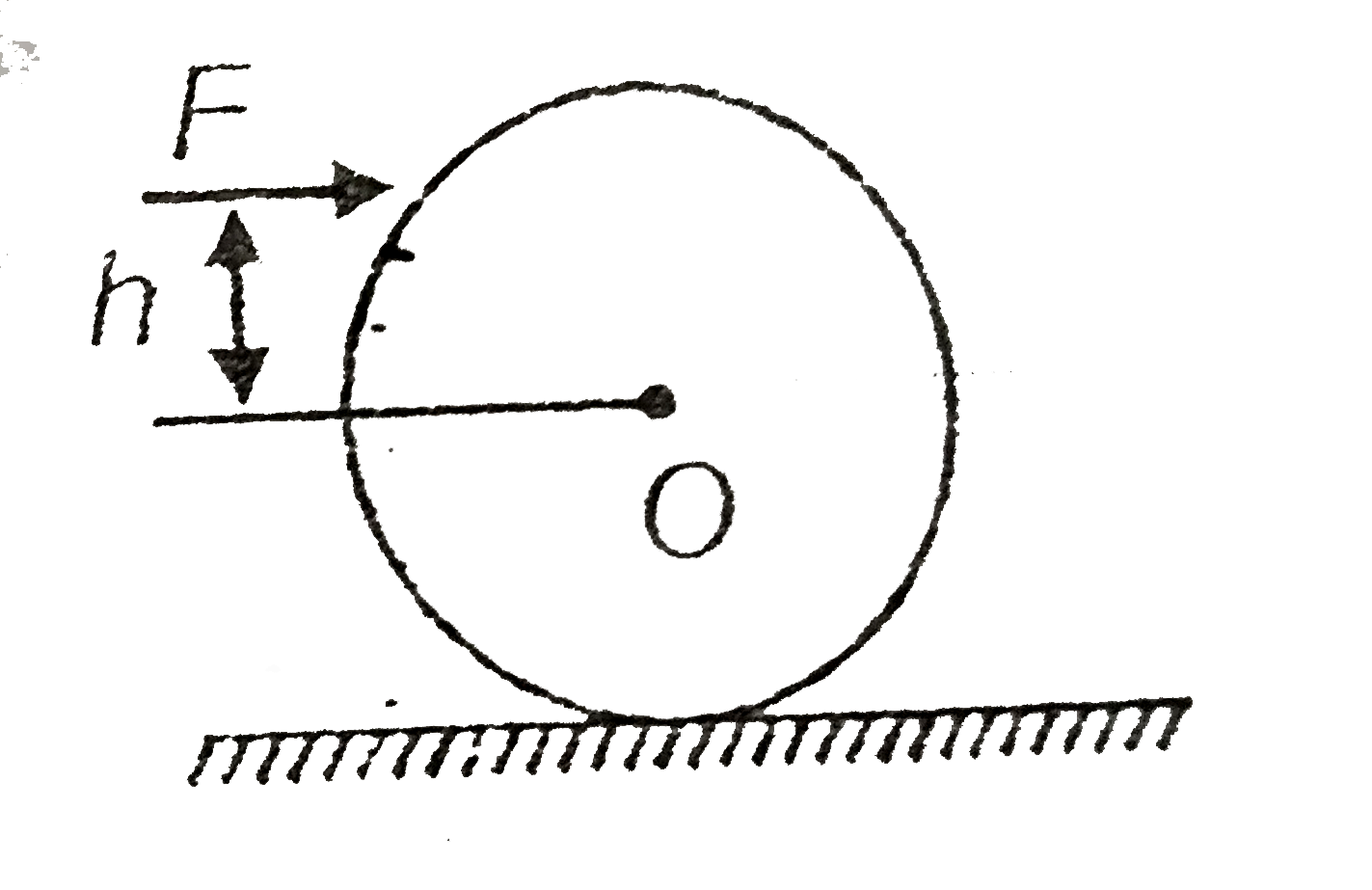
View Solution
