a metal solid cylinder tied ot a thread is hanging from the hook of a spring balance. The cylinder is gradually immersed into water contaned in a jar. What changes do you expect in the readings of spring balance? Explain your answer.
To solve the question regarding the changes in the readings of a spring balance when a metal solid cylinder is gradually immersed in water, we can break it down into the following steps:
1. Understanding the Initial Condition:
- When the metal cylinder is hanging in air, the spring balance measures the actual weight of the cylinder. This weight (W_A) can be expressed as:
WA=m⋅g
where m is the mass of the cylinder and g is the acceleration due to gravity.
2. Immersion into Water:
- As the cylinder is gradually immersed into the water, it experiences an upward force known as the buoyant force (F_B). According to Archimedes' principle, this buoyant force is equal to the weight of the water displaced by the submerged part of the cylinder.
3. Calculating the Net Weight in Water:
- When the cylinder is fully immersed in water, the reading on the spring balance (W_B) will be affected by this buoyant force. The net weight of the cylinder when submerged can be calculated as:
WB=WA−FB
- Substituting the expression for WA:
WB=m⋅g−FB
4. Observing the Change in Reading:
- Since the buoyant force acts in the opposite direction to the weight of the cylinder, the reading on the spring balance will decrease as the cylinder is submerged. The more the cylinder is submerged, the greater the buoyant force, leading to a further decrease in the reading.
5. Conclusion:
- Therefore, as the metal solid cylinder is gradually immersed into the water, the reading on the spring balance will decrease. This decrease continues until the cylinder is fully submerged, at which point the spring balance will show a weight that is less than the weight of the cylinder in air.
Summary:
- The reading on the spring balance decreases as the cylinder is immersed in water due to the upward buoyant force acting against the weight of the cylinder.
- When the metal cylinder is hanging in air, the spring balance measures the actual weight of the cylinder. This weight (W_A) can be expressed as:
WA=m⋅g
where m is the mass of the cylinder and g is the acceleration due to gravity.
- As the cylinder is gradually immersed into the water, it experiences an upward force known as the buoyant force (F_B). According to Archimedes' principle, this buoyant force is equal to the weight of the water displaced by the submerged part of the cylinder.
- When the cylinder is fully immersed in water, the reading on the spring balance (W_B) will be affected by this buoyant force. The net weight of the cylinder when submerged can be calculated as:
WB=WA−FB
- Substituting the expression for WA:
WB=m⋅g−FB
- Since the buoyant force acts in the opposite direction to the weight of the cylinder, the reading on the spring balance will decrease as the cylinder is submerged. The more the cylinder is submerged, the greater the buoyant force, leading to a further decrease in the reading.
- Therefore, as the metal solid cylinder is gradually immersed into the water, the reading on the spring balance will decrease. This decrease continues until the cylinder is fully submerged, at which point the spring balance will show a weight that is less than the weight of the cylinder in air.
- The reading on the spring balance decreases as the cylinder is immersed in water due to the upward buoyant force acting against the weight of the cylinder.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A) (Multiple Choice Question)3videos
UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATION
- BOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A) (Numerical)10videos
UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATION
- BOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(c) (Numerical)9videos
UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATION
- BOOK - ICSECHAPTER - SOUNDEXERCISE - TOPIC 2 Infrasonic and Ultrasonic Waves ( 3 Marks Questions)3videos
SOUND
Similar Questions
When a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water. What reading do you expect on the scale of spring balance?
View SolutionWhen a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water.What reading do you expect on the scale of spring balance?
View SolutionA block hangs from a spring balance. The balance reads 40 N in air, 35 N when block is immersed in water and 25 N when block is immersed in another liquid. Find the density of block and other liquid.
View SolutionA light spring balance hangs from the hook of the other light spring balance and a block of mass M kg hangs from the former one. Then the true statement about the scale reading is
View SolutionA light spring balance hangs from the hook of the other light spring balance and a block of mass M kg hangs from the former one. Then the true statement about the scale reading is
View SolutionA 20N metal block is suspended by a spring balance. A beaker containing some water is placed on a weighing machine which reads 40N. The spring balance is now lowered so that the block gets immersed in the water. The spring balance now reads 16N. The reading of the weighing machine will be.
View SolutionAs Fig. shows, S1 and S2, are spring balances. Block A is hanging from spring balance S1 and immersed in liquid L which is contained in beaker B. The mass of beaker B is 1kg and mass of liquid L is 1.5kg. Balances S1 and S2 reads 2.5kg and 7.5kg, respectively. What will be the readings of S1 and S2 when block A is pulled up out of the liquid. Find the reading of S1 and S_(2)?
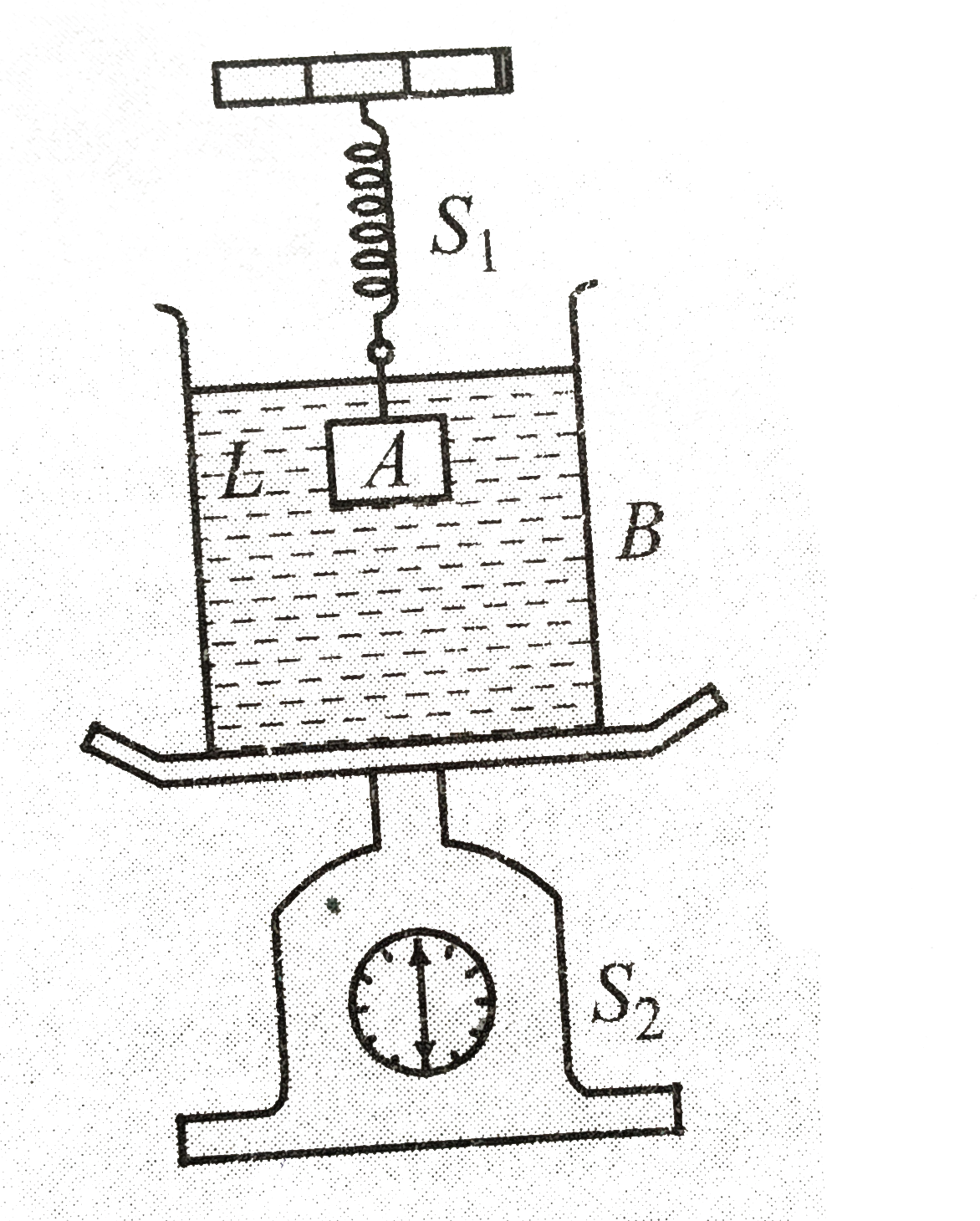 View Solution
View SolutionThe reading of a spring balance when a block is suspended from it in air is 60 N. This reading is changed to 40 N when the block is submerged in water. The relative density of the block is:
View SolutionThe reading of a pring balance when a block is suspended from it in air is 60 N. This reading is change to 40 N when the block is submerged in water. The specific gravity of the block must be therefore
View SolutionA beaker containing water is placed on the platform of a spring balance. The balance reads 1.5kg. A stone of mass 0.5kg and density 104kg/m3 is immersed in water without touching the walls of the beaker. What will be the balance reading now?
View Solution

