A metallic rod of length l is moved perpendicular to its length with velocity v in a magnetic field →B acting perpendicular to the plane in which rod moves. Derive the expression for the inducced emf.
To derive the expression for the induced electromotive force (emf) in a metallic rod moving in a magnetic field, we can follow these steps:
Step 1: Understand the Setup
We have a metallic rod of length L moving with a velocity v perpendicular to its length in a magnetic field →B that is also perpendicular to the plane of motion of the rod.
Step 2: Determine the Change in Area
As the rod moves, the area associated with the magnetic flux changes. The change in area dA as the rod moves a small distance dx can be expressed as:
dA=L⋅dx
Step 3: Calculate the Change in Magnetic Flux
The magnetic flux Φ through the area A is given by:
Φ=→B⋅→A=B⋅A⋅cos(θ)
Since the magnetic field →B is perpendicular to the area vector (which is also perpendicular to the motion of the rod), the angle θ=0∘, and thus cos(0)=1. Therefore, the change in magnetic flux dΦ due to the change in area is:
dΦ=B⋅dA=B⋅(L⋅dx)
Step 4: Apply Faraday's Law of Electromagnetic Induction
According to Faraday's law, the induced emf E is given by the negative rate of change of magnetic flux:
E=−dΦdt
Substituting the expression for dΦ:
E=−d(B⋅L⋅dx)dt
Step 5: Relate dx to Time
The term dxdt represents the velocity v of the rod. Therefore, we can rewrite the equation:
E=−B⋅L⋅dxdt=−B⋅L⋅v
Step 6: Final Expression for Induced EMF
The magnitude of the induced emf is given by:
|E|=B⋅L⋅v
Thus, the expression for the induced emf in the rod moving in a magnetic field is:
E=B⋅L⋅v
We have a metallic rod of length L moving with a velocity v perpendicular to its length in a magnetic field →B that is also perpendicular to the plane of motion of the rod.
As the rod moves, the area associated with the magnetic flux changes. The change in area dA as the rod moves a small distance dx can be expressed as:
dA=L⋅dx
The magnetic flux Φ through the area A is given by:
Φ=→B⋅→A=B⋅A⋅cos(θ)
Since the magnetic field →B is perpendicular to the area vector (which is also perpendicular to the motion of the rod), the angle θ=0∘, and thus cos(0)=1. Therefore, the change in magnetic flux dΦ due to the change in area is:
dΦ=B⋅dA=B⋅(L⋅dx)
According to Faraday's law, the induced emf E is given by the negative rate of change of magnetic flux:
E=−dΦdt
Substituting the expression for dΦ:
E=−d(B⋅L⋅dx)dt
The term dxdt represents the velocity v of the rod. Therefore, we can rewrite the equation:
E=−B⋅L⋅dxdt=−B⋅L⋅v
The magnitude of the induced emf is given by:
|E|=B⋅L⋅v
Thus, the expression for the induced emf in the rod moving in a magnetic field is:
E=B⋅L⋅v
Similar Questions
A small conducting rod of length l, moves with a uniform velocity v in a uniform magnetic field B as shown in fig __
 View Solution
View Solution(a) A rod of length l is moved horizontal with a uniform velocity v in a direction perendicular to its length through a region in which a uniform magnetic field is acting vertically downward. Derive the expression for the emf induced across the end of the rod.
(b) How does one understand this motional emf by invoking the Lorentz force acting on the free charge carriers of the conductor? Explain.View SolutionA glass rod of length l moves with velocity v in a uniform magnetic field B. What is the e.m.f induced in the rod?
View SolutionA metallic square loop ABCD is moving in its own plane with velocity v in a uniform magnetic field perpendicular to its plane as shown in the figure. An electric field is induced
 View Solution
View SolutionA metallic square loop ABCD is moving in its own plane with velocity v in a uniform magnetic field perpendicular to its plane as shown in the figure. An electric field is induced
 View Solution
View SolutionA conducting rod AB of length l is rotated about point O with an angular velocity velocity omega in a uniform magnetic field (B_0) directed perpendicular into the plane of rotation as shown in the figure. The value of VB−VA is
View SolutionA metalic square loop ABCD is moving in its own plane with velocity v is in a uniform magnetic field perpendicular to its plane as shown in the figure . An electric field is induced
 View Solution
View SolutionA conducting rod OA of length l is rotated about its end O with an angular velocity ω in a uniform magnetic field directed perpendicualr to the rotation. Find the emf induced in the rod, between it's ends.
View SolutionRod PQ of length 2l is rotating about one end P in a uniform magnetic field B which is perpendicular to the plane of rotation of the rod . Point M is the mid- point of the rod. Find the induced emf between M and Q if the potential between P and Q is 100V.
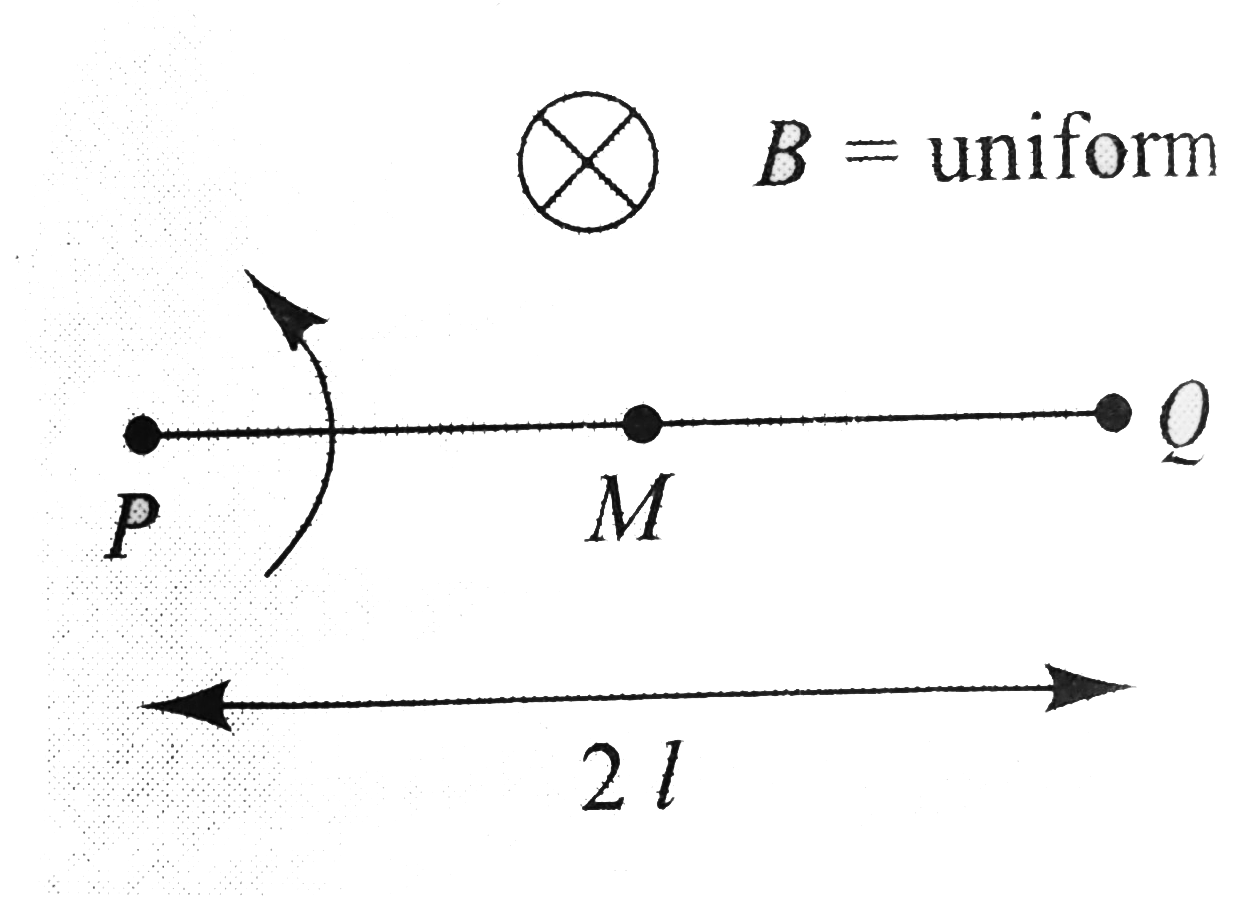 View Solution
View SolutionRod PQ of length 2l is rotating about one end P in a uniform magnetic field B which is perpendicular to the plane of rotation of the rod . Point M is the mid- point of the rod. Find the induced emf between M and Q if the potential between P and Q is 100V.
 View Solution
View Solution

