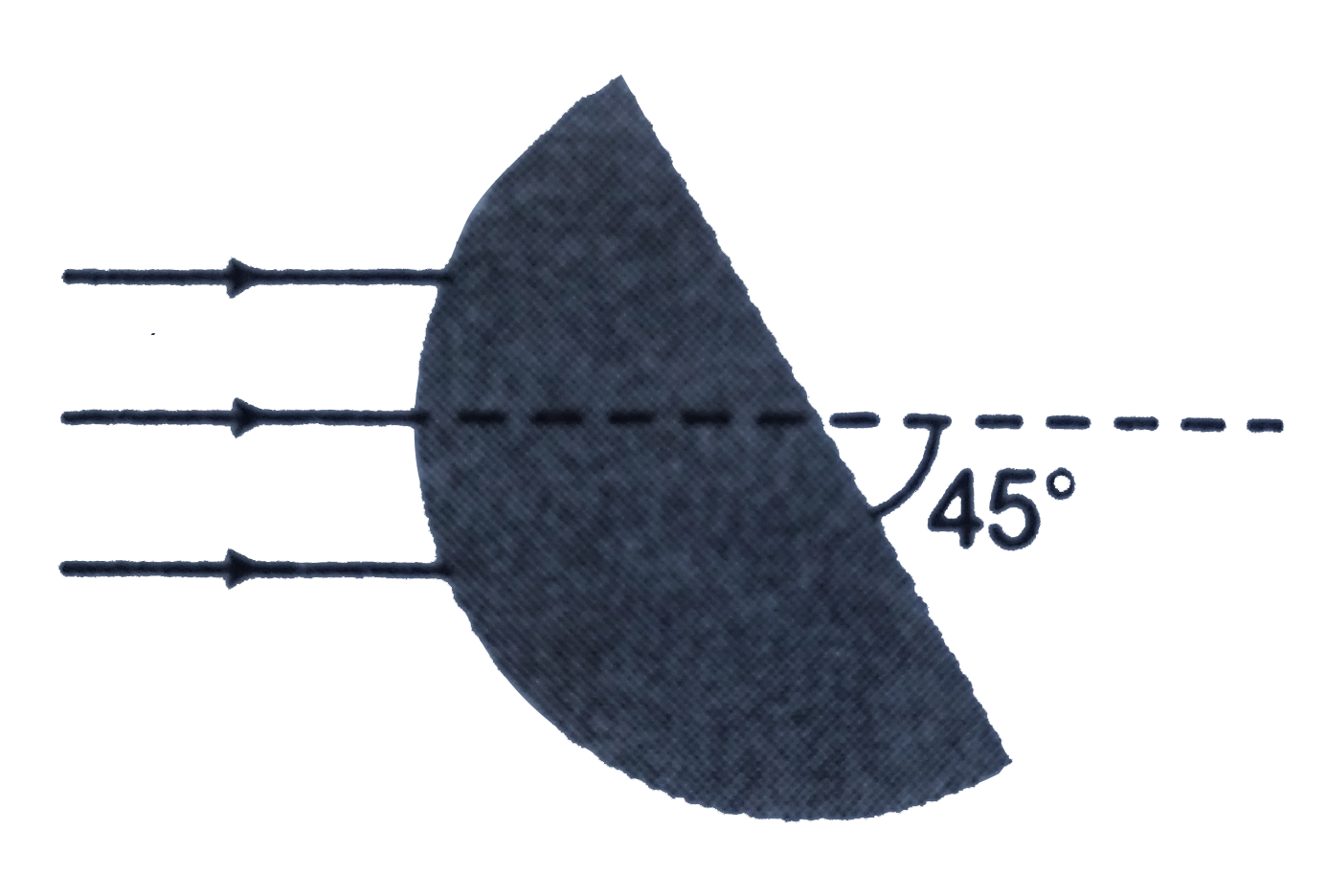
A narrow beam of natural light falls on the surface of a thick transparent plane-parallel plate at the Brewster angle. Its top surface. Find the degree of polarization of beams 1−4 (Fig.)

Since natural light is incident at the Brewster's angle, the reflected light 1 is complately polarized and P1=1.
Similarly the ray 2 is incident on glass air surface at Brewster's angle (tan−1.1n) so 3 is also completely polarized. Thus P3=1
Now as in 5.167(b)
P2=ρ1−ρ=0.087 if ρ=0.080


Finally as shown in the figure
P4=12−12(1−2ρ)212+12(1−2ρ)2=2ρ(1−ρ)1−2ρ(1−ρ)=0.173
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Dispersion And Absorption Of Light24videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources22videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Diffraction Of Light60videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems92videos
MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
Similar Questions
A narrow beam of natural light falls on a set of N thick plane-parallel glass plates at the Brewster angle. Find:
(a) the degree P of polarization of the transmitted beam,
(b) what P is equal to when N=1,2,5, and 10.View SolutionA beam of natural light falls on the surface of glass at an angle of 45∘. Using the Fresnel equations, find the degree of polarization of
(a) reflected light,
(b) refracted light.View Solution
Knowledge Check
- Question 1 - Select One
A parallel narrow beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index μ=1.5 as shown. The position of the image formed by refraction at the image formed by refraction at the spherical surface only as
 AR2B3RCR3D2R
AR2B3RCR3D2R - Question 2 - Select One
An unpolarized light beam is incident on a surface at an angle of incidence equal to Brewster's angle. Then,
Athe reflected and the refracted beam are both partially polarizedBthe reflected beam is partially polarized and the refracted beam is completely polarized and are at right angles to each otherCthe reflected beam is completely polarized and the refracted beam is partially polarized and are at right angles to each otherDboth the reflected and the refracted beams are completely polarized and are at right angles to each other. - Question 3 - Select One
An upolarized light beam is incident on a surface at an angle of incidence equal to Brewster's angle. Then,
Athe reflected and the refracted beam are both partially polarizedBthe reflected beam is partially polarized and the refracted beam is completely polarized and are at right angles to each otherCthe reflected beam is completely polarized and the refracted beam is partially polarized and are at right angles to each otherDboth the reflected and the refracted beams are completely polarized and are at right angles to each other.
A plane beam of natural light with intensity I0 falls on the surface of water at the Brewster angle. A fraction ρ=0.039 of luminous flux is reflected. Find the intensity of the refracted beam.
View SolutionNatural light falls at the Brewster angle on the surfcae of glass. Using the Fresnel equations, find
(a) the reflection coefficient,
(b) the degree of polarization of refracted light.View SolutionTwo identical imperfect polarizers are placed in the way of a natural beam of light. When the polarizers' planes are parallel, the system transmits η=10.0 times more than in the case of crossed planes. Find the dergee of polarization of light produced
(a) by each polarizer separately,
(b) by the whole system when the planes of the polarizers are parallel.View SolutionA beam of plane-polarized light falls on the surface of water at the Brewster angle. The polarization plane of the electric vector of the electromagnetic wave makes an angle φ=45∘ with the incidence plane. Find the reflection coefficient.
View SolutionA parallel narrow beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index μ=1.5 as shown. The position of the image formed by refraction at the image formed by refraction at the spherical surface only as
 View Solution
View Solution

