A narrow beam of natural light with wavelength $λ = 589 n m$ falls normally on the surface of a Wollaston polarizing prism made of Iceland spar as shown in Fig. The optical axes of the two parts of the prism are mutually perpendicualr. Find the angle $δ$ between the directions of the beams behind the prism if the angle $θ$ is equal to $30^{\circ}$ .

Text Solution

Verified by Experts

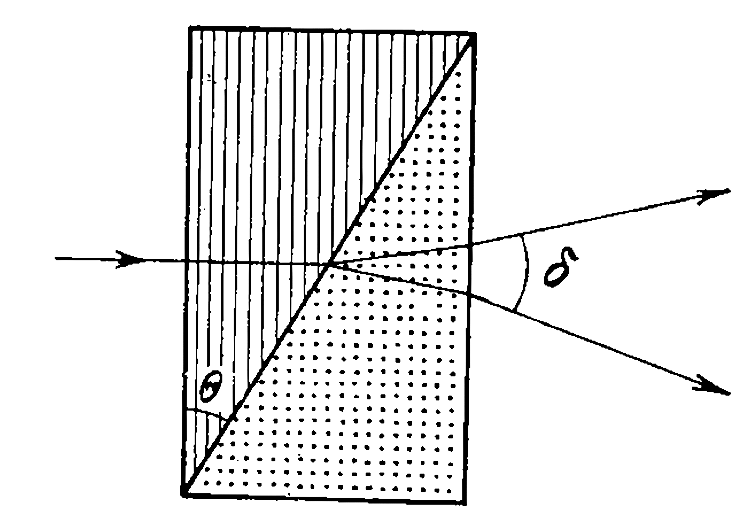
In a uniaxial crystal, an unpolarized beam of light (or even a polarized one) splits up into $O$ (for ordinary) and $E$ (for extraordianary) light waves. The direction of vibration in the $O$ and $E$ waves are most easily spectfied in terms of the $O$ and $E$ principle planes. The principle plane of the ordianry wave is defined as the plane containing the $O$ ray and the optic. Similarly the principle plane of the $E$ wave is the plane contaning the $E$ ray and the optic axis. In terms of these plane the following is true: The $O$ vibrations are perpendicualr to the principle plane of the $O$ ray while the $E$ vibrations are in the principle plane of the $E$ ray.
When we apply this definition to the wollaston prism we find the following: (exaggerated.)
When unpolarized light enters from the left the $O$ and $E$ waves travel in the same direction but with different speeds. The $O$ ray on the left its vibrations normal to the plane of the paper and it becomes $E$ ray on crossing the diagonal boundary of the two prism similarly the $E$ ray on the left becomes $O$ ray on the right. In this case Snell's law is applicable only approxilately. The two rays are incident on the boundary at an angle $θ$ and in the right prism the ray which we have called $O$ ray on the right emerges at
${sin}^{- 1} . \frac{n_{e}}{n_{0}} sin θ = {sin}^{- 1} . \frac{1.658}{1.486} \times \frac{1}{2} = {33.91}^{\circ}$
where we have used
$n_{e} = 1.1658, n_{0} = 1.486$ and $θ = 30^{\circ}$ .
Similarly the $E$ ray on the right emerges within the prism at
${sin}^{- 1} . \frac{n_{0}}{n_{e}} sin θ = {26.62}^{\circ}$
This means that the $O$ ray is incident at the bounary between the prism and air at
$33.91 - 30^{\circ} = {3.91}^{\circ}$
and will emerge into with a deviation of
${sin}^{- 1} . n_{0} {sin 3.91}^{\circ}$
$= {sin}^{- 1} . (1.658 {sin 3.91}^{\circ}) = {6.49}^{\circ}$
The $E$ ray will emerge with an oppsite deviation of
${sin}^{- 1} . (n_{e} sin (30^{\circ} - {26.62}^{\circ}))$
$= {sin}^{- 1} . (1.486 {sin 3.38}^{\circ}) = {5.03}^{\circ}$
Hence $δ \approx {6.49}^{\circ} + {5.03}^{\circ} = {11.52}^{\circ}$
This result is accurate to first order in $(n_{e} - n_{0})$ because Snell's law holds when $n_{e} = n_{0}$ .

Updated on:21/07/2023

A beam of monochromatic light of wavelength $λ$ falls normally on a diffraction grating of line spacing d. If $θ$ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of $sin θ$ ?
A $\frac{λ}{d}$
B $\frac{d}{λ}$
C $\frac{2 λ}{d}$
D $\frac{2 d}{λ}$