A non-relativistic enegry particle moves in a transverse uniform magnetic fiel with induction B. Find the time dependence of the particles's kinetic enegry diminshing due to radiation. How soon will its kinetic enegry decrease e-fold? Calculate this time interval for the case (a) of an electron, (b) of a proton.
R=mveB.
Then P=14πε023c3(eV2R)2=14πε023c3(e2BVm)2
=13πε0c3(B2e4m3)T
This is the radiated power so
dTdt=−B2e43πε0m3c3T
Intergrating T=T0e−v/τ
τ=3πε0m3c3B2e4
τ is (1836)3≈1010 times less for an electorn than for a proton so electrons radiate away theri enegry mush fatser in a megnetic field.
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A particle of mass m, charge q and kinetic energy T enters in a transverse uniform magnetic field of induction B. After the 3 s, the kinetic energy of the particle will be
View SolutionA non-reletivistic proton enters a half-space along the normal to the transverse unifrom magnetic field whose induction equals B=1.0T. Find the raatio of the enrgy lost by the proton due to radiation during its motion in the field to its initial kinetic energy.
View Solution
Knowledge Check
A particle of mass m, charge q and kinetic energy T enters in a transverse uniform magnetic field of induction B. After the 3 s, the kinetic energy of the particle will be
A3TB2TCTD4T
A particle of specific charge alpha enters a uniform magnetic field B=−B0ˆk with velocity v=v0ˆi from the origin. Find the time dependence of velocity and position of the particle.

View SolutionA particle of specific charge alpha enters a uniform magnetic field B=−B0ˆk with velocity v=v0ˆi from the origin. Find the time dependence of velocity and position of the particle.

View SolutionA relativistic particle with charge q and rest mass m, moves along a circle of readius r in a uniform magnetic field of induction B. Find:
(a) the modulus of the particl's momentum vector,
(b) the kinetic energy of the particle,
(c) the acceleartion of the particle.
View SolutionA particle of spectfic charge α enters a uniform magnetic field B=−B0ˆk with velocity V=v0ˆi from the origin Find the time dependence of velocity and position of the particle
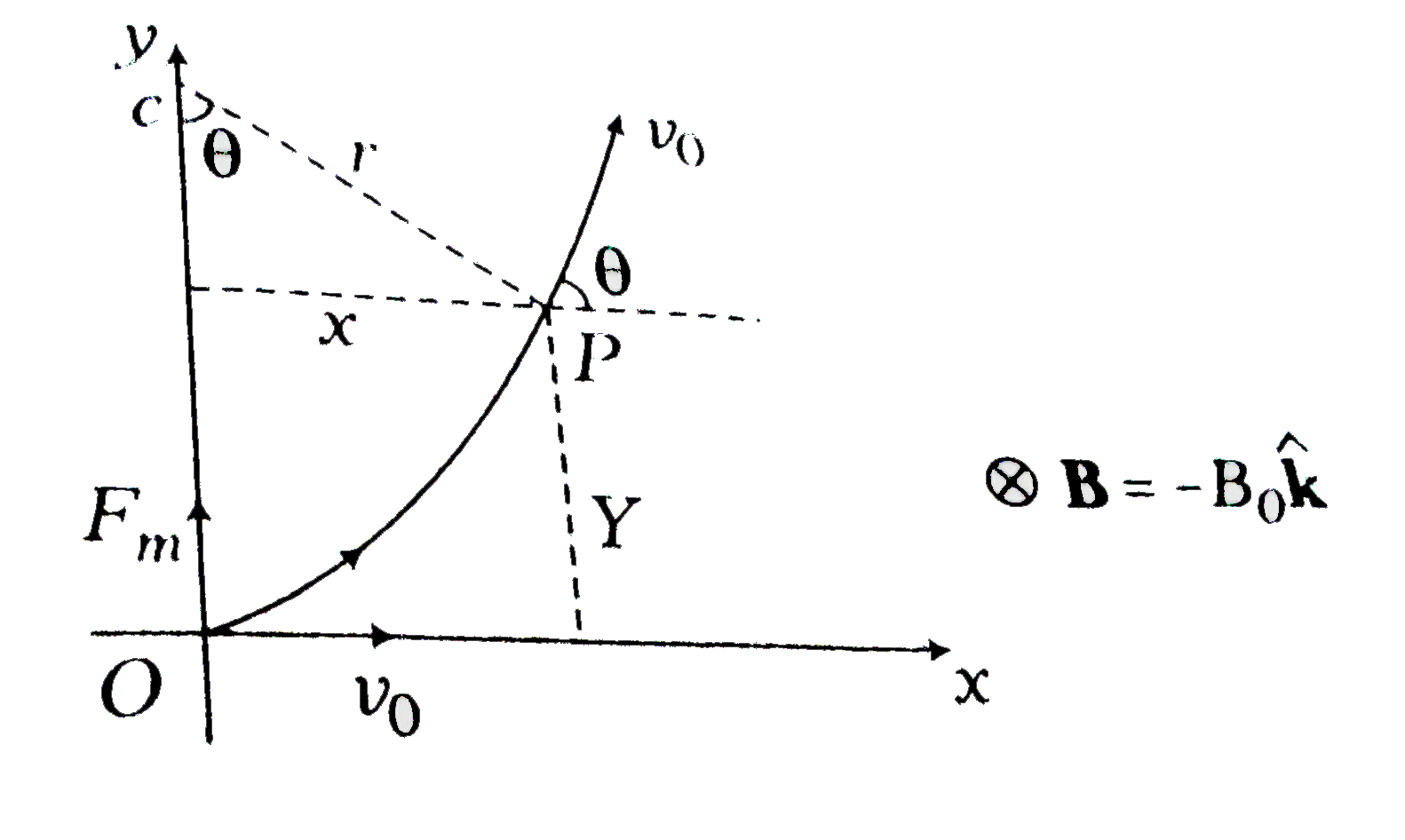
View SolutionA proton and an alpha particle is projected with equal kinetic energy in transverse uniform magnetic field B. The ratio of radii of proton to that of alpha particle is
View SolutionA charged particle moving in a uniform magnetic field penetrates a layer of lead and thereby loses one half of its kinetic energy. How does the time period of revolution of particle change.
View SolutionA proton and an α- particle is projected with equal kinetic energy in transverse uniform magnetic field B. The ratio of radii of proton to that of alpha particle is
View Solution
