(a) Obtain the expression for the potential due to a point charge.
(b) Use the above expression to show that the potential, due to an electric dipole (length 2a), varies as the inverse square' of the distance r of the 'field point' from the centre of the dipole for $r > a$ .

Video Solution

Text Solution

Verified by Experts

(a) Potential at a point B defined as the amount of work done in bringing a unit positive charge from $\infty$ to that point.

Consider a charge $q_{0}$ to brought from $\infty$ to point P.
Small amount of work done in moving the charge from A to B.
$d w = \vec{F} . \vec{d x} = q_{0} \vec{E} . \vec{d x}$
$= q . E . d x {\cos 180}^{\circ} = - q_{0} E . d x$
Total amount of work done to bring it from $\infty$ to P
$W = - \int q_{0} E d x = - \int_{\infty}^{r} q_{0} \frac{1}{4 π \in_{0}} \frac{q}{x^{2}} d x = - \frac{q q_{0}}{4 π \in_{0}} . \underset{\infty}{\int^{r}} x^{- 2} d x$
$= - \frac{q q_{0}}{4 π \in_{0}} {[- \frac{1}{x}]}_{\infty}^{r} = \frac{q q_{0}}{4 π \in_{0}} [\frac{1}{r} - \frac{1}{\infty}]$
Now, $V = \frac{W}{q_{0}} = \frac{1}{4 π \in_{0}} \frac{q}{r}$ .
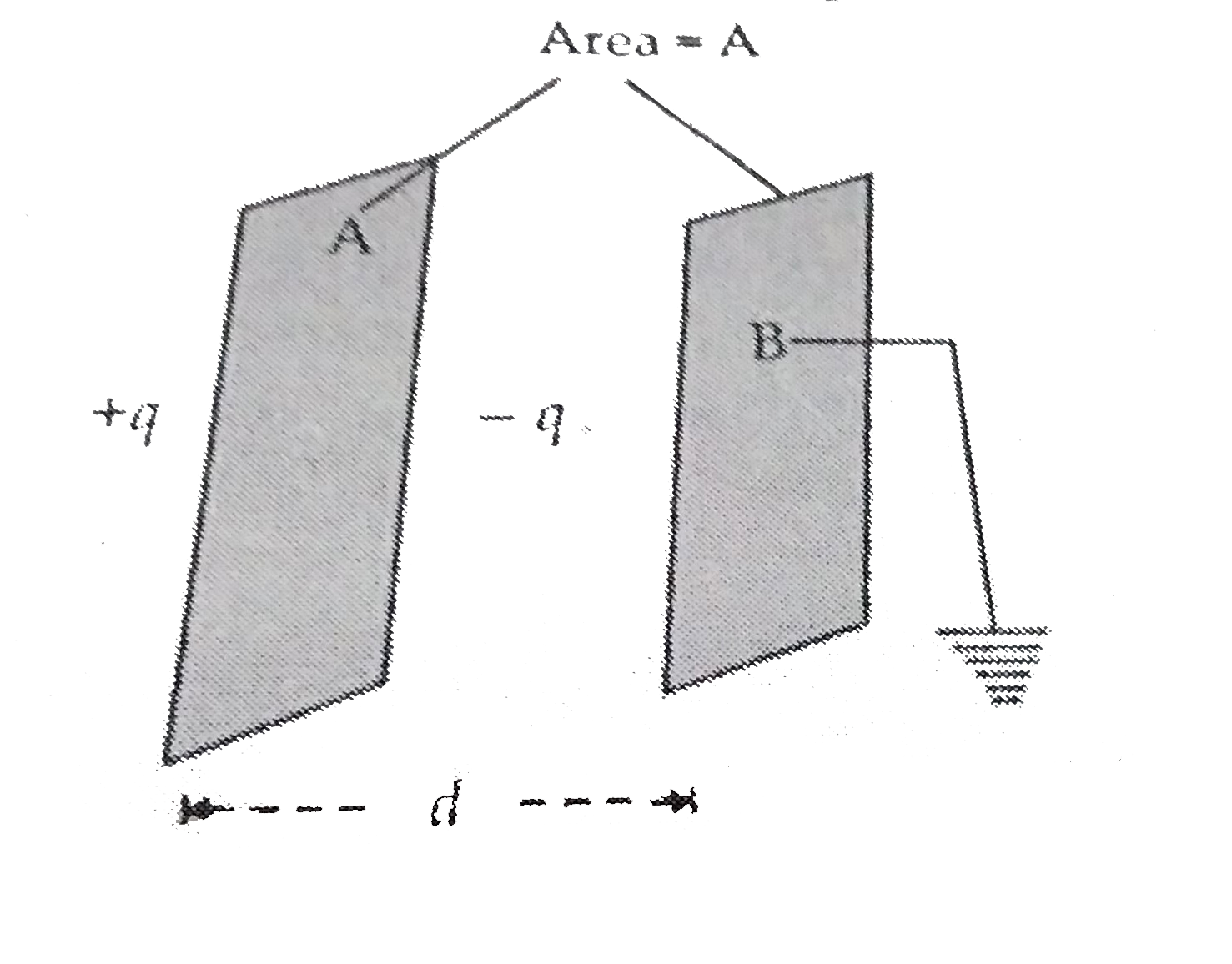
(b) If charge + q is given to plate A, then charge -q is induced on the left face of plate B and charge +q on its right face. When plate B is earthed, the charge + q on its right face flows to earth. Due to charge The electric field between the two plates is related to the potential gradient as
$E = \frac{d V}{d r}$ (in magnitude)
Between the two parallel plates, the electric field is uniform and perpendicular to the plates. Therefore, if V is potential difference between two plates, then
$E = \frac{V}{d} for uniform field (\frac{d V}{d r} = \frac{V}{d})$
or V=Ed
If $σ$ is surface charge density of the plates, of the plates, then the electric field between the two plates is given by
$E = \frac{σ}{ε_{0}}$
where $ε_{0}$ is absolute permittivity of the free space (If it assumed that medium between the plates is carcumm or air).
In the equation, substituting for E, we have
$V = \frac{σ}{ε_{0}} d$
If A is the area of each plate, the
$σ = \frac{q}{A}$
$∴ V = \frac{q}{ε_{0}} \frac{d}{A}$
If C is the capacitance of the parallel plate capacitor, then
$C = \frac{q}{q d / ε_{0} A}$
or $C = \frac{ε_{0} A}{d}$ .

Updated on:21/07/2023