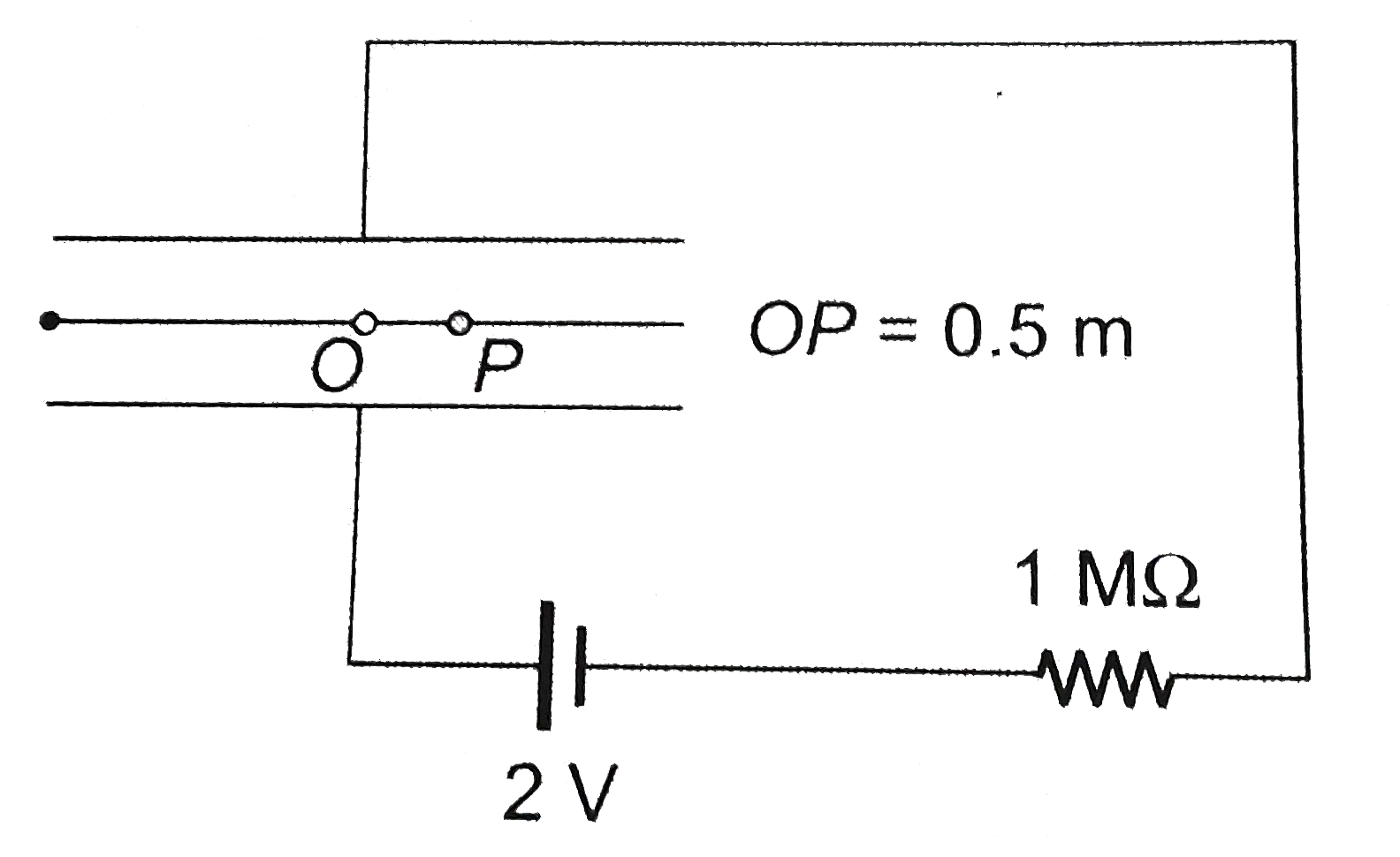
A parallel plate capacitor with circular plates of radius 1 m has a capacitance of 1 nF. ?At t=0, it is connected for charging in series with a resitor R=1M across a 2V battery (Fig. 8.3) calculate the magnetic field at a point P. halfway between the centre and the periphery of the plates after l=s. (The charge on the capacitor at time l is [1-exp(-t/r)]. where the time constant r is equal to CR.)
![A parallel plate capacitor with circular plates of radius 1 m has a capacitance of 1 nF. ?At t=0, it is connected for charging in series with a resitor R=1M Omega across a 2V battery (Fig. 8.3) calculate the magnetic field at a point P. halfway between the centre and the periphery of the plates after l=10^(-3)s. (The charge on the capacitor at time l is q(l)=CV [1-exp(-t/r)]. where the time constant r is equal to CR.)](https://d10lpgp6xz60nq.cloudfront.net/physics_images/NCERT_PHY_V01_XII_C08_S01_001_Q01.png)
The time constant of the CR circuit is .
Then, we have
The electric field in between the plates at time t is = area of the plates.
Consider now a circular loop of radius parallel to the plates passing through P. The magnetic field B at all points on the loop is along the loop and of the same value. Then flux
area of the loop
The displacement current
at . Now, applying Ampere-Maxwell law to the loop, we get
or,
Similar Questions
A parallel plate capacitor with circular plates of radius 1m has a capacitor of 1nF. At t=0, it is connected for charging in series with a resistor R=1MΩ across a 2V battery. Calculate the magnetic field at a point P, halfway between the cnetre and the periphery of the plates, after t=10−3sec.
 View Solution
View SolutionA parallel plate capacitor made of circular plates each of radius 6 cm has a capacitance 100 pF. The capacitor is connected to a 230 V ac supply with a angular frequency of 300 rad/s. The amplitude of magnetic field at point 3 cm from the axis between the plates is
View SolutionA parallel plate capacitor with circular plates of radius R is being charged as shown. At the instant shown, the displacement current in the region between the plates enclosed between R2 and R is given by
 View Solution
View SolutionA parallel plate capacitor has capacitance of 1.0F. If the plates are 1.0mm apart, what is the area of the plates?
View SolutionA parallel plate capacitor has an electric field of 105V/m between the plates. If the charge on the capacitor plate is 1μC, then force on each capacitor plate is-
View SolutionEach plate of a parallel plate capacitor has a charge q on it. The capacitor is now connected to a battery. Now,
View SolutionSuppose a charge +Q1 is given to the positive plates and a charge −Q2 to the negative plate of a capacitor. What is the "charge on the capacitor"?
View SolutionA parallel-plate capacitor with plate are 20cm2and plate separation 1.0mm is connected to a battery .The resistance of the circuit is 10k(Ω).Find the time constant of the circuit.
View SolutionA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
View SolutionThe potentials of the two plates of capacitor are +10V and −10V. The charge on one of the plate is 40C. The capacitance of the capacitor is
View Solution

