A particle A moves with velocity (2ˆi−3ˆj)m/s from a point (4,5m)m. At the same instant a particle B, moving in the same plane with velocity(4ˆi+ˆj)m/s passes through a point C(0,−3)m. Find the x-coordinate (in m) of the point where the particles collide.
The correct Answer is:8
Step by step video, text & image solution for A particle A moves with velocity (2hati-3hatj)m//s from a point (4,5m)m. At the same instant a particle B, moving in the same plane with velocity (4hati+hatj)m//s passes through a point C(0,-3)m. Find the x-coordinate (in m) of the point where the particles collide. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A particle A moves with velocity ( 2 ˆ i − 3 ˆ j ) m / s from a point ( 4 , 5 m ) m . At the same instant a particle B , moving in the same plane with velocity ( 4 ˆ i + ˆ j ) m / s passes through a point C ( 0 , − 3 ) m . Find the x -coordinate (in m ) of the point where the particles collide.
View SolutionA particle of mass m moving with a velocity (3ˆi+2ˆj)ms−1 collides with another body of mass M and finally moves with velocity (−2ˆi+ˆj)ms−1. Then during the collision
View Solution
Knowledge Check
A particle is moving with constant velocity (6ˆi+8ˆj)m/s. It corosses x-axis at point (2,0). The rate of separation from origin at this moment is :
A6ms−1B8ms−1C10ms−1D7ms−1A particle moving with velocity (2ˆi−3ˆj) m/s collides with a surface at rest in xz–plane as shown in figure and moves with velocity (2ˆi+2ˆj) m/s after collision. Then coefficient of restitution is :
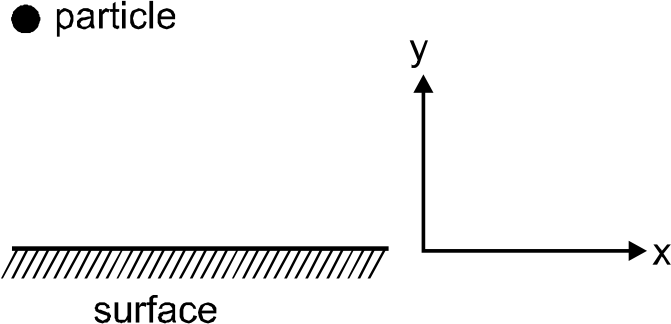 A23B1C√813D45
A23B1C√813D45A particle is projected with initial velocity u=10m/s from a point ( 10m, 0m, 0m ) m along z-axis. At the same time , another particle was also projected with velocity v(ˆi−ˆj+ˆk)m/s from ( 0m, 10m, 0m). It is given that they collide at some point in the space . Then find the value of v. [→g=10m/s2(−ˆk)]
A10B5C5√2D10√2
A particle of mass 2.4kg is having a velocity of (3ˆi+4ˆj)ms at (5,6)m.The angular momentum of the particle about (1,2)m ,in kgm2s ,is?
View SolutionA particle is given an initial velocity of →u=(3ˆi+4ˆj)m/s. Acceleration of the particle is →a=(3t2+2tˆj)m/s2. Find the velocity of particle at t=2s.
View SolutionA particle moves from point (ˆi+ˆj)m to a point (6ˆi−2ˆj)m under the action of force (10ˆi−2ˆj)N. The work done by the force is
View SolutionA particle of mass 1.0 g moving with a velocity →v1=(3ˆi−2ˆj) m/s experiences a perfectly inelastic collision with another particle of mass 2.0 g and velocity →v2=(4ˆi−6ˆj) m/s. Find the magnitude of the velocity vector →v of the coalesced particles.
View SolutionA particle moving with a velocity of (4ˆi−ˆj ) mis strikes a fixed smooth wall and finally moves with a velocity of (3ˆi+2ˆj) m/s. The coefficient of restitution between the wall and the particle in the collision will be
View Solution
