A particle A moves with velocity ( 2 ˆ i − 3 ˆ j ) m / s from a point ( 4 , 5 m ) m . At the same instant a particle B , moving in the same plane with velocity ( 4 ˆ i + ˆ j ) m / s passes through a point C ( 0 , − 3 ) m . Find the x -coordinate (in m ) of the point where the particles collide.
The correct Answer is:8
If particle collide sfter time t at point P, then position of both after time t should be same both have uniform motion.
by S=S0+v×t
for Ist particle SI=4ˆi+5ˆj+(2ˆi−3ˆj)×t
for IInd particle SII=−3ˆj+(4ˆi+ˆj)×t
but SI=SII
4ˆi+5ˆj+(2ˆi−3ˆj)t=−3+(4ˆi+ˆj)+(4ˆi+ˆj)×t
4ˆi+8ˆj=2ˆit+4ˆit
by comrision along ×Δy
t=2 sec
SI=4ˆi+5ˆj+(2ˆi−3ˆj)2
=8ˆi−ˆj
x=8m
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-04[B]
14 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#7
3 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A particle A moves with velocity (2ˆi−3ˆj)m/s from a point (4,5m)m. At the same instant a particle B, moving in the same plane with velocity(4ˆi+ˆj)m/s passes through a point C(0,−3)m. Find the x-coordinate (in m) of the point where the particles collide.
View SolutionA particle moves with a velocity (3i+4j) m/s from origin.The displacement of particle along line x=y after two seconds will be:
View Solution
Knowledge Check
A particle starts moving with constant, velocity v=ms, from position x=5m The position time graph will be
A B
B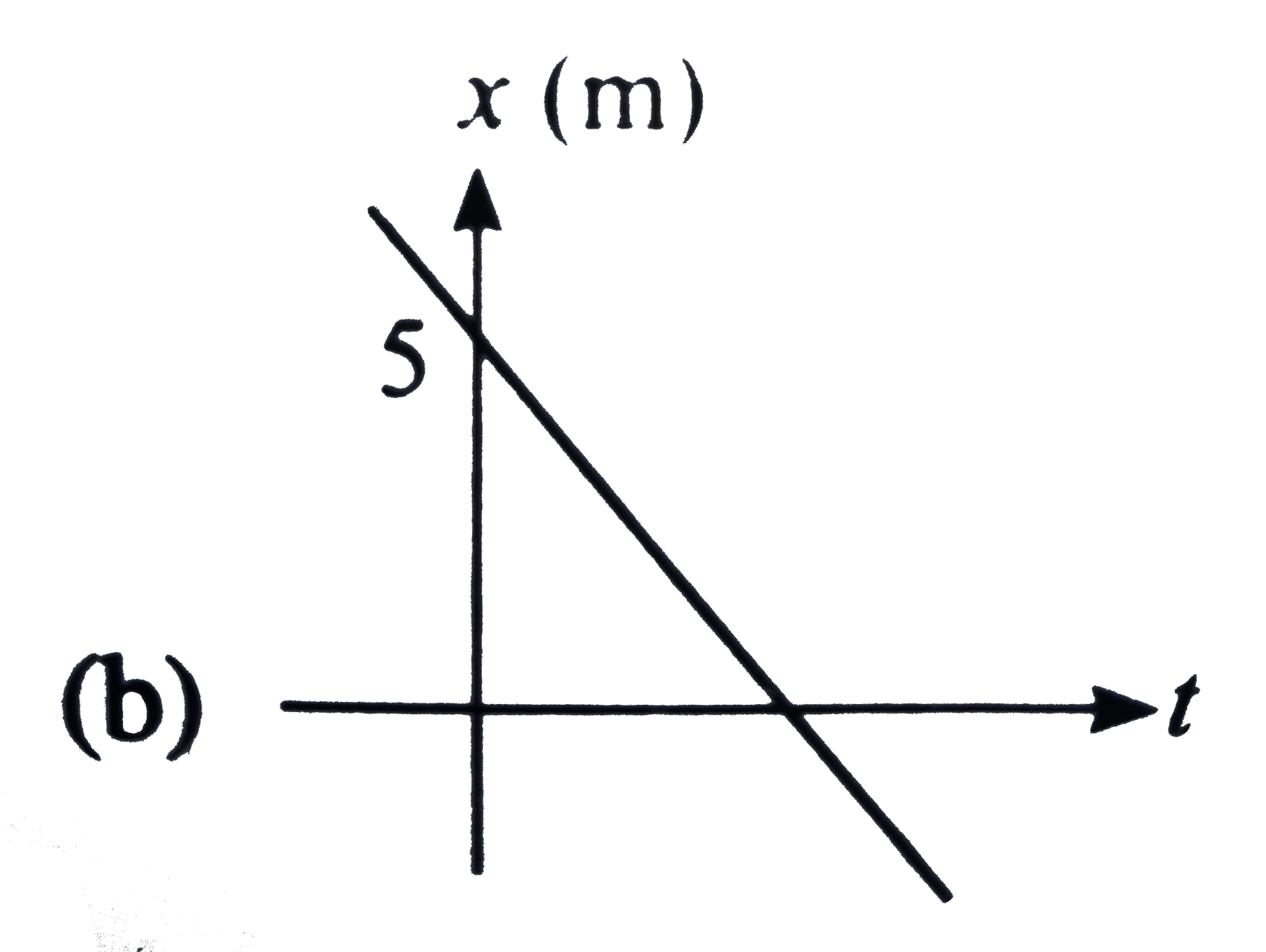 C
C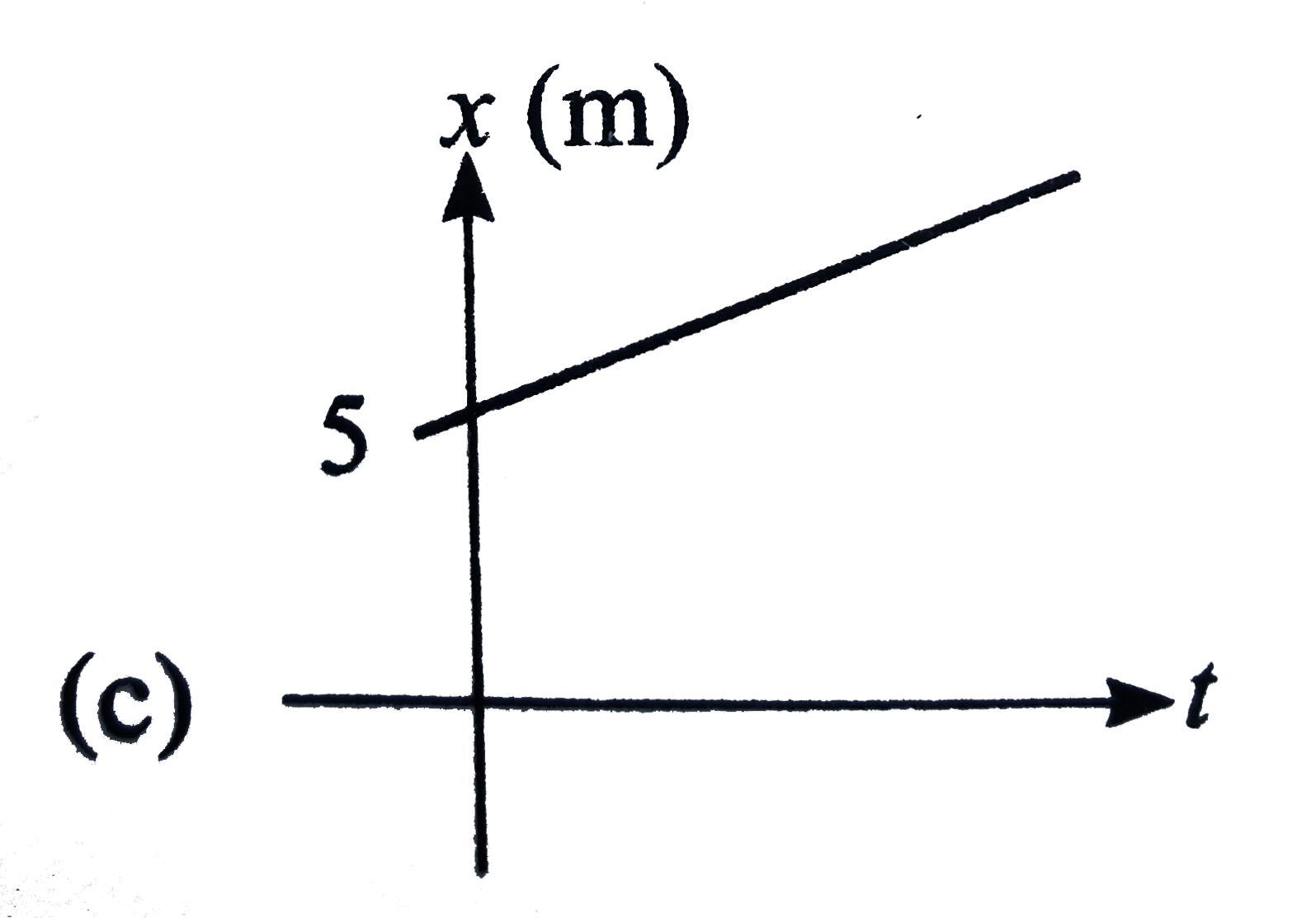 D
D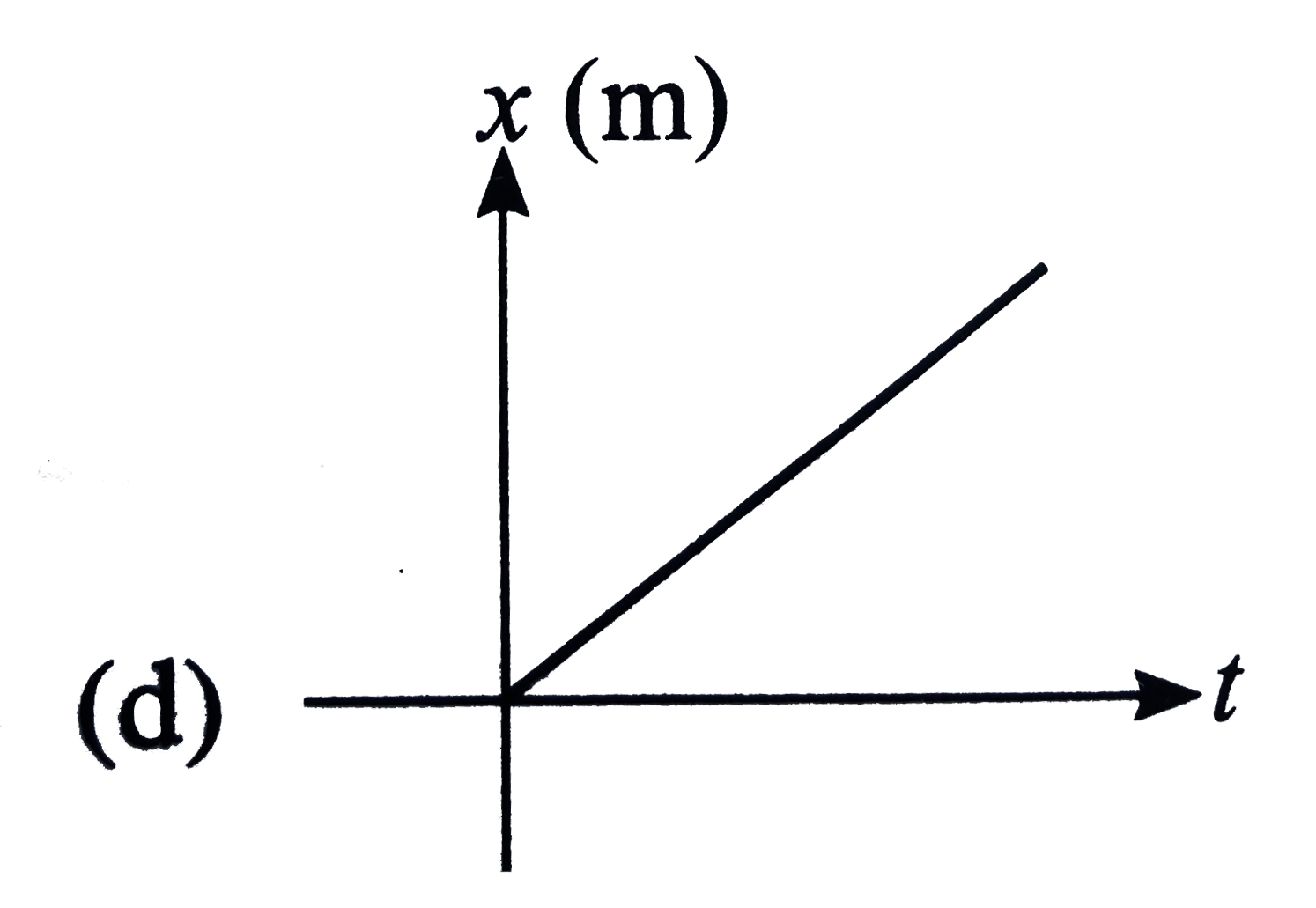
A particle is projected with initial velocity u=10m/s from a point ( 10m, 0m, 0m ) m along z-axis. At the same time , another particle was also projected with velocity v(ˆi−ˆj+ˆk)m/s from ( 0m, 10m, 0m). It is given that they collide at some point in the space . Then find the value of v. [→g=10m/s2(−ˆk)]
A10B5C5√2D10√2A particle moving with velocity (2ˆi−3ˆj) m/s collides with a surface at rest in xz–plane as shown in figure and moves with velocity (2ˆi+2ˆj) m/s after collision. Then coefficient of restitution is :
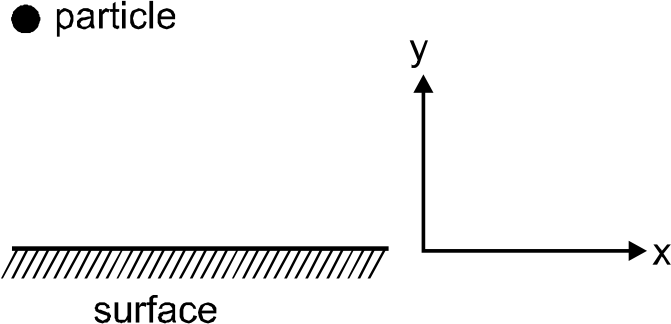 A23B1C√813D45
A23B1C√813D45
A particle of mass m moving with velocity u collides elastically head on with a particle of mass 2m at rest. After collision the heavier particle moves with velocity?
View SolutionFind the kinetic energy of a particle of mass 200g moving with velocity 3ˆi−5ˆj+4ˆkm/s.
View SolutionA particle is moving with an initial velocity of 2m/s and covers a distance of 20m in 5s. Find (a) acceleration of particle (b) time taken by the particle to attain a velocity of 10m/s.
View SolutionThe velocity of a particle at an instant is 10m/s. After 5sec, the velocity of the particle is 20m/s. Find the velocity at 3 seconds before from the instant when velocity of a particle is 10m/s.
View SolutionStarting from origin at t=0, with initial velocity 5ˆjm/s, a particle moves in the x−y plane with a constant acceleration of (10ˆi−5ˆj)m/s2. Find the coordinates of the particle at the moment its y-coordinate is maximum.
View Solution